Question

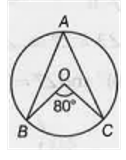
If $\angle BAC={55}^{\circ }$ and the triangle is isosceles, then find the value of $\angle B$

(a) ${72}^{\circ }$

(b) ${62}^{\circ }$

(c) ${60}^{\circ }$

(d) None of these

Options

A. `72^@`
B. `62^@`
C. `60^@`
D. None of these

Explanation

To solve the problem, we need to find the value of angle B in an isosceles triangle where angle BAC is given as 55 degrees.

1. Understand the Properties of the Isosceles Triangle:
- In an isosceles triangle, two sides are equal, and the angles opposite those sides are also equal. Therefore, if we denote angle B and angle C as the equal angles, we can say:
\( \text{angle B} = \text{angle C} \)

2. Use the Triangle Sum Property:
- The sum of the angles in any triangle is always 180 degrees. Therefore, we can write the equation:
\( \text{angle A} + \text{angle B} + \text{angle C} = 180^\circ \)
- Given that angle A (angle BAC) is 55 degrees, we can substitute this into the equation:
\( 55^\circ + \text{angle B} + \text{angle C} = 180^\circ \)

3. Substitute angle C with angle B:
- Since angle B is equal to angle C, we can replace angle C with angle B in the equation:
\( 55^\circ + \text{angle B} + \text{angle B} = 180^\circ \)
- This simplifies to:
\( 55^\circ + 2 \cdot \text{angle B} = 180^\circ \)

4. Isolate angle B:
- Now, we can isolate angle B by first subtracting 55 degrees from both sides:
\( 2 \cdot \text{angle B} = 180^\circ - 55^\circ \)
\( 2 \cdot \text{angle B} = 125^\circ \)

5. Divide by 2:
- Finally, divide both sides by 2 to find the value of angle B:
\( \text{angle B} = \frac{125^\circ}{2} = 62.5^\circ \)

6. Conclusion:
- Since 62.5 degrees is not listed among the options (72 degrees, 62 degrees, 60 degrees, or none of these), the correct answer is:
\( \text{(d) None of these} \)