Question

Halley's comet has a period of 76 years and in 1986, had a distance of closest approach to the sun
equal to ${\displaystyle 8.9\times {10}^{10}m.}$ What is the comet's farthest distance from the sun if the mass of sun is ${\displaystyle 2\times {10}^{30}\text{kg}}$ and
${\displaystyle G=6.67\times {10}^{-11}\text{MKS}}$ units ?

Explanation

From the problem it is self-evident that the orbit of the cometis elliptic with sun being at one focus. Nov
as for elliptic orbits, according to Kepler.s third law,

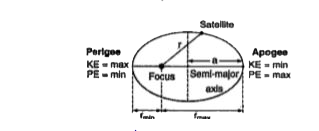
`T^(2)=(4 pi^(2))/("GM")a^(3),` i.e., `a=((T^(2)"GM")/(4 pi^(2)))^(1//3)`
or `a=[((76 xx 3.15 xx 10^(7))^(2)xx 6.67 xx 10^(-11) xx 2 xx 10^(30))/(4 pi^(2))]^(1//3)`
= `2.7 xx 10^(12)m`
But in case of ellipse,
`2a=r_("min")+r_("max"),i.e., r_("max")=2a-r_("min")`
So, `r_("max")=2 xx 2.7 xx 10^(12)-8.9 xx 10^(19)= 5.3 xx 10^(12)m`