If a body is immersed in a fluid, it experiences an upward force called the buoyant force. The magnitude of buoyant force depends on (a) the size or volume of the body and (b) the density of the fluid. How large is this buoyant force? The answer to this question was discovered by the Greek Philosopher and mathematician Archimedes (287-212B.C) and is known as Archimedes Principle which states that ‘When a body is immersed wholly or partly in a fluid, it experiences an upthrust equal to the weight of the fluid displaced by it'. This principle holds for bodies of any shape and for fluids of even non-uniform density. The necessary condition for a body to float in a liquid is that the weight of the liquid displaced by the immersed portion of the body must be equal to the weight of the body.
A cubical-block of density $ρ$ floats completely immersed in two non-mixing liquids of densities $ρ_{1} and ρ_{2}$ as shown in Fig. The relation between $ρ, ρ_{1} and ρ_{2}$ is

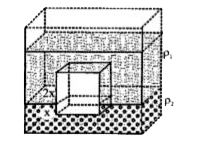
$ρ = \frac{1}{2} (ρ_{1} + ρ_{2})$

$ρ = 2 ρ_{1} + ρ_{2}$

$ρ = \frac{1}{3} (2 ρ_{1} + ρ_{2})$

$ρ = \frac{1}{3} (ρ_{1} + 2 ρ_{2})$

Text Solution

Verified by Experts

The correct Answer is:C

Updated on:21/07/2023