Two communicating cyliridrical tubes contain mercury. The diameter of one is two times that of the other. A column of water of height 70 cm is poured into the narrow vessel. How much will the mercury level in centimeters sink in this vessel? (Take relative density of water as 1 and that of mercury as 14)
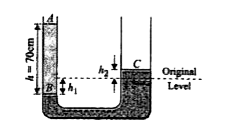
The correct Answer is:4
Since the diameter of wider vessel is twice, its areas is four times and hence, the rise in mercury level is one fourth.
⇒h1=4h2
Now, PB−PA=ρwgh andPB−PC=ρwg(h1+h2)
Since, PA=PC = atmospheric pressure, we have
ρwgh=ρm(h1_h14) ∴h1=4h5ρwρm=45×70x114=4cm
Similar Questions
Two communicating cylindrical tubes contain mercury. The diameter of one vessel is four times large than the diameter of the outer. A column of water of height 70cm is poured into the narrow vessel. How much wil the mercury level rise in the other vessel and how much will it sink in the narow one? How much will the mercury level rise in the narrow vessel, if a column of water of the same height is pured into the broad vessel?
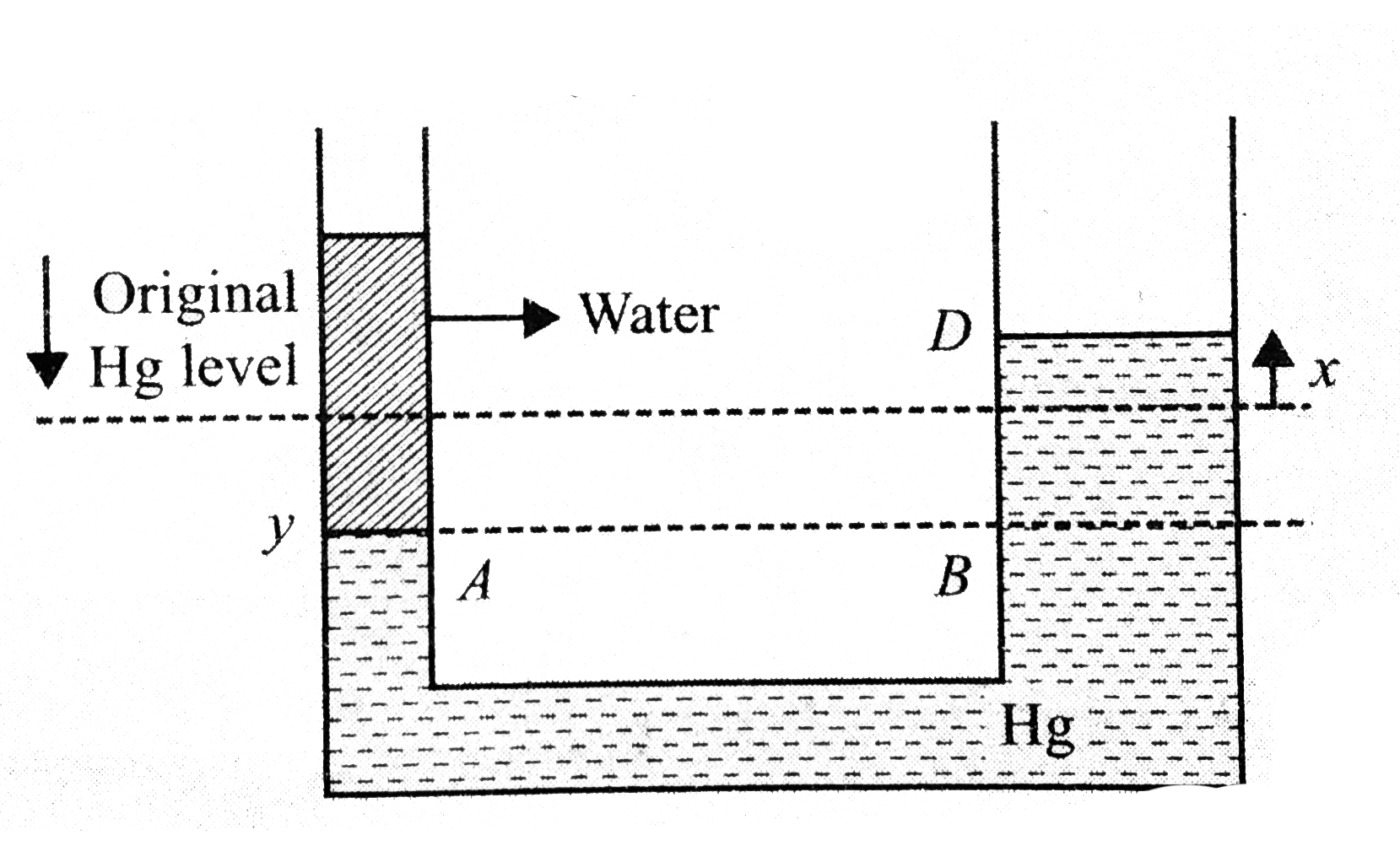
View SolutionTwo communicating cylindrical tubes contain mercury. The diameter of one vessel is four times large than the diameter of the outer. A column of water of height 70cm is poured into the narrow vessel. How much will the mercury level rise in the other vessel and how much will it sink in the narrow one? How much will the mercury level rise in the narrow vessel, if a column of water of the same height is poured into the broad vessel?

View Solution
Knowledge Check
Two communicating vessels contain mercury. The diameter of one vessel in n times larger then the diameter of the other. A column of water of height h is poured into the left vessel. The mercury level will rise in the right hand vessel (s = relative density of mercury and ρ = density of water) by
 An2h(n+1)2sBh(n2+1)sCh(n+1)2sDhn2s
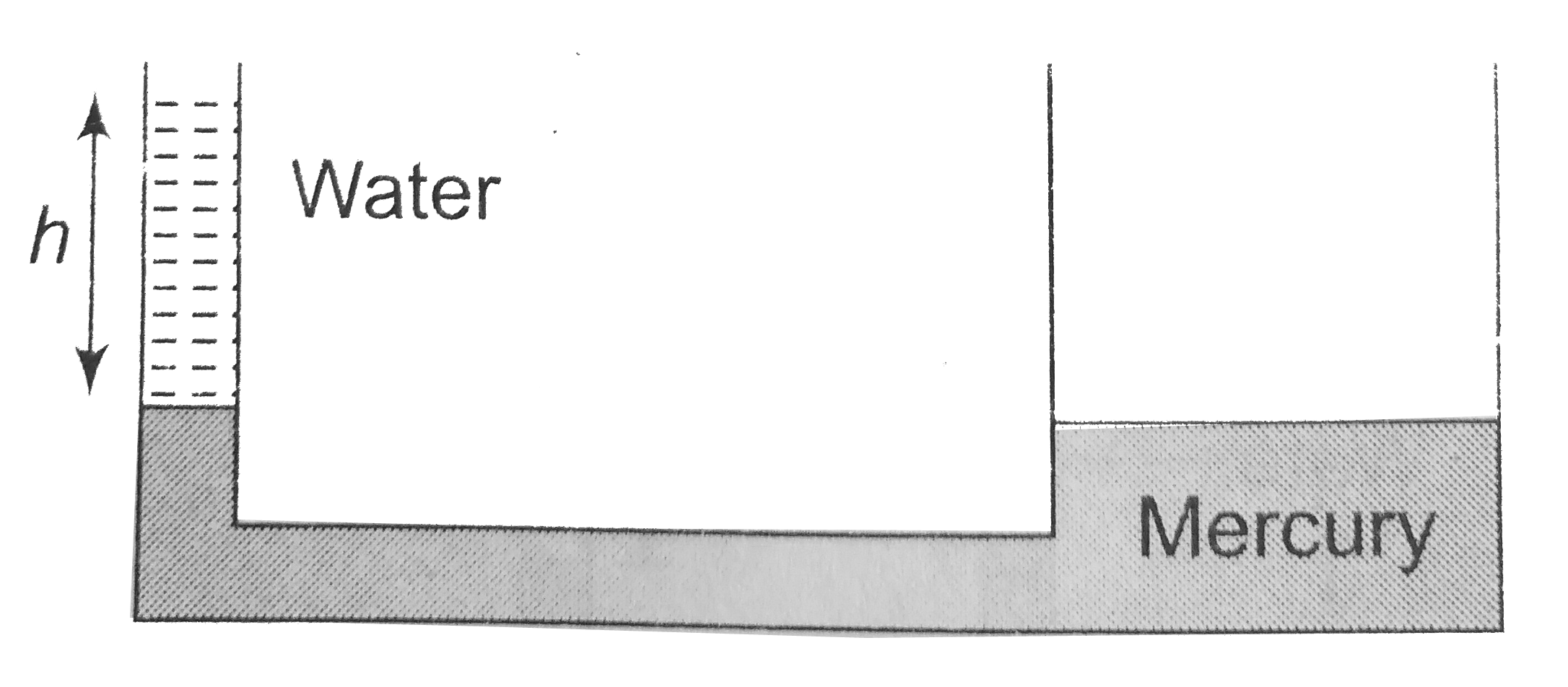
An2h(n+1)2sBh(n2+1)sCh(n+1)2sDhn2sAn open U-tube contains mercury. When 11.2 cm of water is poured into one of the arms of the tube, how high does the mercury rise in the other arm from its initial level ?
A0.82 cmB1.35 cmC0.41 cmD2.32 cmin previous question, if 15 cm of water and spirit each are further poured into the respective arms of the tube. Difference in the level of mercury in the two arms is (Take, relvative density of mercury = 13.6)
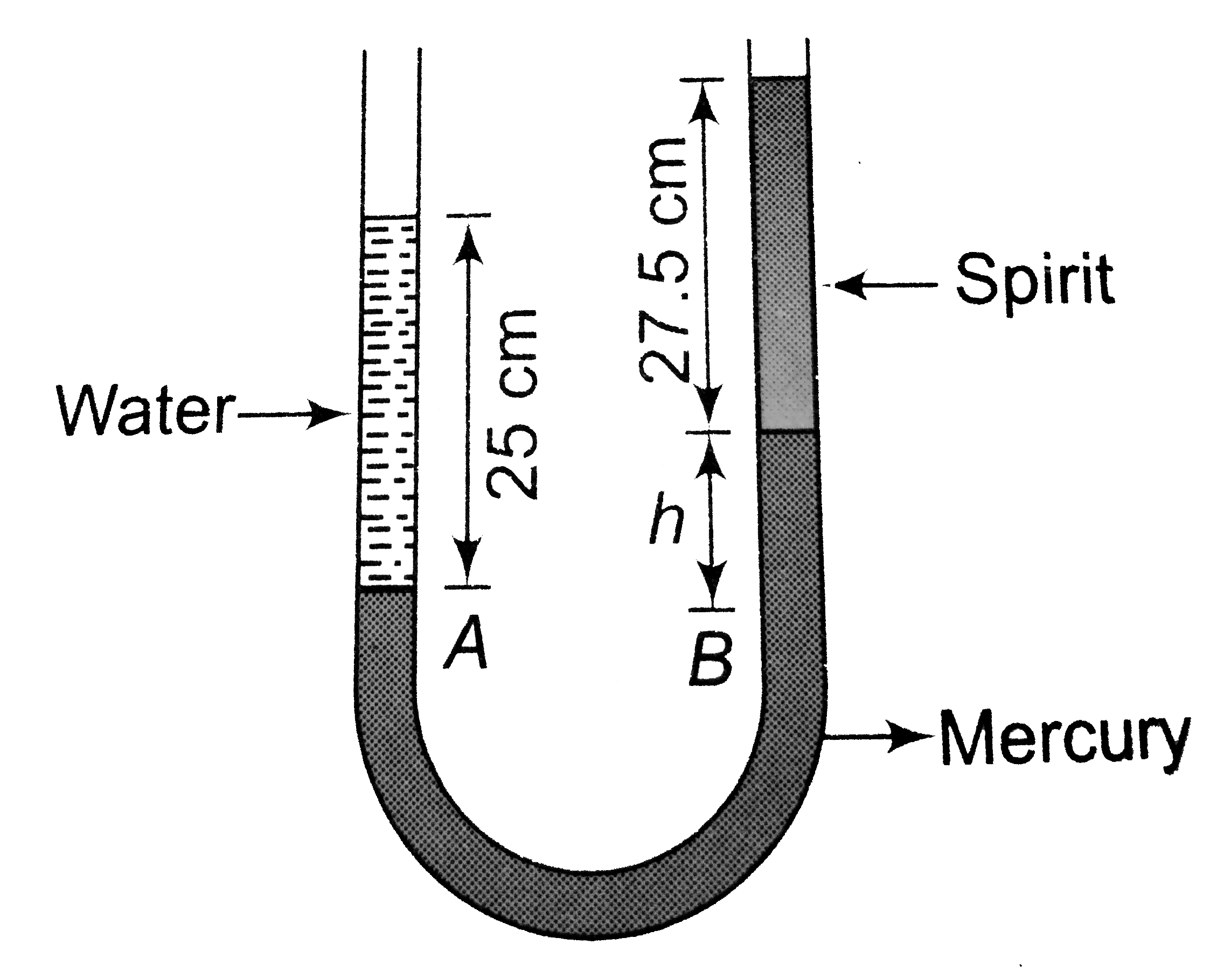 A0.20 cmB0.22 cmC0.27c mD0.26cm
A0.20 cmB0.22 cmC0.27c mD0.26cm
Two communicating vessels contain mercury. The diameter of one vessel in n times larger then the diameter of the other. A column of water of height h is poured into the left vessel. The mercury level will rise in the right hand vessel (s = relative density of mercury and ρ = density of water) by

View SolutionA simple U tube contains mercury to the same level in both of its arms. If water is poured to a height of 13.6 cm in one arm, how much will be the rise in mercury level in the other arm ?
Given : density of mercury = 13.6×103kg m−3 and density of water = 103kg m−3.
View SolutionAn open U-tube contains mercury. When 11.2 cm of water is poured into one of the arms of the tube, how high does the mercury rise in the other arm from its initial level ?
View Solution(i) A cubical metal block of side 10cm is floating in a vessel contatining mercury. The vesel has a square cross section of side length 15cm. Water is poured into the vessel so that the metal block just gets submerged. Calculate the mass of water that was poured into the vessel. It is given that relative density of the metal and mercury are 7.3 and 13.6 respectively.
(ii) In the last question, in place of water if we poured another liquid of relative density 'r' it was found that when the metal block ws just completely submerged it was no longer touching mercury. what is value of r?
View SolutionIf 15.0 cm of water and spirit each are further poured into the respective arms of the tube, what is the difference in the levels of mercury in the two arms? (Relative density of mercury = 13.6).
View Solution
