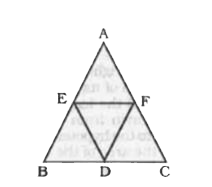
Three straight lines are drawn through the three vertices of a triangle ABC, the line through each vertex being parallel to the opposite side. The triangle DEF is bounded by these parallel lines. Consider the following statements in respect of the triangle DEF :
1. Each side of triangle DEF is double the side of triangle ABC to which it is parallel.
2. Area of triangle DEF is four times the area of triangle ABC.
Which of the above statements is/are correct?
1 only
2 only
Both 1 and 2
Neither 1 nor 2
To solve the problem, we will analyze the statements regarding triangle DEF formed by drawing lines through the vertices of triangle ABC that are parallel to the opposite sides.
1. Understanding the Configuration:
- Let triangle ABC have vertices A, B, and C.
- Lines are drawn through points A, B, and C that are parallel to the opposite sides BC, AC, and AB, respectively.
- The points where these lines intersect form triangle DEF.
2. Analyzing the Sides:
- Since the lines through the vertices are parallel to the opposite sides, triangle DEF is similar to triangle ABC.
- The sides of triangle DEF will be proportional to the sides of triangle ABC.
- Specifically, if the sides of triangle ABC are denoted as a, b, and c, then the corresponding sides of triangle DEF (which are parallel) will be 2a, 2b, and 2c, respectively.
- This confirms that each side of triangle DEF is double the corresponding side of triangle ABC.
3. Analyzing the Area:
- The area of similar triangles is proportional to the square of the ratio of their corresponding sides.
- Since the sides of triangle DEF are double those of triangle ABC, the ratio of the sides is 2:1.
- Therefore, the ratio of the areas will be (2:1)² = 4:1.
- This means the area of triangle DEF is 4 times the area of triangle ABC.
4. Conclusion:
- Both statements are correct:
1. Each side of triangle DEF is double the side of triangle ABC to which it is parallel.
2. The area of triangle DEF is four times the area of triangle ABC.
Final Answer:
Both statements are correct.
- Let triangle ABC have vertices A, B, and C.
- Lines are drawn through points A, B, and C that are parallel to the opposite sides BC, AC, and AB, respectively.
- The points where these lines intersect form triangle DEF.
- Since the lines through the vertices are parallel to the opposite sides, triangle DEF is similar to triangle ABC.
- The sides of triangle DEF will be proportional to the sides of triangle ABC.
- Specifically, if the sides of triangle ABC are denoted as a, b, and c, then the corresponding sides of triangle DEF (which are parallel) will be 2a, 2b, and 2c, respectively.
- This confirms that each side of triangle DEF is double the corresponding side of triangle ABC.
- The area of similar triangles is proportional to the square of the ratio of their corresponding sides.
- Since the sides of triangle DEF are double those of triangle ABC, the ratio of the sides is 2:1.
- Therefore, the ratio of the areas will be (2:1)² = 4:1.
- This means the area of triangle DEF is 4 times the area of triangle ABC.
- Both statements are correct:
1. Each side of triangle DEF is double the side of triangle ABC to which it is parallel.
2. The area of triangle DEF is four times the area of triangle ABC.
Both statements are correct.
Similar Questions
The perimeter of a triangle is 22 cm. Through each vertex of the triangle, a straight line parallel to the opposite side is drawn. What is the perimeter of triangle formed by these lines ?
View SolutionThree straight lines are drawn through a point P lying inside a triangle ABC, parallel to its sides. The area of the resulting triangles are 1,4 and 9 sq units. Find the area of ΔABC in square units.
View Solution
Knowledge Check
If D, E and F are the mid-points of sides BC, CA and AB respectively of △ABC, then the ratio of the areas of △DEF to the area of △ABC is :
A1:4B1:2C1:3D2:3If △ABC≅△DEF then
AAC=DEBBC=DFCFE=CBDAB=DFThrough each vertex of a triangle, a line parallel to the opposite side is drawn. The ratio of the perimeter of the new triangle, thus formed, with that of the original triangle is
A3:2B4:1C2:1D2:3
If D, E and F are the mid-points of the sides of an equilateral triangle ABC, then the ratio of the area of triangle DEF and DCF is :
View SolutionSides the three line segments ABBC and CA that form the triangle ABC are called the sides of the triangle ABC.
View SolutionEach side of triangle ABC is divided into three equal parts. Find the ratio of the are of hexagon PQRSTU to the area of the triangle ABC.
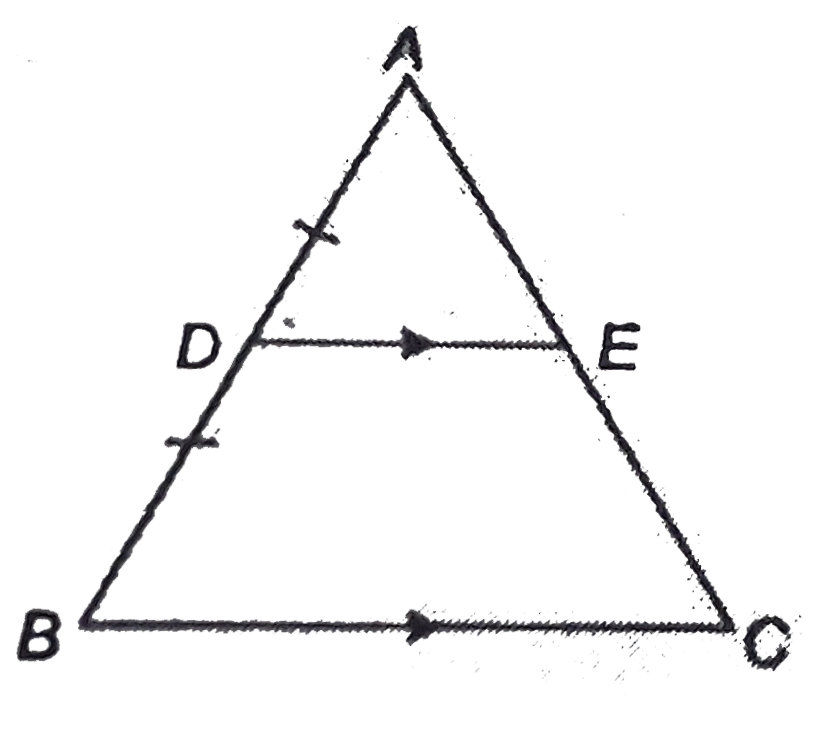
View SolutionProve using similar triangles, that a line drawn through the mid-point of one side of a triangle parallel to another side, bisects the third side.
View SolutionIn △ABC and △ DEF, AB = DE, BC = EF and ∠B=∠E. If the perimeter of △ ABC is 20, then the perimeter of △ DEF is ..........
View Solution
