Question

The chord of an arc of a circle is of length x, the height of the arc is y and the radius of the circle is z. Which one of the following is correct?

Options

A. `y(2z-y)=x^(2)`
B. `y(2z-y)=4x^(2)`
C. `2y(2z-y) =x^(2)`
D. `4y(2z-y) =x^(2)`

Explanation

To solve the problem, we need to establish the relationship between the chord length $$x$$, the height of the arc $$y$$, and the radius of the circle $$z$$.

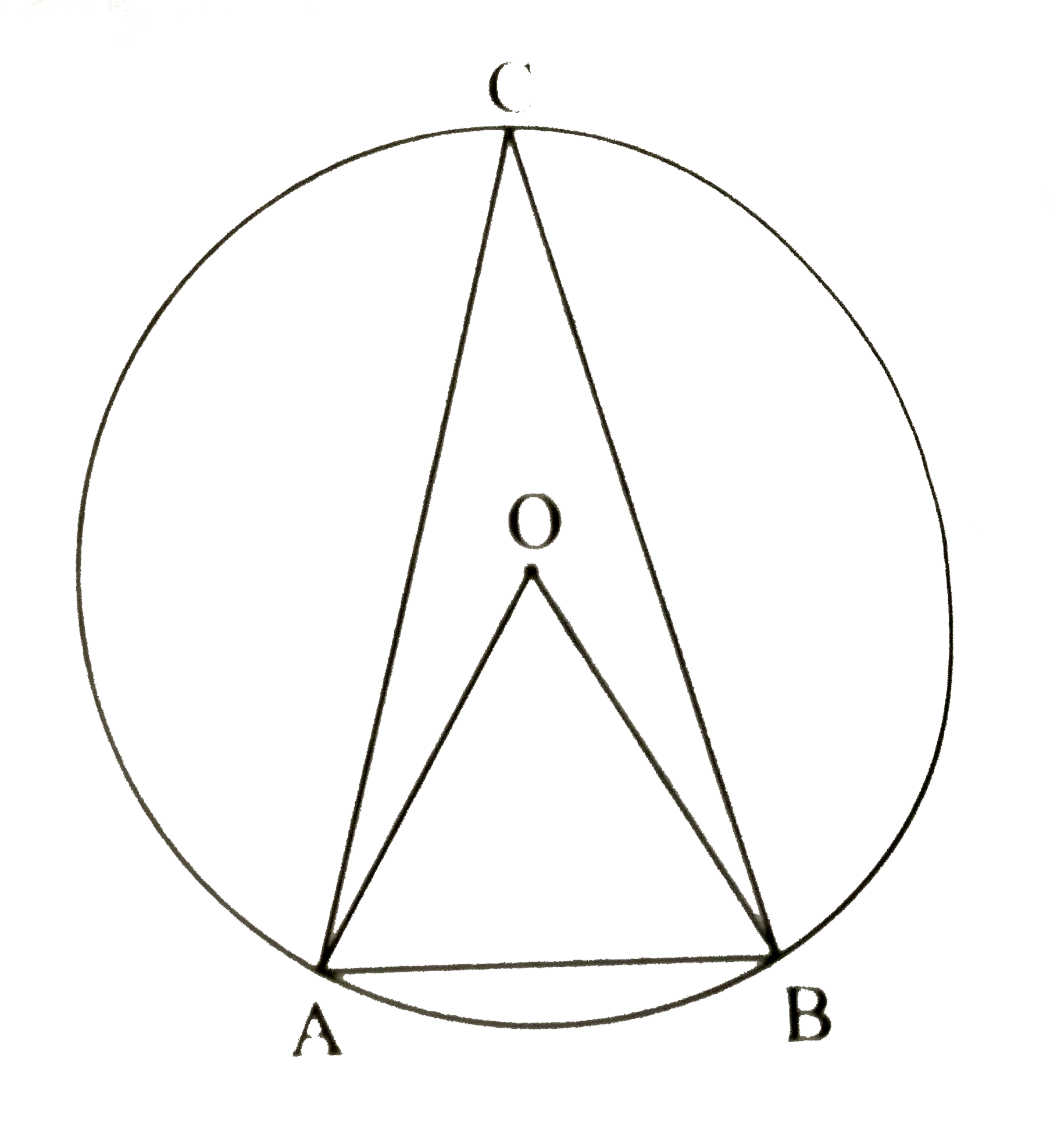
1. Identify the Geometry:
- Let \( AC \) be the chord of the circle with length \( x \).
- Let \( O \) be the center of the circle, and \( D \) be the midpoint of the chord \( AC \).
- The radius of the circle is \( z \), and the height of the arc from the chord to the circle's circumference is \( y \).

2. Relationship Between Chord and Radius:
- The perpendicular from the center \( O \) to the chord \( AC \) bisects the chord. Therefore, \( AD = \frac{x}{2} \).

3. Using the Pythagorean Theorem:
- In the right triangle \( OAD \):
- \( OA = z \) (radius)
- \( AD = \frac{x}{2} \) (half of the chord)
- \( OD = z - y \) (the distance from the center to the chord is the radius minus the height of the arc)
- By the Pythagorean theorem, we have:
\( OA^2 = AD^2 + OD^2 \)
Substituting the values:
\( z^2 = \left(\frac{x}{2}\right)^2 + (z - y)^2 \)

4. Expanding the Equation:
- Expanding the right side:
\( z^2 = \frac{x^2}{4} + (z^2 - 2zy + y^2) \)
- Simplifying gives:
\( z^2 = \frac{x^2}{4} + z^2 - 2zy + y^2 \)

5. Isolating Terms:
- Subtract \( z^2 \) from both sides:
\( 0 = \frac{x^2}{4} - 2zy + y^2 \)

6. Rearranging the Equation:
- Rearranging gives:
\( 2zy - y^2 = \frac{x^2}{4} \)
- Multiplying through by 4 to eliminate the fraction:
\( 8zy - 4y^2 = x^2 \)

7. Final Relationship:
- Thus, we arrive at the relationship:
\( 4y(2z - y) = x^2 \)

Conclusion:
The correct relationship among the chord length \( x \), the height of the arc \( y \), and the radius \( z \) is given by:
\( 4y(2z - y) = x^2 \)