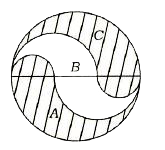
A circle of 3 m radius is divided into three areas by semicircles of radii 1 m and 2 m as shown in the figure above. The ratio of the three areas A, B and C will be
2:3:2
1:1:1
4:3:4
1:2:1
Step by step video & image solution for A circle of 3 m radius is divided into three areas by semicircles of radii 1 m and 2 m as shown in the figure above. The ratio of the three areas A, B and C will be by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Similar Questions
Knowledge Check
- Question 1 - Select One
If the sum of the areas of two circles with radii R1 and R2 is equal to the area of a circle of radius R, then
AR1+R2=RBR21+R22=R2CR1+R2<RDR21+R22<R2 - Question 2 - Select One
Three circle of equal radii touch each other as shown in figure . The radius of each circle is 1 cm. What is the area of shaded region ?
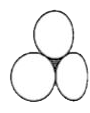 A(2√3−π2)cm2B(3√2−π3)cm2C2√3πcm2Dnone of the above
A(2√3−π2)cm2B(3√2−π3)cm2C2√3πcm2Dnone of the above - Question 3 - Select One
A figure consists of a square of side 'a' m with semicircle drawn on the outside of the square. The area ( in m2) of the figure so formed will be :
Aa2(π+1)Ba2(π+14)Ca2+πa22Dnone of these
Find the areas of the circles whose radii are (a) 14m (b) 28cm
View SolutionThe area of the equilateral triangle, in which three coins of radius 1 cm are placed, as shown in the figure, is :
 View Solution
View SolutionIf a region bounded by a circle C is to be divided into three regions of equal areas by drawing into circles concentric with C , then the ratio of the radii of the two circles must be (a) 1 : 3 (b) 1 :√3 (c) 1 : 2 (d) 1 :√2
View SolutionThe area of the equilateral triangle, in which three coins of radius 1cm are placed, as shown in the figure, is
View SolutionThree circles of radii r1,r2andr3 are drawn concentric to each other. The radii r1 and r2 are such that the area of the circle with radius r1 is equal to the area between the circle of radius r2 and r1 . The area between the circle of radii r2 and r3 is equal to area between the circle of radii r2 and rr . What is the value of r1:r2:r3 ?
View Solution

