By the Principle of Mathematical Induction, prove the following for all n ∈ N :
The nth term of an A.P. whose first term is 'a’ and common difference ‘d' is a + (n-1) d.Solution in Punjabi
Step by step video & image solution for By the Principle of Mathematical Induction, prove the following for all n in N : The nth term of an A.P. whose first term is 'a’ and common difference ‘d' is a + (n-1) d. by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MATHEMATICAL INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - MATHEMATICAL INDUCTIONEXERCISE - EXERCISE
85 Videos
- LINEAR INEQUATIONSBOOK - MODERN PUBLICATIONCHAPTER - LINEAR INEQUATIONSEXERCISE - EXERCISE
289 Videos
- MATHEMATICAL MODELLINGBOOK - MODERN PUBLICATIONCHAPTER - MATHEMATICAL MODELLINGEXERCISE - EXAMPLE
3 Videos
Similar Questions
Let P (n) be the statement : “P(n) : 10n + 3 is prime’’. Is P(3) true ?
View SolutionSolve the following inequation :
3x−9<0.
View SolutionThe angle of elevation of the top of a tower from a point O, on the ground, which is 600 m away from the foot of the tower, is 45∘. Obtain the height of the tower.
View SolutionTheorem:-Let a be the first term and d be the common difference of an AP. Then its nth term and general term is given by an=a+(n−1)d
View SolutionTheorem: The sum of nth terms of an AP with first term a and common difference d is Sn=n2(2a+(n−1)d)
View SolutionIn an A.P., the first term is 22, nth term is −11 and the sum to first n terms is 66. Find n and d , the common difference.
View Solutionl, m, n are three numbers in G.P., a is the first term of an A.P., whose l th, mth and nth terms are in H.P. If d is the common difference of the A.P.. prove that a = (m + 1)d.
View SolutionFor all n∈N, prove by principle of mathematical induction that,
a+(a+d)+(a+2d)+... to n terms =n2[2a+(n−1)d].
View SolutionThe first term and common difference of an A.P. are a and d respectively. If mth and nth terms of this A.P. are 1mand1n respectively, then the value of (a−d) is-
View SolutionA sequence is called an A.P if the difference of a term and the previous term is always same i.e if an+1−an= constant ( common difference ) for all n∈N
For an A.P whose first term is 'a ' and common difference is d is
Sn=n2(2a+(n−1)d)=n2(a+a+(n−1)d)=n2(a+l)
1. A sequence whose nth term is given by tn=An+N , where A,B are constants , is an A.P with common difference
A. 2
B. 1
C. A
D. B
If sum of n terms Sn for a sequence is given by Sn=An2+Bn+C, then sequence is an A.P. whose common difference is
A. A
B. B
C. 2A
D. 2B
View SolutionA sequence is called an A.P if the difference of a term and the previous term is always same i.e if an+1−an= constant ( common difference ) for all n∈N
For an A.P whose first term is 'a ' and common difference is d has nth term as tn=a+(n−1)d
Sum of n terms of an A.P. whose first term is a, last term is l and common difference is d is
Sn=n2(2a+(n−1)d)=n2(a+a+(n−1)d)=n2(a+l)
If sum of n terms Sn for a sequence is given by Sn=An2+Bn+C, then sequence is an A.P. whose common difference is
View SolutionA sequence is called an A.P if the difference of a term and the previous term is always same i.e if an+1−an= constant ( common difference ) for all n∈N
For an A.P whose first term is 'a ' and common difference is d has nth term as tn=a+(n−1)d
Sum of n terms of an A.P. whose first term is a, last term is l and common difference is d is
Sn=n2(2a+(n−1)d)=n2(a+a+(n−1)d)=n2(a+l)
a1,a2,a3,....a8 is an A.P. with common difference d. Then a8−2k−ak(1≤k<4k∈N) is equal to
View Solutionnth term of an A.P with first term a and common difference d
View SolutionThe nth term of an A.P. whose first term is 'a' and common difference d' is a+(n−1)d. Prove by PMI.
View SolutionBy Principle of Mathematical Induction :
nth term of A.P. whose first term is 'a' and common difference is 'd' is ______
View SolutionFill in the blanks in the following table, given that a is the first term, d the common difference and an the nth term of the A.P.
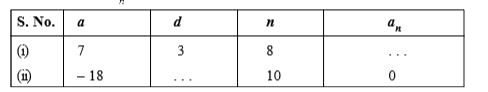

View Solution
