Initial fuel of rocket = 1000kg if it was given acceleration = 20 m/s^2 then find rate of consumption of fuel if velocity of fuel w.r.t rocket = 500 m/s
40
60
80
NONE
To solve the problem step by step, we will use the principles of rocket motion and the concept of thrust.
1. Identify Given Values:
- Initial mass of the rocket (m) = 1000 kg
- Acceleration of the rocket (A) = 20 m/s²
- Velocity of fuel with respect to the rocket (v) = 500 m/s
- Acceleration due to gravity (g) = 10 m/s² (assuming standard value)
2. Understand the Forces Acting on the Rocket:
- The rocket experiences an upward force due to the thrust generated by burning fuel.
- The effective acceleration of the rocket is the sum of the rocket's acceleration and the acceleration due to gravity acting in the opposite direction.
3. Calculate the Relative Acceleration:
- The relative acceleration (A_relative) can be expressed as:
Arelative=A+g=20m/s2+10m/s2=30m/s2
4. Apply the Rocket Equation:
- According to the thrust equation, the thrust (F) generated by the rocket can be expressed as:
F=v⋅dmdt
- Here, dmdt is the rate of consumption of fuel.
5. Relate Thrust to Mass and Acceleration:
- The thrust can also be related to the mass and the relative acceleration:
F=m⋅Arelative=1000kg⋅30m/s2=30000N
6. Set the Thrust Equations Equal:
- Equating the two expressions for thrust, we have:
v⋅dmdt=30000N
7. Solve for Rate of Consumption of Fuel:
- Rearranging gives:
dmdt=30000N500m/s=60kg/s
8. Conclusion:
- The rate of consumption of fuel is dmdt=60kg/s.
Final Answer:
The rate of consumption of fuel is 60 kg/s.
---
- Initial mass of the rocket (m) = 1000 kg
- Acceleration of the rocket (A) = 20 m/s²
- Velocity of fuel with respect to the rocket (v) = 500 m/s
- Acceleration due to gravity (g) = 10 m/s² (assuming standard value)
- The rocket experiences an upward force due to the thrust generated by burning fuel.
- The effective acceleration of the rocket is the sum of the rocket's acceleration and the acceleration due to gravity acting in the opposite direction.
- The relative acceleration (A_relative) can be expressed as:
Arelative=A+g=20m/s2+10m/s2=30m/s2
- According to the thrust equation, the thrust (F) generated by the rocket can be expressed as:
F=v⋅dmdt
- Here, dmdt is the rate of consumption of fuel.
- The thrust can also be related to the mass and the relative acceleration:
F=m⋅Arelative=1000kg⋅30m/s2=30000N
- Equating the two expressions for thrust, we have:
v⋅dmdt=30000N
- Rearranging gives:
dmdt=30000N500m/s=60kg/s
- The rate of consumption of fuel is dmdt=60kg/s.
The rate of consumption of fuel is 60 kg/s.
Topper's Solved these Questions
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-A
80 Videos
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-B
40 Videos
- JEE MAINBOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINEXERCISE - All Questions
473 Videos
- JEE MAIN 2022BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2022EXERCISE - Question
492 Videos
Similar Questions
(a) A rocket set for vertical firing weighs 50kg and contains 450kg of fuel. It can have a maximum exhaust velocity of 2km/s. What should be its minimum rate of fuel consumption
(i) to just lift off the launching pad?
(ii) to give it an initial acceleration of 20m/s2?
(b) What will be the speed of the rocket when the rate of consumption of fuel is 10kg/s after whole of the fuel is consumed? (Take g=9.8m/s2)
View SolutionA rocket of mass 20kg has 180kg fuel. The exhaust velocity of the fuel is 1.6km/s. Calculate the minimum rate of consumption of fuel so that the rocket may rise from the ground. Also, calculate the ultimate vertical speed gained by the rocket when the rate of consumption of fuel is (g=9.8m/s2)
(i) 2kg/s (ii) 20kg/s
View Solution
Knowledge Check
The force on a rocket moving with a veloctiy 300 m/s is 210N. The rate of consumption of fuel of rocket is
A0.7 kg/sB1.4 kg/sC0.07 kg/sD10.7 kg/sIf the force on a rocket moving moving with a velocity of 300 m/s is 345 N, then the rate of combustion of the fuel is
A0.55 kg/sB0.75 kg/sC1.15 kg/sD2.25 kg/sIf the force on a rocket moving with a velocity of 300m/s is 345 N, then the rate of combustion of the fuel is
A2.25kg/sB0.75kg/sC1.15 kg/sD0.55kg/s
If rocket engine ejects fuel at the rate μ kg/s with relative velocity vr. At time t = 0, speed of rocket is u. Consider the value of acceleration due to gravity is constant and equal to g.
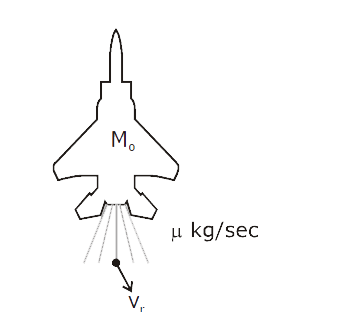
Find velocity of rocket as function of time.
View SolutionA rocket is fired vertically up from the ground with an acceleration 10 m/s2. If its fuel is finished after 1 minute then calculate -
(a) Maximum velocity attained by rocket in ascending motion.
(b) Height attained by rocket fuel is finished.
(c ) Time taken by the rocket in the whole motion.
(d) Maximum height attained by rocket.
View SolutionA rocket of mass 40kg has 160kg fuel. The exhaust velocity of the fuel is 2.0km/s. The rate of consumption of fuel is 4kg/s. Calculate the ultimate vertical speed gained by the rocket. (g=10m/s2)
View SolutionDuring the rocket propulsion, gases ejecting with velocity 1 km/s relative to rocket. The rate of fuel consumption is m10 kg/s, where m is the instantaneous mass of the rocket. If air ressitance varies according to equation f=0.15 mv, then terminal velocity of the rocket is -
View SolutionFor a Rocket propulsion velocity of exhaust gases relative to rocket is 2km/s. If mass of rocket system is 1000 kg, then the rate of fuel consumption for a rockt to rise up with acceleration 4.9m/s2 will be:-
View Solution
