A particle of mass 2M is divided in four particles of mass m, m, m-M, M-m. All particles are arrange on vertex of square of side a. Find ration of M/m for which potential energy of this system is maximum/minimum
2
44228
3
44256
To solve the problem of finding the ratio Mm for which the potential energy of the system is maximum or minimum, we can follow these steps:
Step 1: Understand the System
We have a mass 2M divided into four particles with masses m,m,M−m,M−m arranged at the vertices of a square of side a.
Step 2: Calculate the Potential Energy
The gravitational potential energy U between two masses m1 and m2 separated by a distance r is given by:
U=−Gm1m2r
For our system, we need to consider the potential energy contributions from all pairs of masses.
Step 3: Identify the Distances
- The distance between adjacent vertices of the square is a.
- The distance between opposite vertices (diagonal) is √2a.
Step 4: Write the Total Potential Energy
The total potential energy U of the system can be expressed as the sum of the potential energies of all unique pairs of masses:
U=−G(m⋅ma+m⋅(M−m)a+m⋅(M−m)a+(M−m)⋅(M−m)a+m⋅m√2a+m⋅(M−m)√2a+(M−m)⋅(M−m)√2a)
Step 5: Simplify the Expression
Combine the terms to simplify U:
U=−G(2m2+2m(M−m)+(M−m)2a+2m2+2m(M−m)+(M−m)2√2a)
This can be simplified further to express U in terms of m and M.
Step 6: Differentiate to Find Extrema
To find the values of M/m that maximize or minimize U, we differentiate U with respect to m and set the derivative equal to zero:
dUdm=0
Step 7: Solve the Derivative Equation
After differentiating and simplifying, we will obtain a relation involving M and m. Solving this will yield the ratio Mm.
Step 8: Conclusion
Upon solving, we find that the ratio Mm=2 gives us the condition for maximum or minimum potential energy.
Final Answer
Thus, the ratio Mm for which the potential energy of this system is maximum or minimum is:
Mm=2
We have a mass 2M divided into four particles with masses m,m,M−m,M−m arranged at the vertices of a square of side a.
The gravitational potential energy U between two masses m1 and m2 separated by a distance r is given by:
U=−Gm1m2r
For our system, we need to consider the potential energy contributions from all pairs of masses.
- The distance between adjacent vertices of the square is a.
- The distance between opposite vertices (diagonal) is √2a.
The total potential energy U of the system can be expressed as the sum of the potential energies of all unique pairs of masses:
U=−G(m⋅ma+m⋅(M−m)a+m⋅(M−m)a+(M−m)⋅(M−m)a+m⋅m√2a+m⋅(M−m)√2a+(M−m)⋅(M−m)√2a)
Combine the terms to simplify U:
U=−G(2m2+2m(M−m)+(M−m)2a+2m2+2m(M−m)+(M−m)2√2a)
This can be simplified further to express U in terms of m and M.
To find the values of M/m that maximize or minimize U, we differentiate U with respect to m and set the derivative equal to zero:
dUdm=0
After differentiating and simplifying, we will obtain a relation involving M and m. Solving this will yield the ratio Mm.
Upon solving, we find that the ratio Mm=2 gives us the condition for maximum or minimum potential energy.
Thus, the ratio Mm for which the potential energy of this system is maximum or minimum is:
Mm=2
Topper's Solved these Questions
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-A80videos
JEE MAIN 2021
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-B40videos
JEE MAIN 2021
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINEXERCISE - All Questions473videos
JEE MAIN
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2022EXERCISE - Question492videos
JEE MAIN 2022
Similar Questions
Knowledge Check
- Question 1 - Select One
Three particles each of mass m are palced at the corners of an equilateral triangle of side b. The gravitational potential energy of the system of particle is
A−3Gm22bB−Gm22bC−3Gm2bD−Gm2b - Question 2 - Select One
Four particles each of mass m are placed at the vertices of a square of side l. the potential at the centre of square is
A−2√2GmlB−3√2GmlC−2GmlD−4√2Gml
The four particles of masses m,3m,2m and 4m are placed on the vertices of a sqare of side L. Locate the center of mass
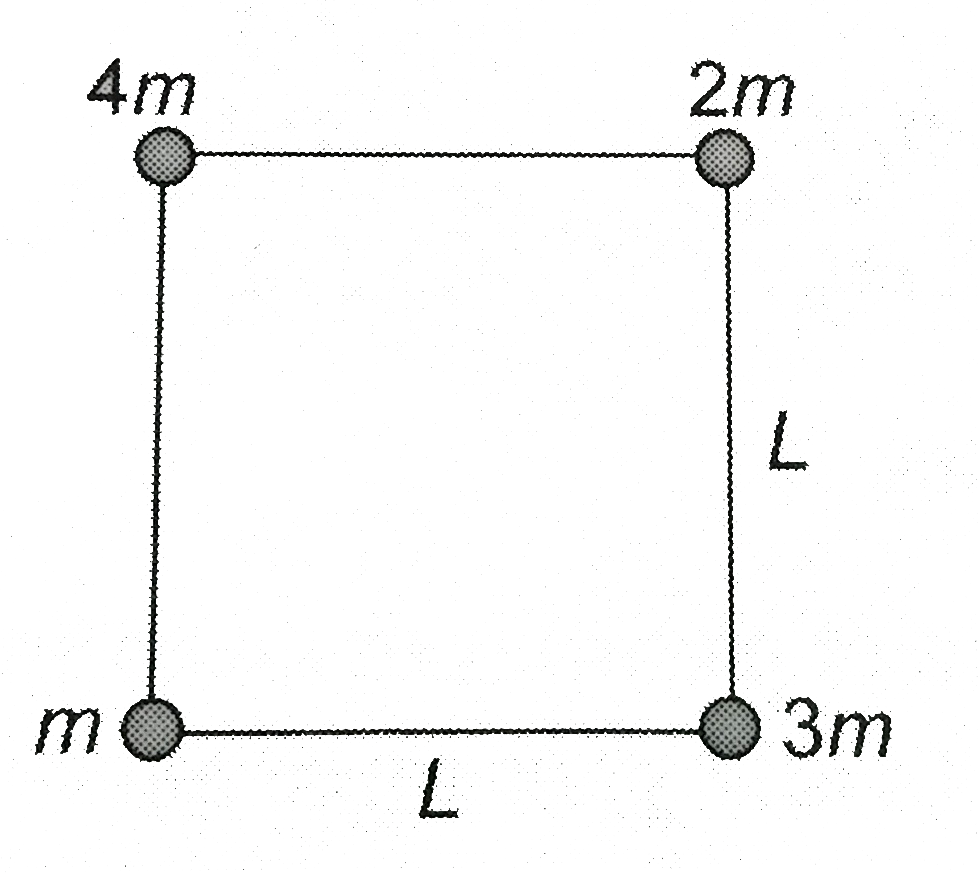 View Solution
View SolutionEight particles of mass 'm' each are placed at the verties of a cube of side 'a' . Find gravitational potential energy of this system .
 . View Solution
. View SolutionA body of mass (2M) splits into four masses {m,M−m,m,M−m}, which are rearranged to form a square as shown in the figure. The ratio of Mm for which, the gravitational potential energy of the system becomes maximum is x : 1 . The value of x is ________.
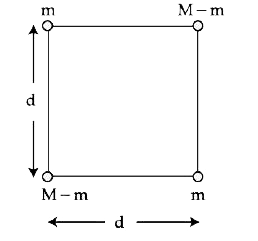 View Solution
View SolutionFour particles of masses m, m, 2m and 2m are placed at the corners of a square of side a, as shown in fig. Locate the centre of mass.
View SolutionFour particles of masses m,2m,3m,4m are placed at corners of a square of side 'a' as shown in fig. Find out coordinateds of centre of mass.
 View Solution
View SolutionFour particles A,B,CandD of masses m,2m,3mand4m respectively are placed at corners of a square of side x as shown in Fig.
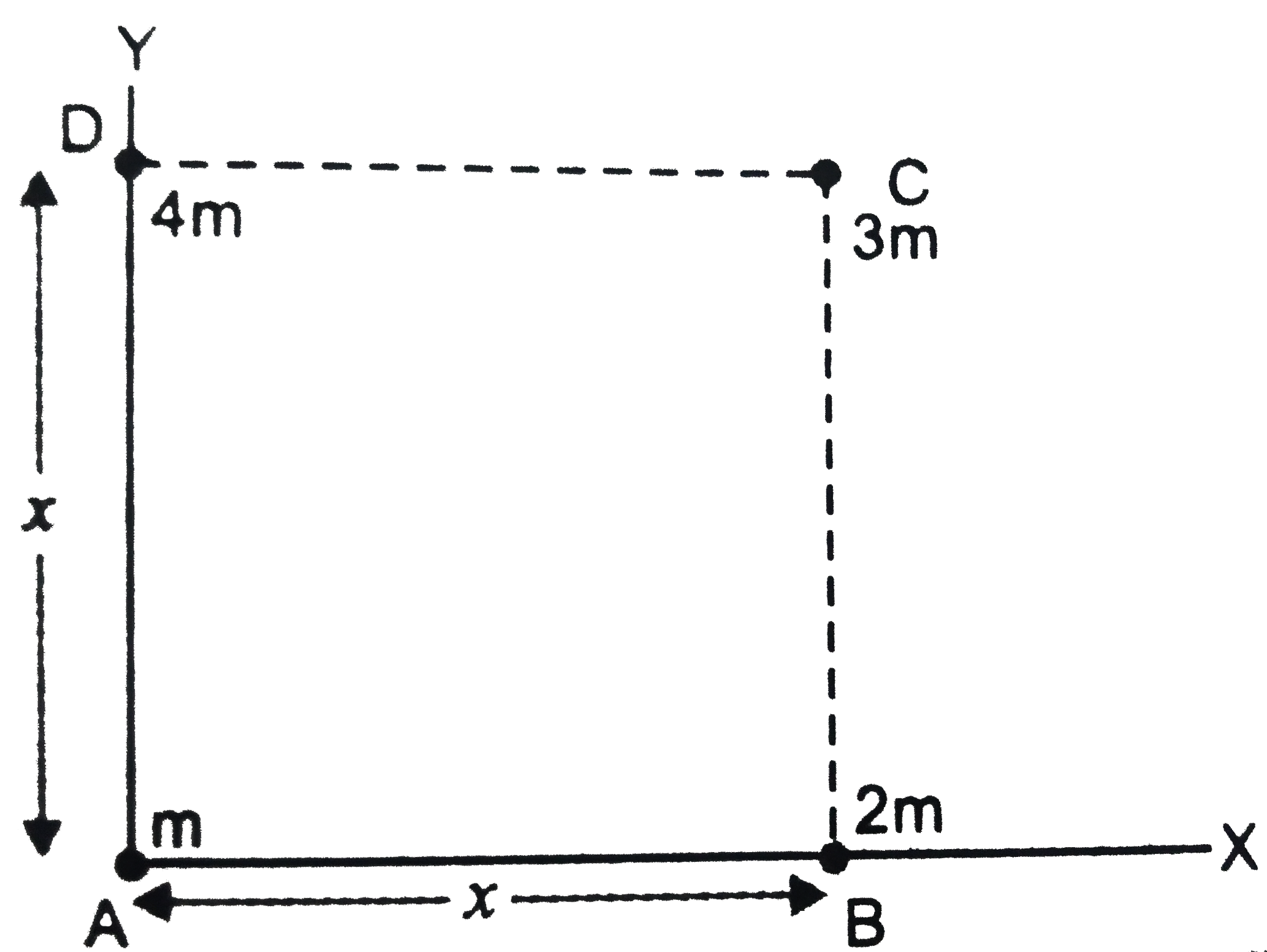
Locate the centre of mass.View Solution

