How many words can be formed by taking 3 consonants and 2 vowels out of $ consonants and -4 vowels
A
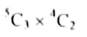
B
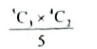
C
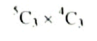
D
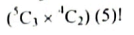
Text Solution
The correct Answer is:D
|
Similar Questions
Knowledge Check
How many words can be formed by taking 3 consonants and 2 vowels out of 5 consonants and 4 vowels?
A5C3×4C2B5C3×4C25C5C3×4C3D5C3×4C2(5)!Find the number of different words that can be formed from 15 consonants and 5 vowels by taking 2 consonants and 4 vowels in each word.
A625B378000C525D615Number of different words that can be formed from 15 consonants and 5 vowels by taking 2 consonants and 4 vowels in each word is
A15P2×5C4B15C2×5C4×6!C15P2D15P2×5P4×2!×4!
Find the number of different words that can be formed from 12 consonants and 5 vowels by taking 4 consonants and 3 vowels in each words.
View SolutionHow many words can be formed out of 10 consonants and 4 vowels, such that each contains 3 consonants and 2 vowels?
View SolutionOut of 12 consonants and 5 vowels, how many words of 2 consonants and 3 vowels can be formed?
View SolutionFind the number of different words that can be formed from 15 consonants and 5 vowels by taking 2 consonants and 4 vowels in each word.
View SolutionHow many words of 4 consonants and 3 vowels is can be made from 12 consonants and 4 vowels . If all the letters are different?
View Solution
