The average marks of section A are 65 and that of section B are 70. If th average marks of both the sections combined are 67, then the ratio of the number of studnts of section A to that of section B is
A)3:2
B)1:3
C)3:1
D)2:3
To solve the problem, we need to find the ratio of the number of students in section A to the number of students in section B given their average marks.
Step 1: Define Variables
Let the number of students in section A be nA and the number of students in section B be nB.
Step 2: Use the Average Formula
The average marks of section A is given as 65, and for section B, it is 70. The combined average for both sections is 67.
The formula for average is:
Average=Total MarksNumber of Students
Thus, we can express the total marks for each section:
- Total marks for section A = 65×nA
- Total marks for section B = 70×nB
Step 3: Set Up the Combined Average Equation
The combined average can be expressed as:
(65×nA)+(70×nB)nA+nB=67
Step 4: Cross Multiply to Eliminate the Fraction
Cross multiplying gives:
(65×nA)+(70×nB)=67(nA+nB)
Step 5: Expand the Equation
Expanding the right side:
65nA+70nB=67nA+67nB
Step 6: Rearrange the Equation
Rearranging the equation to isolate terms involving nA and nB:
65nA+70nB−67nA−67nB=0
−2nA+3nB=0
Step 7: Solve for the Ratio
Rearranging gives:
3nB=2nA
Thus, the ratio of nA to nB is:
nAnB=32
Step 8: Conclusion
The ratio of the number of students in section A to section B is 3:2.
Let the number of students in section A be nA and the number of students in section B be nB.
The average marks of section A is given as 65, and for section B, it is 70. The combined average for both sections is 67.
Average=Total MarksNumber of Students
- Total marks for section A = 65×nA
- Total marks for section B = 70×nB
The combined average can be expressed as:
(65×nA)+(70×nB)nA+nB=67
Cross multiplying gives:
(65×nA)+(70×nB)=67(nA+nB)
Expanding the right side:
65nA+70nB=67nA+67nB
Rearranging the equation to isolate terms involving nA and nB:
65nA+70nB−67nA−67nB=0
−2nA+3nB=0
Rearranging gives:
3nB=2nA
Thus, the ratio of nA to nB is:
nAnB=32
The ratio of the number of students in section A to section B is 3:2.
Similar Questions
If the average marks of 30 students of section A is 55 and the average marks of 30 students of section B is 60 ,then find the combined average of both the sections.
View SolutionIf the average marks of 30 students of section A is 55 and the average marks of 30 students of section B is 60 ,then find the combined average of both the sections.
View Solution
Knowledge Check
The average mathematics marks of two Sections A and B of Class IX in the annual examination is 74. The average marks of Section A is 77.5 and that of Section B is 70. The ratio of the number of students of Section A and B is
A7:8B7:5C8:7D8:5The average mathematics marks of two Sections A and B of Class IX in the annual examination is 74. The average marks of Section A is 77.5 and that of Section B is 70. The ratio of the number of students of Section A and B is:
A7:8B7:5C8:7D8:5The average marks obtained by all the students of class A and class B is in a examination were 68.4 and 71.2 respectively .If the combined average of the marks obtained by the students of both the classes is 70 , then the ratio of the number of students of class A anc B is :
A15:6B3:7C4:3D3:4
Out of two sections A and B, 10 students of section B shift to A, as a result strength of A becomes 3 times the strength of B. But, if 10 students shift over from A to B, both A and B become equal in strength. Ratio of the number of students in section A that of section B is
View SolutionThe average score of two serctions is 28. The average score of section A is 30, which there are 25 students, If there are 20 students in section B,then the average score of section B is
View SolutionThe pie chart given below shows the number of students in 5 sections .The number of students is 7200.
The number of students in section A is what percent of that of section B?
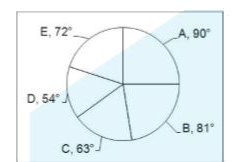
View SolutionRead the data given below and answer the questions based on it.
There were 3 sections namely A, B and C in a test. Out of three 33 students passed in section A, 34 students passed in Section B and 32 passed in Section C. 10 students passed in Section A and Section B, 9 passed in Section B and Section C, 8 passed in Section A and Section C. The number of students who passed each section alone was equal and was 21 for each section.
The ratio of the number of students passed in one or more of the sections to the number of students who passed in section A alone is :
A. 78/21
B. 3
C. 73/21
D. 75/21
View SolutionThere were five sections in MAT paper. The average score of Pooja in first 3 sections was 83 and the average in the last 3 sections was 96 and the average of all the sections (i.e., whole paper) was 92, then her score in the third section was :
View Solution
