A particle is moving in a horizontal circular motion of radius R with constant speed v, then the angular momentum of particle about the axis passing through centre is....
constant
magnitude is varying
direction is varying
both magnitude and direction are varying
To find the angular momentum of a particle moving in a horizontal circular motion of radius R with constant speed v, we can follow these steps:
Step 1: Understand the Definition of Angular Momentum
Angular momentum L of a particle about a point is defined as:
L=r×p
where r is the position vector from the point of rotation to the particle, and p is the linear momentum of the particle.
Step 2: Express Linear Momentum
The linear momentum p of the particle can be expressed as:
p=mv
where m is the mass of the particle and v is its constant speed.
Step 3: Determine the Position Vector
In circular motion, the position vector r can be represented as:
r=R^r
where R is the radius of the circle and ^r is the unit vector pointing from the center to the particle.
Step 4: Calculate the Angular Momentum
Using the formula for angular momentum:
L=r×p=(R^r)×(mv)
This simplifies to:
L=mRv(^r×^v)
Since in uniform circular motion, the position vector ^r is always perpendicular to the velocity vector ^v, we have:
^r×^v=1(since sin(90∘)=1)
Thus,
L=mRv
Step 5: Analyze the Magnitude and Direction
- Magnitude: The magnitude of angular momentum L=mRv is constant because m, R, and v are all constant.
- Direction: The direction of angular momentum is given by the right-hand rule. If you curl the fingers of your right hand in the direction of the particle's motion, your thumb will point upwards, indicating that the direction of angular momentum is constant and perpendicular to the plane of motion.
Conclusion
The angular momentum of the particle about the axis passing through the center is constant in both magnitude and direction.
Final Answer
The angular momentum of the particle about the axis passing through the center is constant.
---
Angular momentum L of a particle about a point is defined as:
L=r×p
where r is the position vector from the point of rotation to the particle, and p is the linear momentum of the particle.
The linear momentum p of the particle can be expressed as:
p=mv
where m is the mass of the particle and v is its constant speed.
In circular motion, the position vector r can be represented as:
r=R^r
where R is the radius of the circle and ^r is the unit vector pointing from the center to the particle.
Using the formula for angular momentum:
L=r×p=(R^r)×(mv)
This simplifies to:
L=mRv(^r×^v)
Since in uniform circular motion, the position vector ^r is always perpendicular to the velocity vector ^v, we have:
^r×^v=1(since sin(90∘)=1)
Thus,
L=mRv
- Magnitude: The magnitude of angular momentum L=mRv is constant because m, R, and v are all constant.
- Direction: The direction of angular momentum is given by the right-hand rule. If you curl the fingers of your right hand in the direction of the particle's motion, your thumb will point upwards, indicating that the direction of angular momentum is constant and perpendicular to the plane of motion.
The angular momentum of the particle about the axis passing through the center is constant in both magnitude and direction.
The angular momentum of the particle about the axis passing through the center is constant.
Topper's Solved these Questions
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-A
80 Videos
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-B
40 Videos
- JEE MAINBOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINEXERCISE - All Questions
473 Videos
- JEE MAIN 2022BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2022EXERCISE - Question
492 Videos
Similar Questions
A particle is revolving in a circular path of radius 25m with constant angular speed 12 rev/min.Then the angular acceleration of particle is
View SolutionA particle is revolving in a circular path of radius 2 m with constant angular speed 4 rad/s. The angular acceleration of particle is
View Solution
Knowledge Check
the circular motion of a particle with constant speed is
ASimple harmonic but not periodicBPeriodic and simple harmonicCneither periodic nor simple harmonicDperiodic but not simple harmonicthe circular motion of a particle with constant speed is
ASimple harmonic but not periodicBPeriodic an simple harmonicCNeither periodic nor simple harmonicDPeriodic but not simple harmonicA particle is revoiving in a circular path of radius 25 m with constant angular speed 12 rev/min. then the angular acceleration of particle is
A2π2 rad/s2B4π2rad/s2Cπ2 rad/s2Dzero
A particle is revolving in a circular path of radius 25m with constant angular speed 12 rev/min.Then the angular acceleration of the particle is?
View SolutionA particle of mass 1kg is moving about a circle of radius 1m with a speed of 1m/s. Calculate the angular momentum of the particle.
View Solution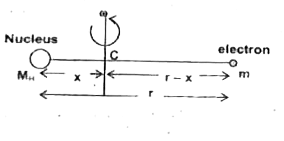
The angular momentum of the atom will be constant about an axis passing through
View SolutionA particle is moving on a circular path of radius R with constant speed v. During motion of the particle form point A to point B

View SolutionA particle of mass m is describing a circular path of radius r with uniform speed. If L is the angular momentum of the particle (about the axis of the circle), then the kinetic energy of the particle is given by
View Solution
