Question

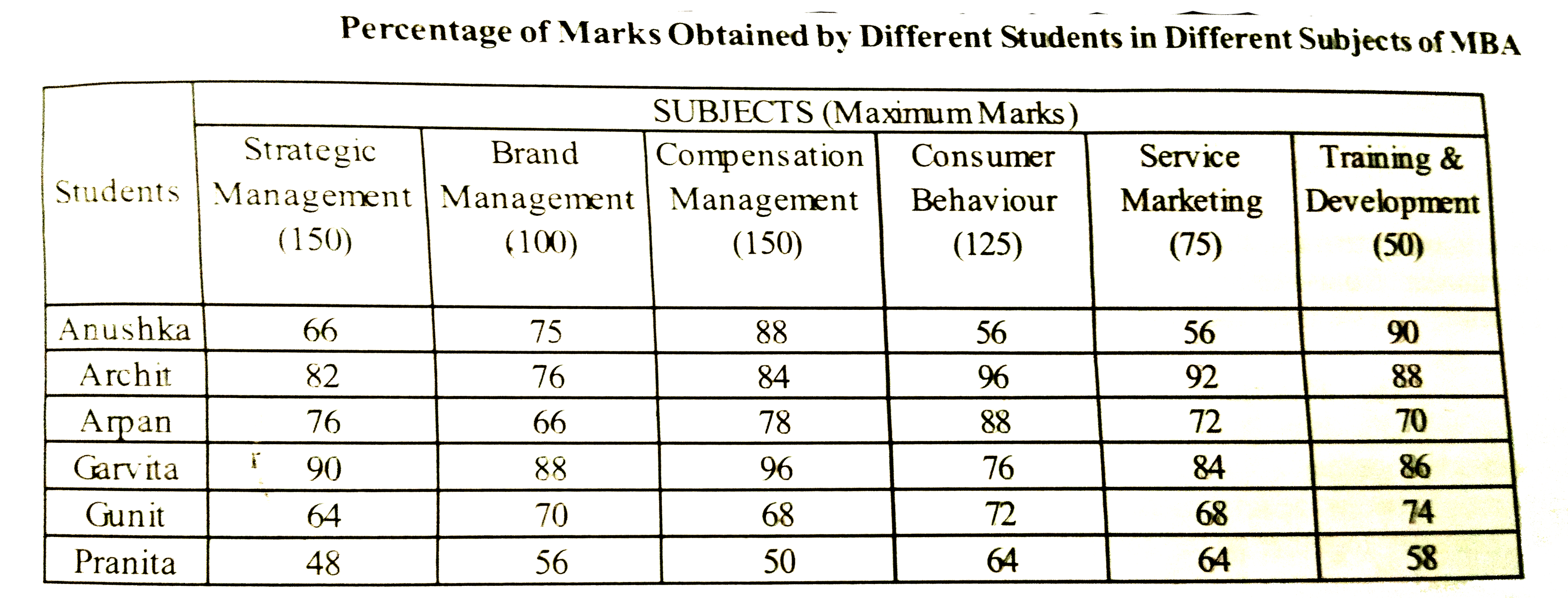
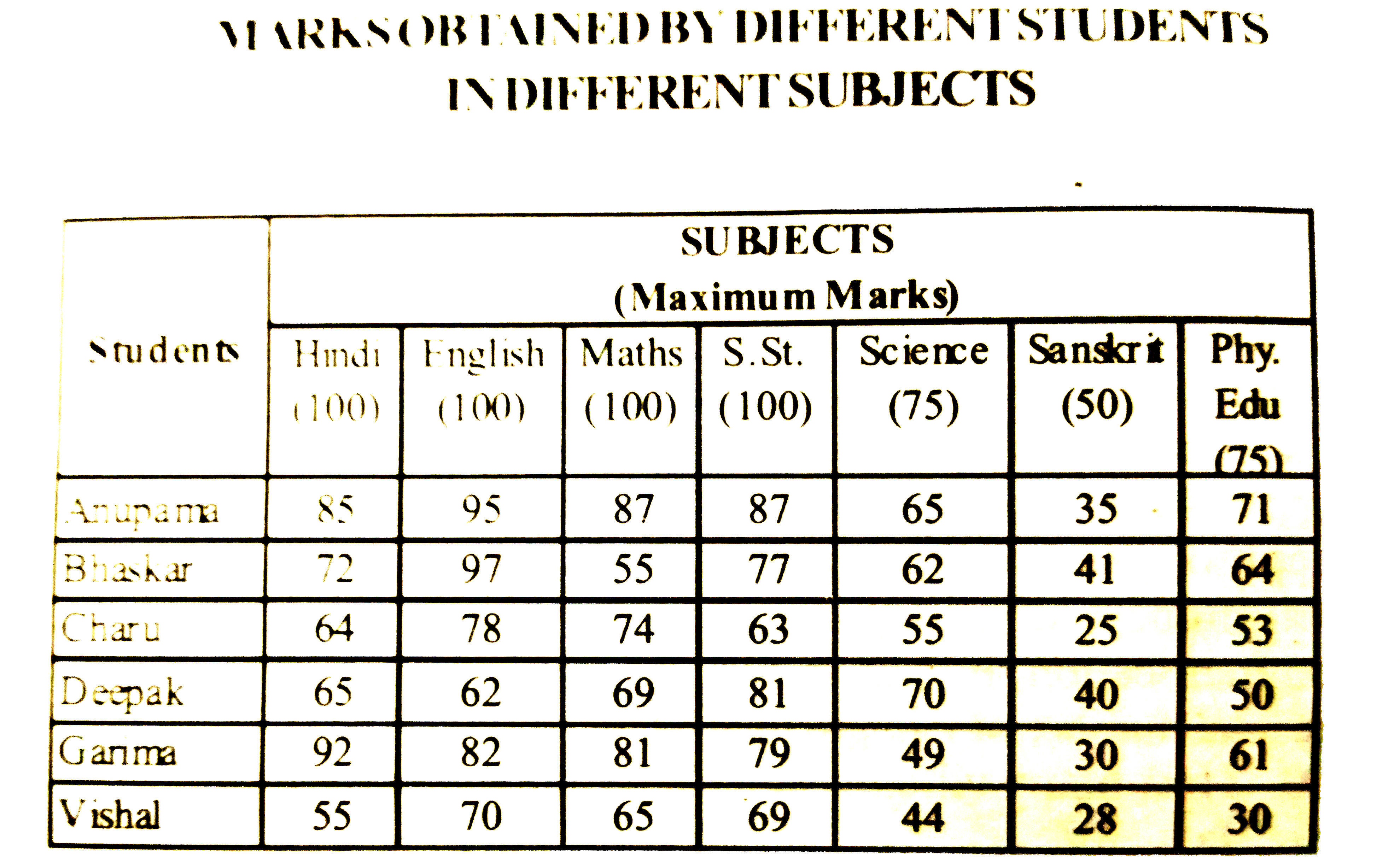
Two students appeared for an examination. One student who scored 20 marks more than the other, his score was 60% of the sum of their marks. What was his score?

Options

A. 80
B. 20
C. 40
D. 60

Answer

D

Explanation

To solve the problem step by step, we will denote the scores of the two students as follows:

1. Let the score of the first student be \( x \).
2. Since the second student scored 20 marks more than the first, his score will be \( x + 20 \).

Now, according to the problem, the score of the second student is also 60% of the sum of their scores. The sum of their scores can be calculated as:

\( \text{Sum of scores} = x + (x + 20) = 2x + 20 \)

Now, we can express the condition given in the problem mathematically:

\( x + 20 = 0.6 \times (2x + 20) \)

Next, we will simplify the equation:

1. Expand the right side:
\( x + 20 = 0.6 \times 2x + 0.6 \times 20 \)
\( x + 20 = 1.2x + 12 \)

2. Rearranging the equation to isolate \( x \):
\( x + 20 - 12 = 1.2x \)
\( x + 8 = 1.2x \)

3. Subtract \( x \) from both sides:
\( 8 = 1.2x - x \)
\( 8 = 0.2x \)

4. Now, solve for \( x \):
\( x = \frac{8}{0.2} = 40 \)

Now that we have the score of the first student, we can find the score of the second student:

\( \text{Score of the second student} = x + 20 = 40 + 20 = 60 \)

Thus, the score of the second student is 60.

Summary of Steps:
1. Let the first student's score be \( x \) and the second student's score be \( x + 20 \).
2. Set up the equation based on the condition given in the problem.
3. Simplify the equation to isolate \( x \).
4. Solve for \( x \) to find the score of the first student.
5. Calculate the score of the second student.