If a capacitor connected to a battery is fully charged, then a dielectric (k) is inserted between plates then increase in the potential energy is

k times

k-1 times

$\frac{1}{k}$ times

$k^{2}$ times

Video Solution

Text Solution

Generated By DoubtnutGPT

To solve the problem of how much the potential energy increases when a dielectric is inserted into a fully charged capacitor connected to a battery, we can follow these steps:
1. Understand the Initial Setup:
- We have a parallel plate capacitor connected to a battery with voltage $V$ .
- The initial capacitance of the capacitor is $C_{0}$ .
2. Calculate Initial Potential Energy:
- The potential energy ( $U_{i}$ ) stored in the capacitor when it is fully charged is given by the formula:
$U_{i} = \frac{1}{2} C_{0} V^{2}$
- This is our initial potential energy.
3. Introduce the Dielectric:
- When a dielectric with dielectric constant $k$ is inserted between the plates of the capacitor, the capacitance increases to:
$C^{'} = k C_{0}$
4. Calculate Final Potential Energy:
- The new potential energy ( $U_{f}$ ) after inserting the dielectric is given by:
$U_{f} = \frac{1}{2} C^{'} V^{2} = \frac{1}{2} (k C_{0}) V^{2} = \frac{1}{2} k C_{0} V^{2}$
5. Relate Final Potential Energy to Initial Potential Energy:
- We can express the final potential energy in terms of the initial potential energy:
$U_{f} = k U_{i}$
6. Calculate the Change in Potential Energy:
- The change in potential energy ( $Δ U$ ) when the dielectric is inserted is given by:
$Δ U = U_{f} - U_{i}$
- Substituting the expressions we found:
$Δ U = k U_{i} - U_{i} = (k - 1) U_{i}$
Final Answer:
The increase in potential energy when a dielectric is inserted into a fully charged capacitor is:
$Δ U = (k - 1) U_{i}$

Updated on:18/9/2024

A capacitor is kept connected to the battery and a dielectric slab is inserted between the plates. During this process
ANo work is done
BWork is done at the cost of the energy already stored in the capacitor before the slab is inserted
CWork is done at the cost of the battery
DWork is done at the cost of both the capacitor and the battery
While a capacitor remains connected to a battery and dielectric slab is applied between the plates, then
APotential difference between the plates is changed
BChange flows from the battery to the capacitor
CElectric field between the plates increases
DEnergy store in the capacitor decreases
Two identical capacitor $C_{1}$ and $C_{2}$ are connected in series with a battery. They are fully charged. Now a dielectric slab is inserted between the plates of $C_{2}$ . The potential across $C_{1}$ will :

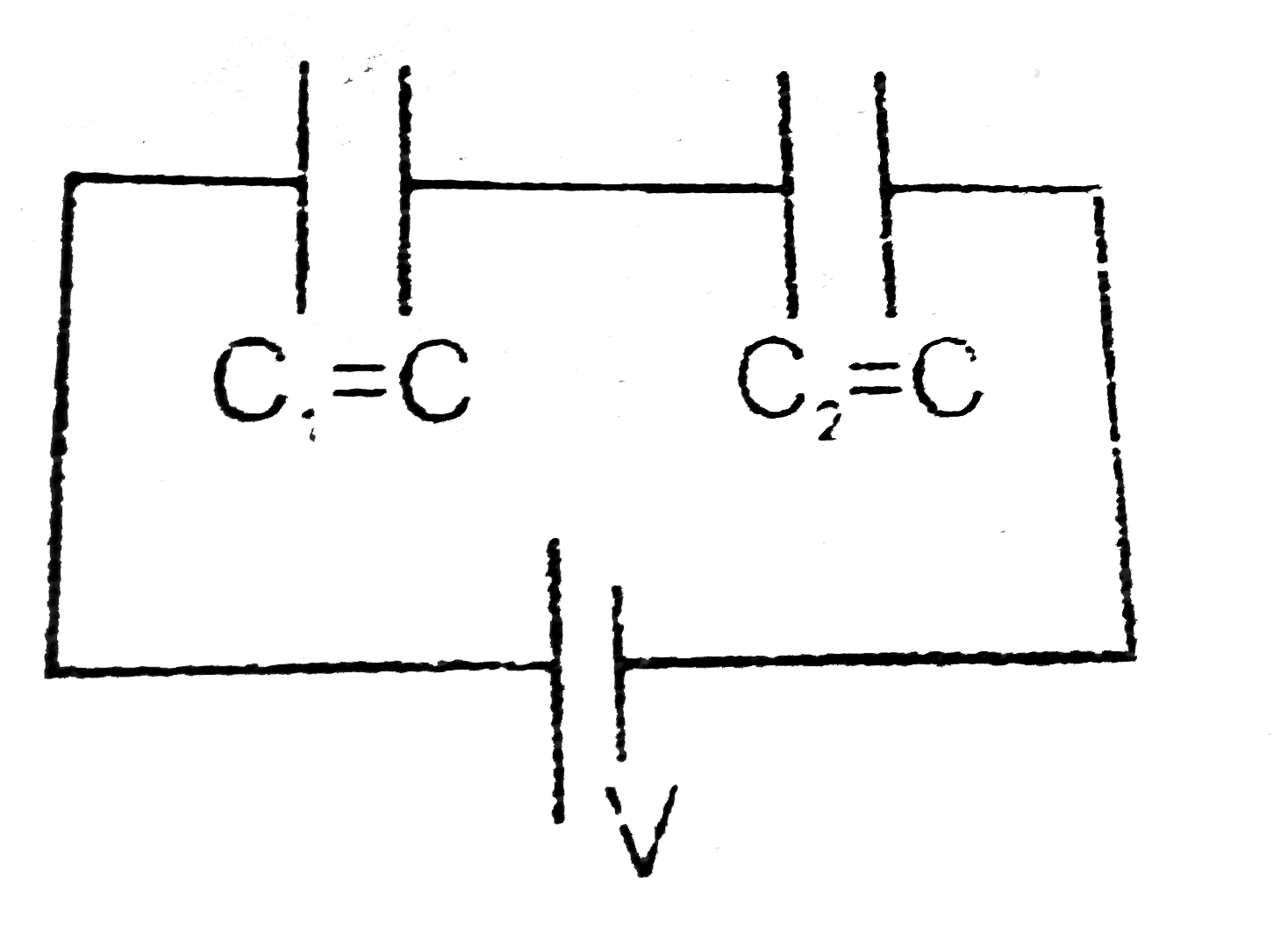Aincrease
Bdecrease
Cremain same
Ddepend on internal resistance of the cell