यदि ∫xpq−p−1(xp+1)qdx=2(1+x−p)1−qλp(q−1)+c(p,q∈N−{1}) है तब λ का मान है ( यहां c एक स्वेच्छ अचर है)
The correct Answer is:B
Step by step video, text & image solution for यदि int(x^(pq-p-1))/((x^(p)+1)^(q))dx=(2(1+x^(-p))^(1-q))/(lamdap(q-1))+c(p, q in N-{1}) है तब lamda का मान है ( यहां c एक स्वेच्छ अचर है) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
मान लीजिये की x, y, z धनात्मक वास्तविक संख्याएँ इस प्रकार है की वे GP में है और tan−1 x , tan−1y तथा tan−1z तीनों AP में है, तो निम्नलिखित में से कौन-सा सही है?
View Solutionएक यादृच्छिक चर X का प्रायिकता बंटन निम्न रूप में दिया गया है:
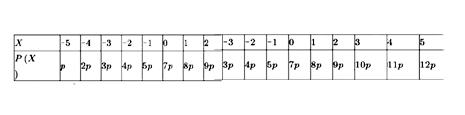
तब, p का मान है
View Solutionif |x|<1 then d(dx)[1+(p)/(q)x+(p(p+q))/(2!)((x)/(q))^(2)+
View SolutionIf y = 11+xp−r+xq−r+11+xp−q+xr−q+11+xq−p+xr−p,thendydx=
View Solution∫dxx2014+x=1pln(xq1+xr)+C where p,qr∈N then the value of (p+q+r) equals (Where C is constant of integration)
View Solution(p+1q)p.(p−1q)q(q+1p)p.(q−1p)q=(pq)x then x=
View SolutionIf ∫xpq−p−1(xp+1)qdx=2(1+x−p)1−qλp(q−1)+c (p,q∈N−{1}), then the value of λ is (here, c is an arbitary constant)
View Solutionयदि ∫(x+1)√2x−1dx=f(x)√2x−1+C है, जहाँ C एक समाकलन अचर है, तो f(x) बराबर है
View Solutionयदि P(A) =P(B) =x और P(A∩B)=P(A'∩B')=13, तब x का मान है
View Solution∫pxp+2q−1−qxq−1x2p+2q+2xp+q+1dx is equal to
(1) −xpxp+q+1+C
(2) xqxp+q+1+C
(3) −xqxp+q+1+C
(4) xpxp+q+1+C
View SolutionThe base BC of a ABC is bisected at the point (p,q) & the equation to the side AB&AC are px+qy=1 & qx+py=1 . The equation of the median through A is: (p−2q)x+(q−2p)y+1=0 (p+q)(x+y)−2=0 (2pq−1)(px+qy−1)=(p2+q2−1)(qx+py−1) none of these
View SolutionThe value of p and q(p≠0,q≠0) for whichp,q are the roots of the equation x2+px+q=0 are (a)p=1,q=−2 (b) p=−1,q=−2 (c) p=−1,q=2 (d) p=1,q=2
View SolutionIf ∫1ex+1dx=px−qlog|1+ex|+C then p+q=….........
View Solution∫dxx2014+x=1pln(xq1+xr)+C where p,qr∈N then the value of (p+q+r) equals (Where C is constant of integration)
View Solution(a) If r2=pq, show that p:q is the duplicate ratio of (p+r) : (q+r).
(b) If (p−x):(q−x) be the duplicate ratio of p:q then show that : 1p+1q=1r.
View SolutionIf ((p^-1 q^2)/(p^3 q^-2))^(1/3) div ((p^6 q^-3)/(p^-2 q^3))^(1/3) =p^a q^b , then the value of a+b where p and q are different positive primes is- यदि ((p^-1 q^2)/(p^3 q^-2))^(1/3) div ((p^6 q^-3)/(p^-2 q^3))^(1/3) =p^a q^b है, तो a+b का मान क्या है जिसमें p और q विभिन्न धनात्मक अभाज्य है?
View Solution
