A coaxial cable consists of an inner wire of radius 'a' surrounded by an outer shell of inner and outer radii 'b' and 'c respectively. The inner wire carries an electric current i0. which is distributed uniformly across cross-sectional area. The outer shell carries an equal current in opposite direction and distributed uniformly. What will be the ratio of the magnetic field at a distance x from the axis when (i)x<aand(ii)a<x<b ?
b2−a2x2
a2x2
x2a2
x2b2−a2
To solve the problem, we need to analyze the magnetic field in two different regions of a coaxial cable:
1. When x<a (inside the inner wire)
2. When a<x<b (between the inner wire and the inner surface of the outer shell)
Step 1: Magnetic Field for x<a
When x<a, we are inside the inner wire. The current i0 is uniformly distributed across the cross-sectional area of the wire.
- Current Density J:
J=i0πa2
- Enclosed Current i′ at radius x:
The area at radius x is πx2, so the enclosed current is:
i′=J⋅Area=i0πa2⋅πx2=i0x2a2
- Using Ampere's Law:
∮B⋅dl=μ0i′
The circumference of the loop at radius x is 2πx:
B⋅(2πx)=μ0(i0x2a2)
Therefore, the magnetic field B1 is:
B1=μ0i0x2πa2
Step 2: Magnetic Field for a<x<b
When a<x<b, we are outside the inner wire but inside the outer shell.
- Enclosed Current:
In this region, the only current contributing to the magnetic field is i0 from the inner wire, as the outer shell carries an equal current in the opposite direction, which does not contribute to the enclosed current:
i′=i0
- Using Ampere's Law:
∮B⋅dl=μ0i′
The circumference of the loop at radius x is still 2πx:
B⋅(2πx)=μ0i0
Therefore, the magnetic field B2 is:
B2=μ0i02πx
Step 3: Ratio of Magnetic Fields
Now, we need to find the ratio B1B2:
B1B2=μ0i0x2πa2μ0i02πx=x2a2
Final Answer
Thus, the ratio of the magnetic field at a distance x from the axis is:
B1B2=x2a2
2. When a<x<b (between the inner wire and the inner surface of the outer shell)
J=i0πa2
The area at radius x is πx2, so the enclosed current is:
i′=J⋅Area=i0πa2⋅πx2=i0x2a2
∮B⋅dl=μ0i′
The circumference of the loop at radius x is 2πx:
B⋅(2πx)=μ0(i0x2a2)
Therefore, the magnetic field B1 is:
B1=μ0i0x2πa2
In this region, the only current contributing to the magnetic field is i0 from the inner wire, as the outer shell carries an equal current in the opposite direction, which does not contribute to the enclosed current:
i′=i0
∮B⋅dl=μ0i′
The circumference of the loop at radius x is still 2πx:
B⋅(2πx)=μ0i0
Therefore, the magnetic field B2 is:
B2=μ0i02πx
B1B2=μ0i0x2πa2μ0i02πx=x2a2
Thus, the ratio of the magnetic field at a distance x from the axis is:
B1B2=x2a2
Topper's Solved these Questions
Similar Questions
Consider a coaxial cable which consists of an inner wire of radius a surrounded by an outer shell of inner and outer radii b and c respectively. The inner wire carries an electric current (i0) and the outer shell carries an equal current in opposite direction. Find the magnetic field at a distance x from the axis where (a) x<a, (b) a<x<b, (c) b<x<c and (d)x>c. Assume that the current density is uniform in the inner wire and also uniform in the outer shell.
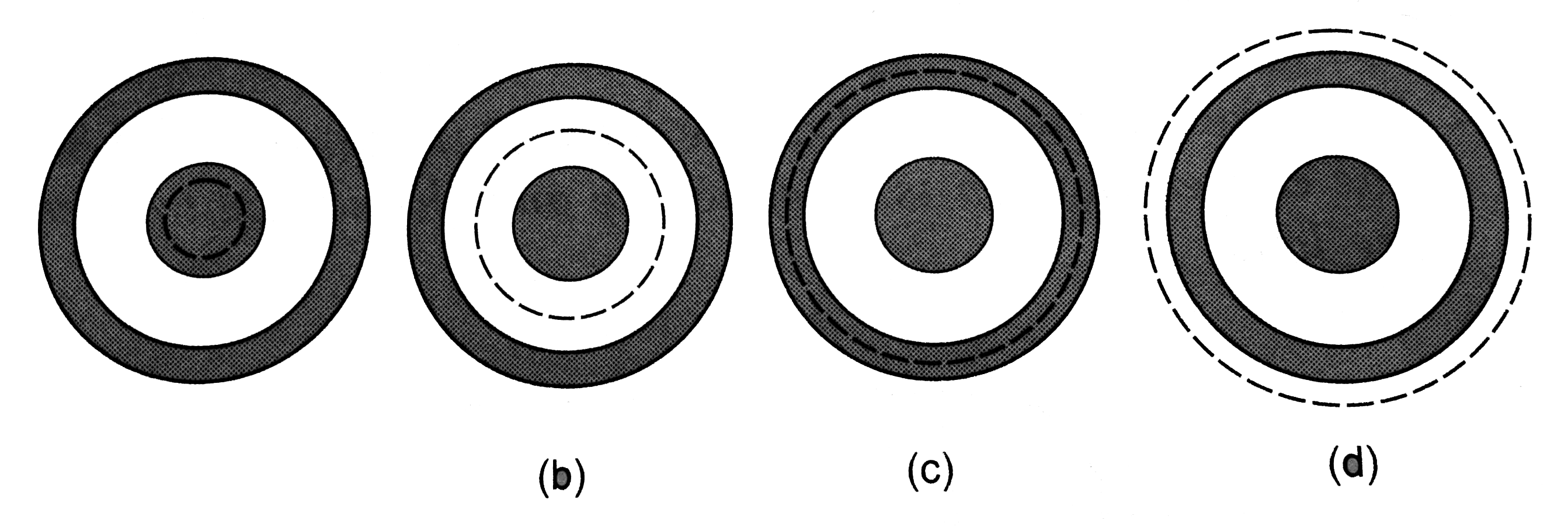
View SolutionA long, cylindrical wire of radius b carries a current i distributed uniformly over its cross section. Find the magnitude of the magnetic field at a point inside the wire at a distance a from the axis.
View Solution
Knowledge Check
Consider a ????cable which consists of an inner wilre of radius a surrounded by an outer shell of inner and outer radii b and c respectively. The inner wire caries a current I and outer shell carries an equal and opposite current. The magnetic field at a distance x from the axis where b<x<c is
Aμ0I(c2−b2)2πx(c2−a2)Bμ0I(c2−x2)2πx(c2−a2)Cμ0I(c2−x2)2πx(c2−b2)DzeroA long cylindrical wire carries a steady current which is uniformly distributed across its cross section. Which of the following is true?
AMagnetic field is zero at the centre.BElectric field is zero at the centre.CElectric field just outside the cylinder is zero.DMagnetic field just outside the cylinder is zero.A long cylinder of wire of radius 'a' carries a current i distributed uniformly over its cross section. If the magnetic fields at distances r<aandR>a from the axis have equal magnitude, then
Aa=R+r2Ba=√ReCa=Rr/(R+r)Da=R2/r
A long, straight wire of radius R carries a current distributed uniformly over its cross section. The magnitude of the magnetic field is
View SolutionA long straight solid metal wire of radius R carries a current I, uniformly distributed over its circular cross-section. Find the magnetic field at a distance r from axis of wire (i) inside and (ii) outside the wire.
View SolutionA long straight wire of a circular cross-section (radius r) carries a steady current I. The current is distributed uniformly across the cross-section. Calculare the magnetic field at a point (a) outside the wire , (b) inside the wire. Draw a graph showing variation of magnetic field.
View SolutionThe adjoining figure shows a long straight wire of a circular cross section (radius a) carrying steady current I. The current I is uniformly distributed across its cross section. Calculate the magnetic field at a distance from its axis for (i)r<a,(ii)r>a.
View SolutionA cylindrical long wire of radius R carries a current I uniformly distributed over the cross sectional area of the wire. The magnetic field at a distance x from the surface inside the wire is
View Solution
