Let IA and IB be moments of inertia of a body about two axes A and B respectively. The axis A passes through the centre of mass of the body but B does not. ThenSolution in Tamil
IA<IB
IfIA<IB the axes pareparallel
If the axes are parallel IA<IB
If the axes are not parallel IA≥IB
The correct Answer is:C
Step by step video & image solution for Let I_(A) and I_(B) be moments of inertia of a body about two axes A and B respectively. The axis A passes through the centre of mass of the body but B does not. Then by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Exercises
86 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Objective -1
26 Videos
- REST AND MOTION : KINEMATICSBOOK - HC VERMACHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Exercises
51 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMACHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercises
58 Videos
Similar Questions
A wheel is making revolutions about its axis with uniform angular acceleration. Starting from rest, till it reaches 100 rev/sec in 4 seconds. Find the angular acceleration.
View SolutionLet →A be a unit vector along the axis of rotation of a purely rotating body and →B be a unit vector along the velocity of a particle P of the body away from the axis. The value of →A.→B is
View SolutionA man goes 10 m due east and then 24 m due north. Find the distance of his current position from the starting point ?
View SolutionA particle executes simple harmonic motion with an amplitude of 10 cm and time period 6s. At t=0 it is at position x=5 cm going towards positive x-direction. Write the equation for the displacement x at time t. Find the magnitude of the acceleration of the particle at t=4s.
View SolutionTwo rigid bodies A and B rotate with angular momenta LA and LB respectively. The moments of inertia of A and B about the axes of rotation are IA and IB respectively. If IA=IB/4 and LA=5LB, then the ratio of rotational kinetic energy KA of A to the rotational kinetic energy KB of B is given by
View SolutionLet I1 and I2 be the moment of inertia of a uniform square plate about axes APC and OPO respectively as shown in the figure.P is centre of square. The ratio I1I2 moment of inertia is -
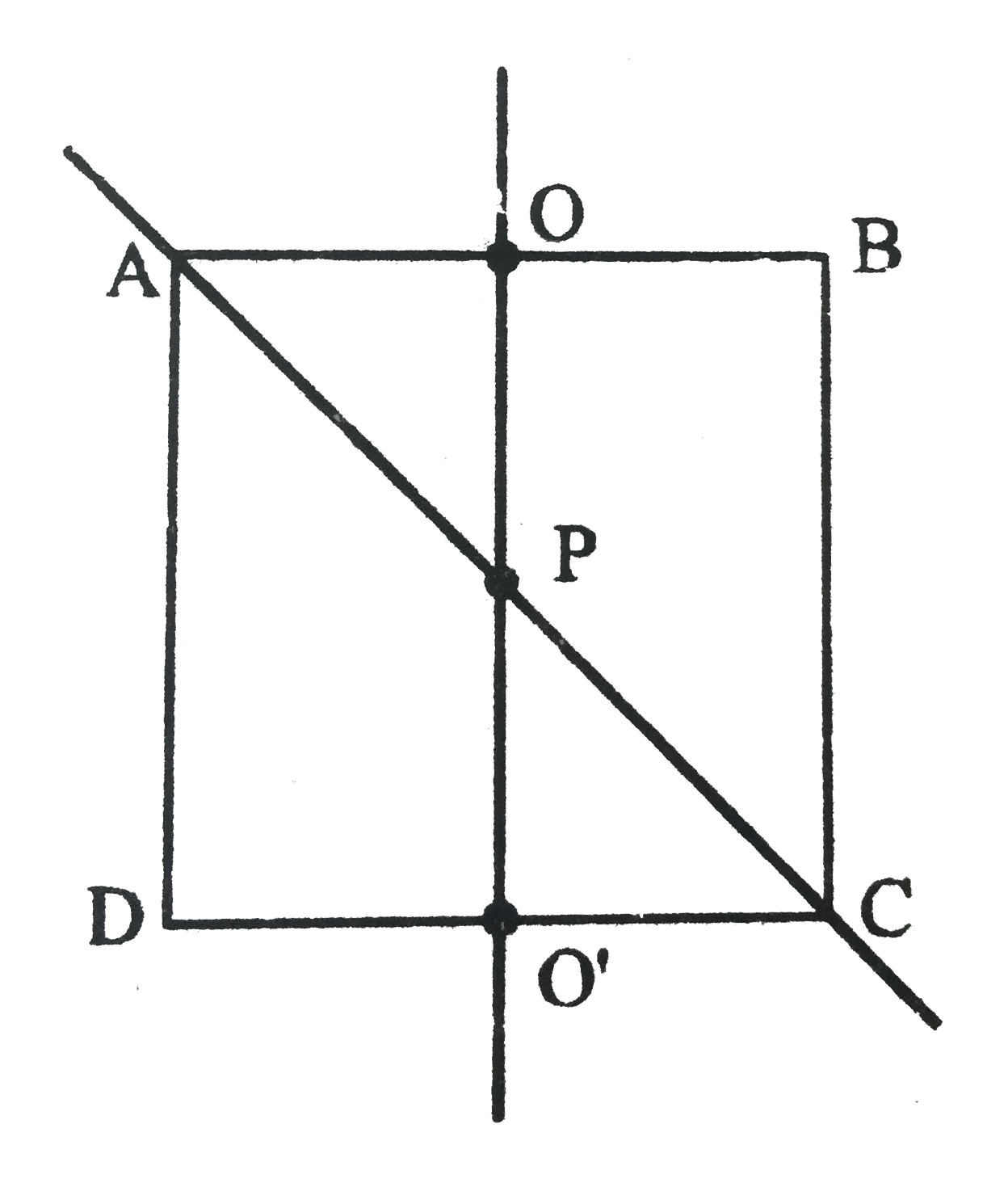 .
.
View SolutionIf I0 is the moment of inertia body about an axis passing through its center of mass. The moment about a parallel axis and at distance d is I=I0+Md2. The variation of I with d is
View SolutionTwo rigid bodies A and B rotate with rotational kinetic energies E_(A) and E_(B) respectively. The moments of inertia of A and B about the axis of rotation are I_(A) and I_(B) respectively. If I_(A) = I_(B)//4 and E_(A)= 100 E_(B), the ratio of angular momentum (L_(A)) of A to the angular momentum (L_(B)) of B is
View SolutionTwo rotating bodies A and B of masses m and 2m with moments of inertia I_(A) and I_(B) (I_(B) gt I_(A)) have equal kinetic energy of rotation. If L_(A) and L_(B) be their angular momenta respectively, then
View SolutionTwo circular loops A and B of radii R and 2R respectively are made of the similar wire. Their moments of inertia about the axis passing through the centre of perpendicular to their plane are IA and IB respectively. The ratioIAIB is :
View SolutionMoment of inertia of a rigid body about an axis passing through its centre of mass is I_(0) and moment of inertia of the same body about another axis parallel to the first axis is I. Then
View SolutionAB is a stick half of which is wood and the other half steel. IA is moment of inertia about an axis passing through A and perpendicular to the length . IB is moment of inertia about an axis passing through B and perpendicular to the length. Then
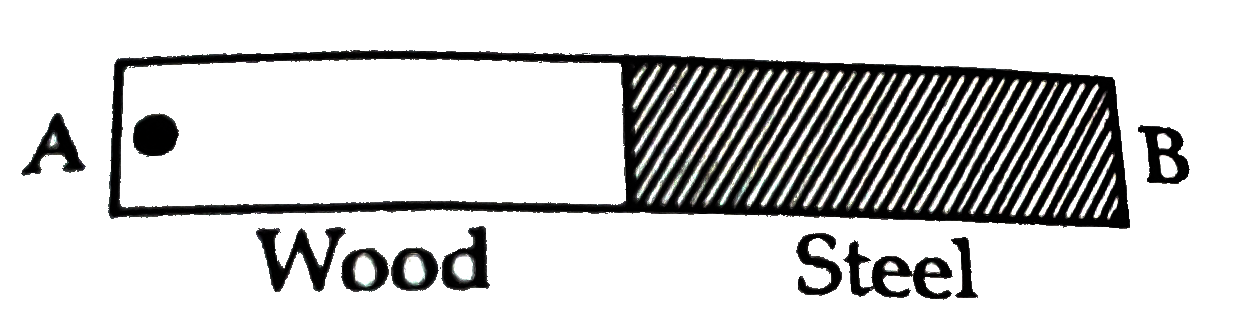
View SolutionIn the figure shown, a planer body is in the shape of a rhombus ABCD: I_(1) and I_(2) are moment of inertias: about axis passing through centre and perpendicular to sides BC and DC respectively. I_(3) and I_(4) are amount of inertias about diagonal axis BD and AC respectively. Then the moment of inertia about an axis passing through centre and perpendicular tothe plane of body is given by
View SolutionIf I1 is the moment of inertia of a thin rod about an axis perpendicular to its length and passing through its centre of mass, and I2 is the moment of inertia (about central axis) of the ring formed by bending the rod, then
View SolutionTwo rotating bodies A and B of masses mand 2m with moments of inertia IAandIB(IB>IA) have equal kinetic energy of rotation. If LAandLB be their angular momenta respectively, then
View Solutionदो घूर्णन पिण्ड A एवं B के जड़त्व आघूर्ण IA एवं IB (IA>IB) हैं। यदि इनके कोणीय संवेग बराबर हों, तो-
View Solution
