The mean score of 3 students in a test out of 25 is 18. Two new students take the test. What is the lowest marks that can be obtained by one newcomer who scores less than the other one for the overall average of the five students to rise to 20 ?
22
21
23
20
The correct Answer is:B
To solve the problem, let's break it down step by step.
Step 1: Calculate the total score of the first three students.
The mean score of the three students is 18. To find the total score of these students, we can use the formula for mean:
Mean=Total ScoreNumber of Students
Given that the mean is 18 and the number of students is 3, we can rearrange the formula to find the total score:
Total Score=Mean×Number of Students=18×3=54
Step 2: Determine the desired total score for five students.
We want the average score of all five students to be 20. Therefore, we can calculate the total score needed for five students:
Total Score for 5 Students=Mean×Number of Students=20×5=100
Step 3: Calculate the total score needed from the two new students.
Now, we need to find out how much score the two new students must achieve together to reach the total score of 100:
Total Score from 2 New Students=Total Score for 5 Students−Total Score of 3 Students=100−54=46
Step 4: Set up the equation for the scores of the two new students.
Let’s denote the score of the first newcomer as x and the score of the second newcomer as y. We know that x<y and:
x+y=46
Step 5: Find the lowest score for the first newcomer.
To find the lowest score for the first newcomer x, we can express y in terms of x:
y=46−x
Since x<y, we can substitute y:
x<46−x
Now, solving for x:
2x<46⟹x<23
Step 6: Determine the maximum possible value for x.
Since x must be less than 23, the highest integer value x can take is 22. Therefore, if x=22, we can find y:
y=46−22=24
Conclusion:
The lowest score that can be obtained by the newcomer who scores less than the other one is:
22
The mean score of the three students is 18. To find the total score of these students, we can use the formula for mean:
We want the average score of all five students to be 20. Therefore, we can calculate the total score needed for five students:
Now, we need to find out how much score the two new students must achieve together to reach the total score of 100:
Let’s denote the score of the first newcomer as x and the score of the second newcomer as y. We know that x<y and:
To find the lowest score for the first newcomer x, we can express y in terms of x:
Since x must be less than 23, the highest integer value x can take is 22. Therefore, if x=22, we can find y:
The lowest score that can be obtained by the newcomer who scores less than the other one is:
Similar Questions
Knowledge Check
- Question 1 - Select One
The mean score of three students in a test out of 45 is 38. Two new students take the test. What is the lowest mark that can be obtained by the newcomer who scores less than the other new student for the overall average of the five students to rise to 40 ?
A40B42C41D43 - Question 2 - Select One
In a class test, a student scored 22 marks out of 25 marks . The student 's marks in percentage, is
A88B80C90D75 - Question 3 - Select One
In a class test, a student scored 8 marks out of 25 marks. The student's marks in percentage is:
A32B35C29D30
In question 2 above, the number of students who scored marks less than 4 is
View SolutionIn a class test, a student scored 8 marks out of 25 marks. The student's marks in percentage is:
View SolutionIn a class test, a student scored 21 marks out of 25 marks. The student's marks in percentage terms is:
View SolutionIn a class test, a student scored 21 marks out of 25 marks. The student's marks in percentage terms is:
View SolutionThe marks (maximum marks 100) obtained by 20 students in a test are given below :
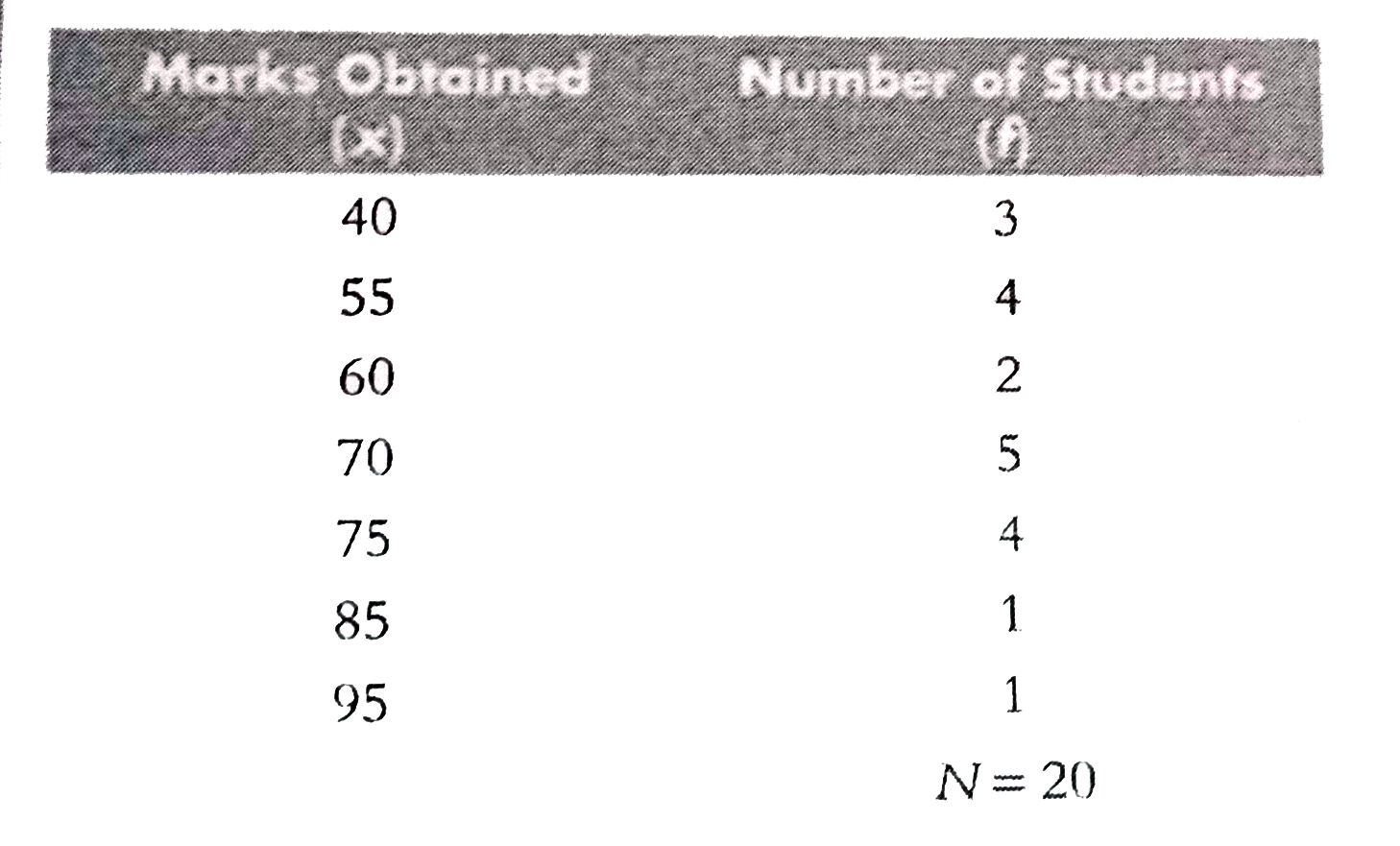
Find the mean marks of the 20 students.View Solution