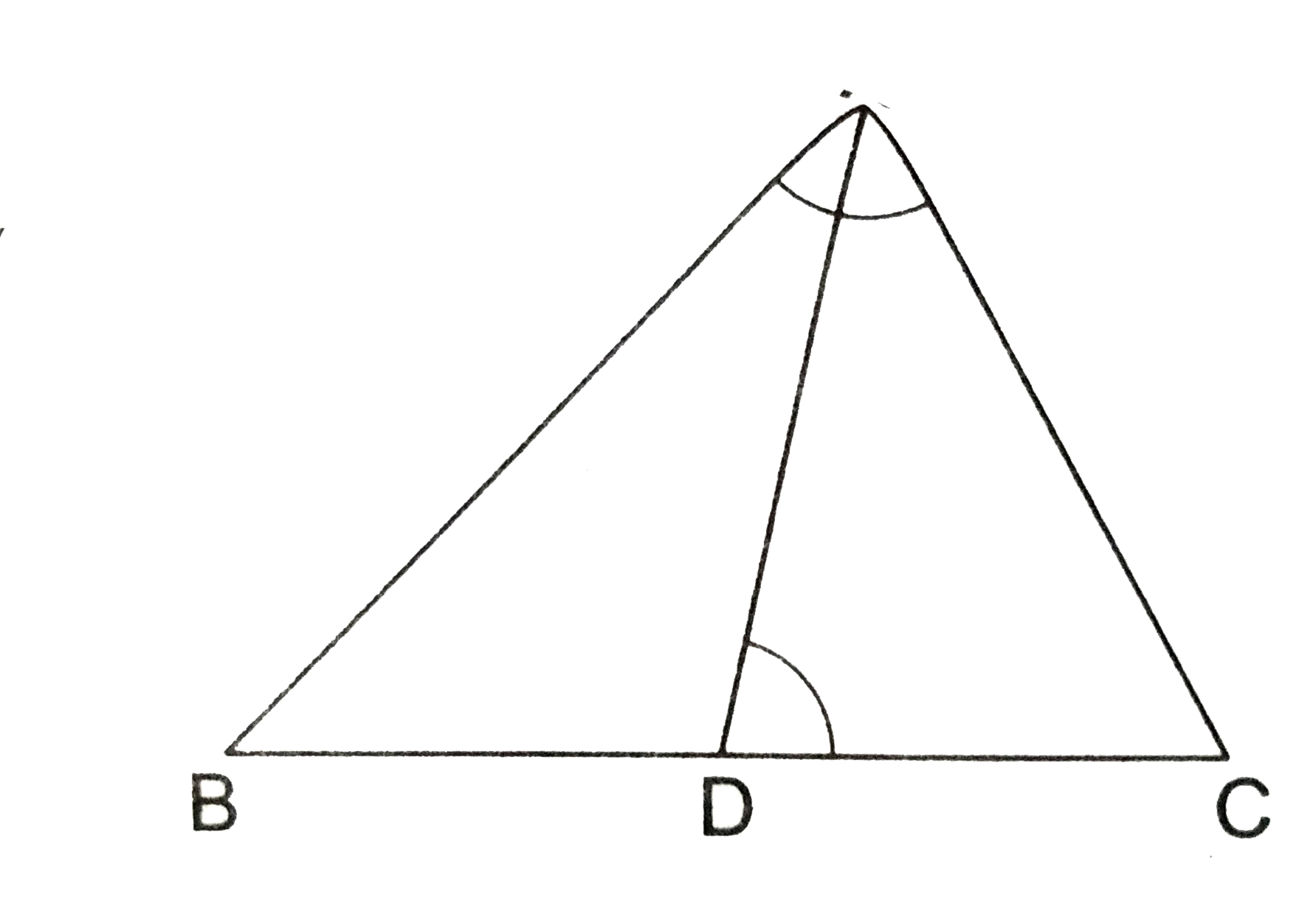
In △ABC,D is point on BC such that ∠BAD=12∠ADC,∠BAC=84∘and∠C=42∘. What is the measure of ∠ADB ?
102∘
68∘
94∘
72∘
The correct Answer is:D
To solve the problem step by step, let's follow the reasoning laid out in the video transcript.
Step 1: Understand the given information
We have triangle ABC with:
- ∠BAC=84∘
- ∠C=42∘
- D is a point on BC such that ∠BAD=12∠ADC
Step 2: Find ∠ABC
Using the property that the sum of angles in a triangle is 180∘:
∠ABC+∠BAC+∠C=180∘
Substituting the known angles:
∠ABC+84∘+42∘=180∘
∠ABC+126∘=180∘
∠ABC=180∘−126∘=54∘
Step 3: Set up the angles involving point D
Let ∠ADC=2x. According to the problem:
∠BAD=x
Since ∠BAD=12∠ADC, we have:
∠BAD=xand∠ADC=2x
Step 4: Use the exterior angle theorem in triangle ADB
In triangle ADB, the exterior angle ∠ADC is equal to the sum of the two opposite interior angles:
∠ADC=∠ADB+∠ABD
Substituting the known values:
2x=∠ADB+54∘
Step 5: Solve for x
From the equation 2x=∠ADB+54∘, we can express ∠ADB as:
∠ADB=2x−54∘
Step 6: Find the value of x
Using the triangle ADB's interior angles, we know:
∠ADB+∠BAD+∠ABD=180∘
Substituting the known angles:
(2x−54∘)+x+54∘=180∘
Simplifying:
3x=180∘
x=60∘
Step 7: Find ∠ADB
Now substituting x back into the expression for ∠ADB:
∠ADB=2(60∘)−54∘
∠ADB=120∘−54∘=66∘
Step 8: Find the final angle
Now we have:
∠ADB+54∘+54∘=180∘
This gives:
∠ADB+108∘=180∘
∠ADB=180∘−108∘=72∘
Thus, the measure of ∠ADB is 72∘.
Final Answer
∠ADB=72∘
We have triangle ABC with:
- ∠BAC=84∘
- ∠C=42∘
- D is a point on BC such that ∠BAD=12∠ADC
Using the property that the sum of angles in a triangle is 180∘:
∠ABC+∠BAC+∠C=180∘
Substituting the known angles:
∠ABC+84∘+42∘=180∘
∠ABC+126∘=180∘
∠ABC=180∘−126∘=54∘
Let ∠ADC=2x. According to the problem:
∠BAD=x
Since ∠BAD=12∠ADC, we have:
∠BAD=xand∠ADC=2x
In triangle ADB, the exterior angle ∠ADC is equal to the sum of the two opposite interior angles:
∠ADC=∠ADB+∠ABD
Substituting the known values:
2x=∠ADB+54∘
From the equation 2x=∠ADB+54∘, we can express ∠ADB as:
∠ADB=2x−54∘
Using the triangle ADB's interior angles, we know:
∠ADB+∠BAD+∠ABD=180∘
Substituting the known angles:
(2x−54∘)+x+54∘=180∘
Simplifying:
3x=180∘
x=60∘
Now substituting x back into the expression for ∠ADB:
∠ADB=2(60∘)−54∘
∠ADB=120∘−54∘=66∘
Now we have:
∠ADB+54∘+54∘=180∘
This gives:
∠ADB+108∘=180∘
∠ADB=180∘−108∘=72∘
∠ADB=72∘
Topper's Solved these Questions
- SSC SELECTION POST (6 NOV 2020) SHIFT 3 HSCBOOK - SSC SELECTION POSTCHAPTER - SSC SELECTION POST (6 NOV 2020) SHIFT 3 HSCEXERCISE - SECTION : QUANTITATIVE APTITUDE
25 Videos
- SSC SELECTION POST 10 NOV 2020 SHIFT 1 MATRICULATION LEVELBOOK - SSC SELECTION POSTCHAPTER - SSC SELECTION POST 10 NOV 2020 SHIFT 1 MATRICULATION LEVELEXERCISE - Section : Quantitative Aptitude
25 Videos
Similar Questions
The following the pie chart represent the distrubution of candidates who were enrolled for an entrance examination and the candidates (out of those enrolled) wh passed the examination in different instritutes (P,Q,R,S,T,V,X) Study the charts and answer the question that follows.
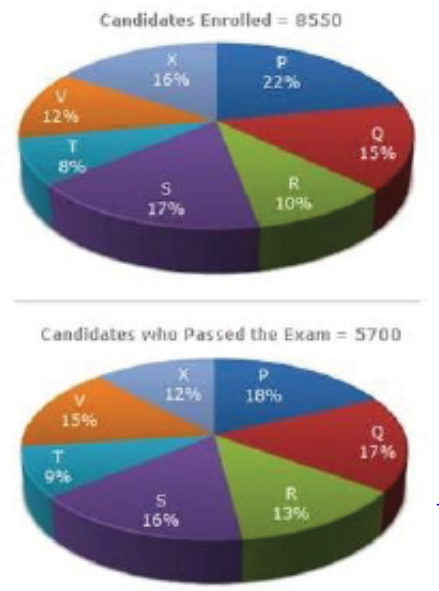
The number of candidates who passed from insitutues P and Q toghther exceeds the number of candidates who enrolled from institutes T and R together by :
View SolutionWhich of the following is divisible by 99?
View Solutionएक त्रिभुज ABC की भुजा पर एक बिंदु D इस प्रकार स्थित है कि ∠ADC=∠BAC है दर्शाइए कि CA2=CB.CD है
View SolutionIn the given figure , D is a point on the side BC of ΔABC such that ∠ADC=∠BAC. Prove that CA2=CB×CD.
View SolutionTriangle ABC has angle BAC equal to 90∘. What is the measure of the angle ABC? A. The angle ABC is 35∘ B. The angle CBA is 55∘
View SolutionIn △ ABC, AB = AC and the bisector of ∠A< intersects BC at D. Prove that, ( 1 )△ADB=△ADC(2)∠ABC=∠ACB
View SolutionABC is an isosceles triangle with AB = AC and D is on side BC such that AD _|_ BC Is. angle BAD = angle CAD To prove it, a student followed the following procedure: Delta ABD And Delta ACD In, AB = AC (Given) angle B = angle C (Because AB = AC) And angle ADB = angle ADC (each 90^(@) , Therefore, Delta ABD ~= Delta ACD (A AS) So, angle BAD = angle CAD (CPCT) What is missing in the above arguments?
View SolutionIn ΔABC , the median AD divides ∠BAC such that ∠BAD:∠CAD=2:1. Then cos(A/3) is equal to
View Solution△ABC में यदि D,BC पर कोई बिंदु इस प्रकार है ABAC=BDDC की हो, एवं ∠B=70∘,∠C=50∘ हो, तो ∠BAD ज्ञात कीजिए।
View SolutionI is the incentre of ΔABC,∠ABC=60∘ and ∠ACB=50∘. Then find ∠BIC.
View SolutionConsider ΔABD such that ∠ADB=20∘ and C is a point on BD such that AB = AC and CD = CA. Then the measure of ∠ABCis :
View Solutionਇੱਕ ਤ੍ਰਿਭੁਜ ABC ਦੀ ਭੁਜਾ BC ਉੱਤੇ ਇਕ ਬਿੰਦੂ D ਇਸ ਤਰ੍ਹਾਂ ਸਥਿਤ ਹੈ ਕਿ ∠ADC=∠BAC ਹੈ। ਦਿਖਾਉ ਕਿ CA2=CB.CD ਹੈ।
View SolutionIn triangle ABC ,D is a point on BC such that angle BAD= 1/2 angle ADC , angle BAC= 87^@ and angle C=42^@ . What is the measure of angle ADB ? त्रिभुज ABC में, D, BC पर स्थित एक बिंदु है जो इस प्रकार है कि 2 angle BAD= 1/2 angle ADC , angle BAC= 87^@ तथा angle C=42^@ है | कोण ADB का माप क्या होगा ?
View SolutionIn triangle ABC , D is a point on the side BC such that angle ADC =2 angle BAD . If angle A=80^@ and angle C = 38^@ , then what is the measure of the angle ADB ? triangle ABC में D, BC पर एक बिंदु है जैसे angle ADC =2 angle BAD . यदि angle A=80^@ और angle C = 38^@ है, तो angle ADB का माप क्या है
View SolutionIn ΔABC,AC=BC and ∠BAC=70∘. Find ∠BCA
View Solution
