A candidate obtains the marks in subjects English 60 Hindi 75 , Mathematics 63 , Physics 59 and Chemistry 55 . In the weights of 1,2,1,3,3 respectively are alloted to the subjects then the candidates weighted mean is
59
615
10
61.5
The correct Answer is:D
To find the weighted mean of the candidate's marks in different subjects, we will follow these steps:
Step 1: List the Marks and Weights
We have the following marks and their corresponding weights:
- English: Marks = 60, Weight = 1
- Hindi: Marks = 75, Weight = 2
- Mathematics: Marks = 63, Weight = 1
- Physics: Marks = 59, Weight = 3
- Chemistry: Marks = 55, Weight = 3
Step 2: Calculate the Total Weight
The total weight (N) is calculated by summing the weights of all subjects:
N=1+2+1+3+3=10
Step 3: Calculate the Weighted Marks
Next, we calculate the weighted marks for each subject by multiplying the marks by their respective weights:
- English: 60×1=60
- Hindi: 75×2=150
- Mathematics: 63×1=63
- Physics: 59×3=177
- Chemistry: 55×3=165
Step 4: Sum the Weighted Marks
Now, we sum all the weighted marks:
Total Weighted Marks=60+150+63+177+165=615
Step 5: Calculate the Weighted Mean
Finally, we calculate the weighted mean using the formula:
Weighted Mean=Total Weighted MarksN=61510=61.5
Conclusion
The candidate's weighted mean is 61.5.
---
We have the following marks and their corresponding weights:
- Hindi: Marks = 75, Weight = 2
- Mathematics: Marks = 63, Weight = 1
- Physics: Marks = 59, Weight = 3
- Chemistry: Marks = 55, Weight = 3
The total weight (N) is calculated by summing the weights of all subjects:
Next, we calculate the weighted marks for each subject by multiplying the marks by their respective weights:
- Hindi: 75×2=150
- Mathematics: 63×1=63
- Physics: 59×3=177
- Chemistry: 55×3=165
Now, we sum all the weighted marks:
Finally, we calculate the weighted mean using the formula:
The candidate's weighted mean is 61.5.
Topper's Solved these Questions
- STATISTICS BOOK - ARIHANT PUBLICATION JHARKHANDCHAPTER - STATISTICS EXERCISE - Exam Booster for Cracking Exam
25 Videos
- SOLVED PAPER 2019BOOK - ARIHANT PUBLICATION JHARKHANDCHAPTER - SOLVED PAPER 2019EXERCISE - SECTION I: MATHEMATICS
48 Videos
- TAXATION BOOK - ARIHANT PUBLICATION JHARKHANDCHAPTER - TAXATION EXERCISE - EXAM BOOSTER
14 Videos
Similar Questions
The mean and standard deviation of marks obtained by 50 students of a class in three subjects, Mathematics, Physics and Chemistry are given below:
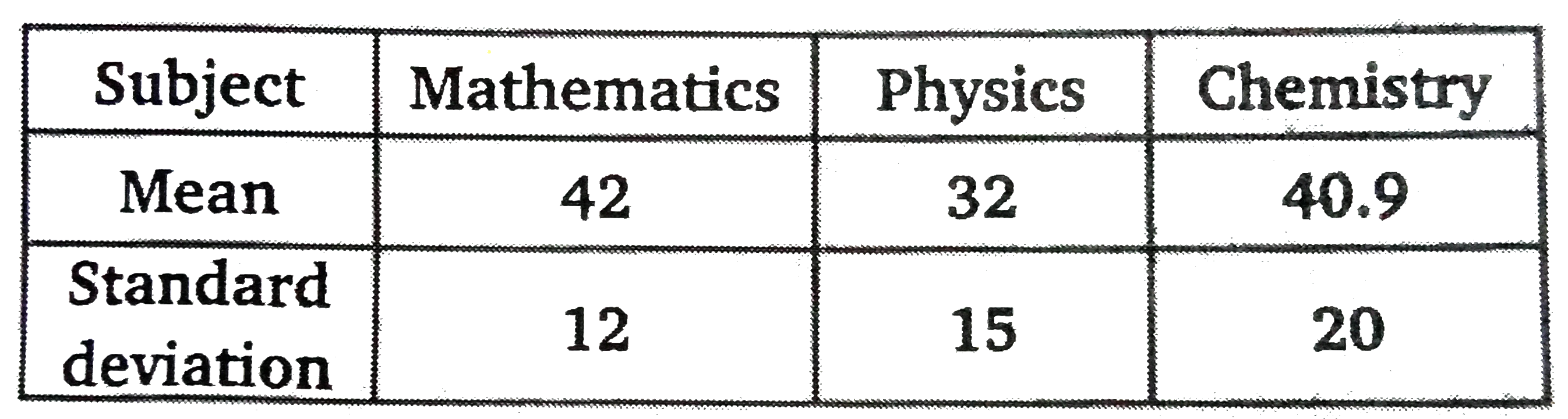
Which of the three subjects shows the highest variability in marks and which shows the lowest?
View SolutionA student obtained 60 marks in English, 75 in Hindi, 63 in Mathematics, 59 in Economics and 55 in Statistics. Calculate weighted mean of the marks if weights are respectively 2, 1, 5, 5 and 3.
View Solution
Knowledge Check
A candidate obtains the marks in subjects, English 40, Hindi 30, Mathematics 60, Physics 70 and Chemistry 40. In the weights of 1, 2, 2, 3, 3 respectively are allotted to the subjects, then in the candidates weighted mean is
A59B615C10D50In an examination, a candidate scores the following percentage of marks. English-44, Hindi-58, Maths—74, Physics-61, Chemistry—62. If weights 2, 4, 4, 5, 3 respectively, are allotted to these subjects, then the candidate's weighted mean percentage is
A61B61.5C62D62.5A candidate obtained the following percentage of marks in an examination English 60, Maths 90, Physics 75, Chemistry 66. If weighs 2, 4, 3, 3 are allotted to these subjects respectively, then the weight mean is given by
A60+90+75+662+4+3+3B60×2+90×4+75×3+66×32+4+3+3C60×2+90×4+75×3+66×34D60+90+75+664
A student obtained 60 marks in English, 75 in Hindi, 63 in Mathematics, 59 in Economics and 55 in Statistics. Calculate weighted mean of the marks if weights are respectively 2, 1, 5, 5 and 3.
View SolutionA student obtained the following marks per centage in an examination English - 50, Accounts - 75, Economics - 60, B Std.-80, Hindi - 55. If weights are 2, 3, 3, 2, 1, respectively allotted to the subjects, his weighted mean is
View SolutionIn an examination, 60% of the candidates passed in English and 70% of the candidates passed in Mathematics, but 20% failed in both of these subjects. If 2500 candidates passed in both the subjects, the number of candidates that appeared at the examination was
View SolutionThe mean and standard deviation of marks obtained by 50 students of a class in three subjects, Mathematics, physics and Chemistry are given below
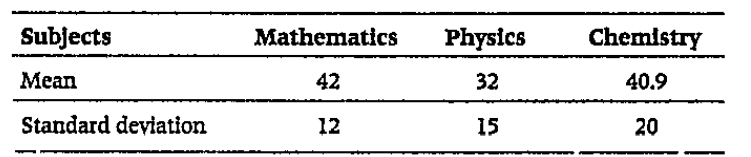
Which of these three subjects shows the highest variability in marks and which shows the lowest ?
View SolutionThe probabilities that a student passes in Mathematics, Physics and Chemistry are m, p and respectively. Of these subjects the student has 75% chance of passing in atleast one, a 50% chance of passing in atleast two and a 40% chance of passing in exactly, two, which of the following relations are true.
View Solution
