Show that ΔU=nCV(T2−T1) and ΔH=nCP(T2−T1)
For one mole of an ideal gas , we have
CV=dUdT
dU=CVdT
For a finite change , we have
ΔU=CVΔT
ΔU=CV(T2−T1)
and n moles of an ideal gas we get
ΔU=nCV(T2−T1) .....(1)
Similarly for n moles of an ideal gas we get
ΔH=nCP(T2−T1) ...(2)
Similar Questions
If K1 and K2 are equilibrium constants at T1 and T2 temperatures (T1>T2) ,then which of the following are correct?
(A) When Δ H=+ve,K2>K1
(B) When Δ H=0 K1=K2
(C) When Δ H=-ve K2<K1
(D) When Δ H=+ve K2<K1
View SolutionShow that the relation R defined in the set A of all triangles as R= {(T1,T2):T1 is similar to T2}, is equivalence relation. Consider three right angle triangles T1 with sides 3,4,5, T2 with sides 5,12,13 and T3 with sides 6,8,10. Which triangles among T1,T2 and T3 are related ?
View Solution
Knowledge Check
If ΔG=ΔH−TΔS and ΔG=ΔH+T[d(ΔG)dT]P then variation of EMF of cell , with temperature T, is given by
AΔSnFB−ΔSnFCΔHnFDΔGnF
p−V diagram of same mass of a gas are drawn at two different temperatures (T1) and (T2). Explain whether T1>T2orT2>T1.
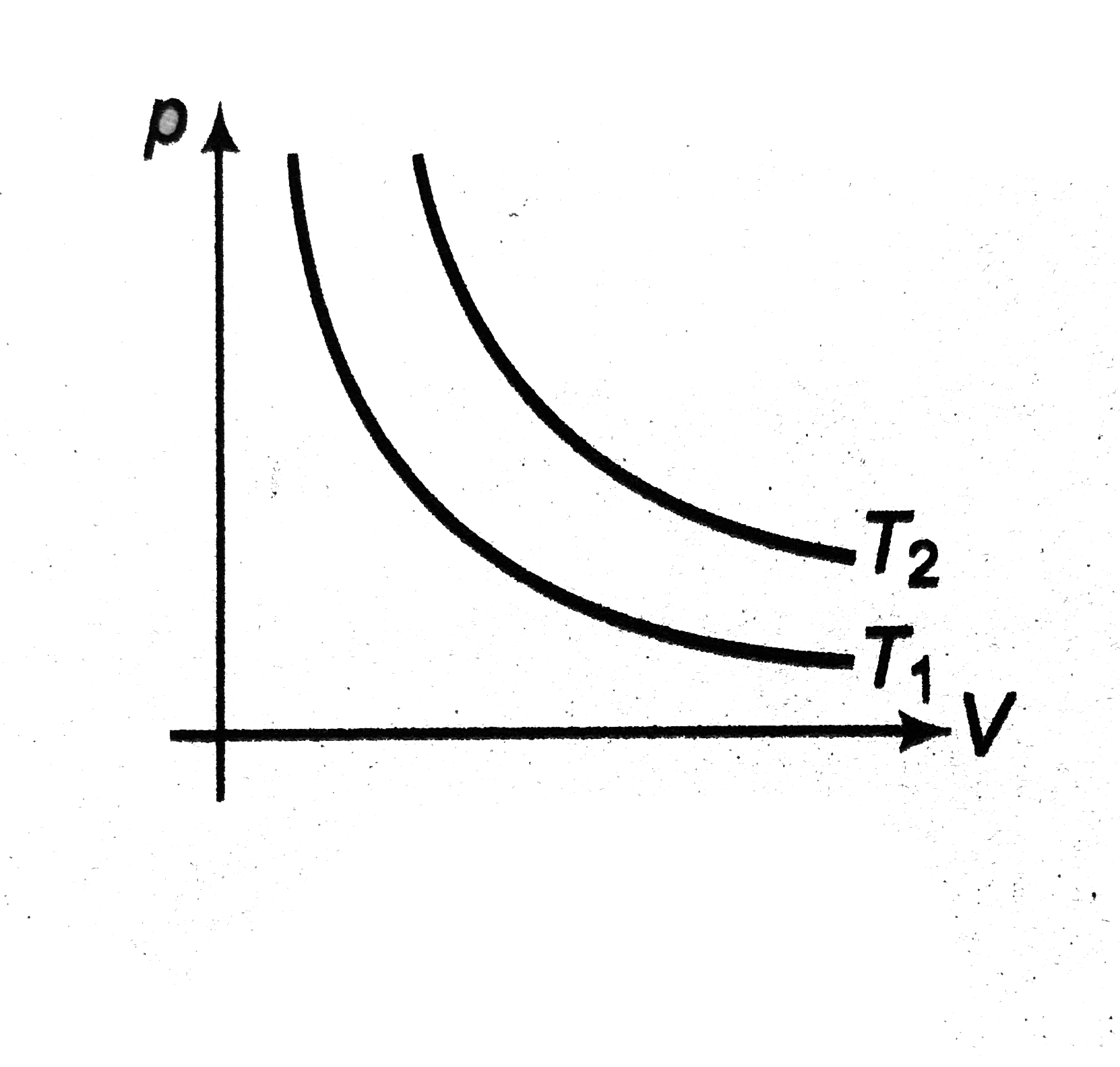 .
.
View SolutionShow that the relation R defined in the set A of all triangles as R={(T1,T2):T1 is similar to T2}, is equivalence relation.
View SolutionShow that the relation R defined in the set A of all triangles as R={(T1,T2):T1 is similar to T2}, is equivalence relation.
View SolutionNumber of correct statements are :
ΔU=nCVmΔT is applicable for ideal gas undergoing reversible process but not irreversibe (No chemical and phase change)
ΔS=nCVmΔT is applicable for ideal gas undergoing reversible process but not irreversible (No chemical and phase change)
ΔS=nCVmlnT2T1+nRln'V2V1 is applicable for ideal gas undrgoing reversible as well as irreversibel process (No chemical and phase change )
ΔH=ΔU+Δ(PV) isapplicable for chemical and phase change carried out reversibly or irreversibly
PVγ = constant for reversible and irreversible adiabatic process involving ideal gas.
View SolutionABCD is a square with side AB = 2. A point P moves such that its distance from A equals its distance from the line BD. The locus of P meets the line AC at T1 and the line through A parallel to BD at T2 and T3. The area of the triangle T1T2T3 is :
View SolutionABCD is a square with side AB = 2. A point P moves such that its distance from A equals its distance from the line BD. The locus of P meets the line AC at T1 and the line through A parallel to BD at T2 and T3. The area of the triangle T1T2T3 is :
View SolutionA body is released from rest at t0=0,x0=0 and moves with constant acceleration. Its position is measured at the equally spaced times t1=Δt,t2=2Δt,t3=3Δt and so forth. Prove that displacements Δxj−xj−1 which occur in the successive time intervals Δt are in the ratio
Δx1:Δx2:Δx3......≈1:3:5...
View Solution
