Consider a parallel plate capacitor whose plates are closely spaced. Let R be the radius of the plates and the current in the wire connected to the plates is 5A, calculate the displacement current through the surface passing between the plates by directly calculating the rate of change of flux of electric field through the surface.

Text Solution

Verified by Experts

Current flowing in the wire connected to the plates is $I_{c} = 5 A$ .
Let the radius of the plates be R.
Let the area of the plates be A.
Electric field between the plates
$E = \frac{Q}{ε_{0} A}$ Q - charge
The flux of this field through the given area is
$ϕ_{E} = \frac{ϕ}{ε_{0} A} \times A$
$= \frac{Q}{ε_{0}}$
$∴$ The displacement current
$I_{d} = ε_{0} \frac{d ϕ_{E}}{d t}$
$I_{d} = ε_{0} \frac{d}{d t} (\frac{Q}{ε_{0}})$
$= \frac{d Q}{d t}$
$I_{d} = I_{C}$ [since $\frac{d Q}{d t} = I_{C}$ ]
The current $I_{C}$ is equal to the rate of flow of charge to the positive state through the connecting wire.
$∴ I_{d} = I_{C} = 5 A$
$∴$ The displacement current = 5 A.

Updated on:21/07/2023

During charging of capacitor, $I_{C}$ . is the conduction current flowing in the wires connecting capacitor to the battery and $I_{d}$ is the Displacement current flowing between the plates of the capacitor.
A $I_{c} > I_{d}$
B $I_{c} < I_{d}$
C $I_{c} = I_{d}$
DNo relation exists between $i_{c}$ and $I_{d}$
There is one parallel plate capacitor with circular plates of radius R. Capacitor is being charged by connecting to a battery . If I is the instantaneous conduction current flowing in the connecting wires then what will be displacement current flowing in the region between the plates enclosed between radii, $R / 2$ to R
A $3 I / 4$
B $I$
C $I / 4$
D $3 I / 5$
An insulator plate is passed between the plates of a capacitor. Then current
.
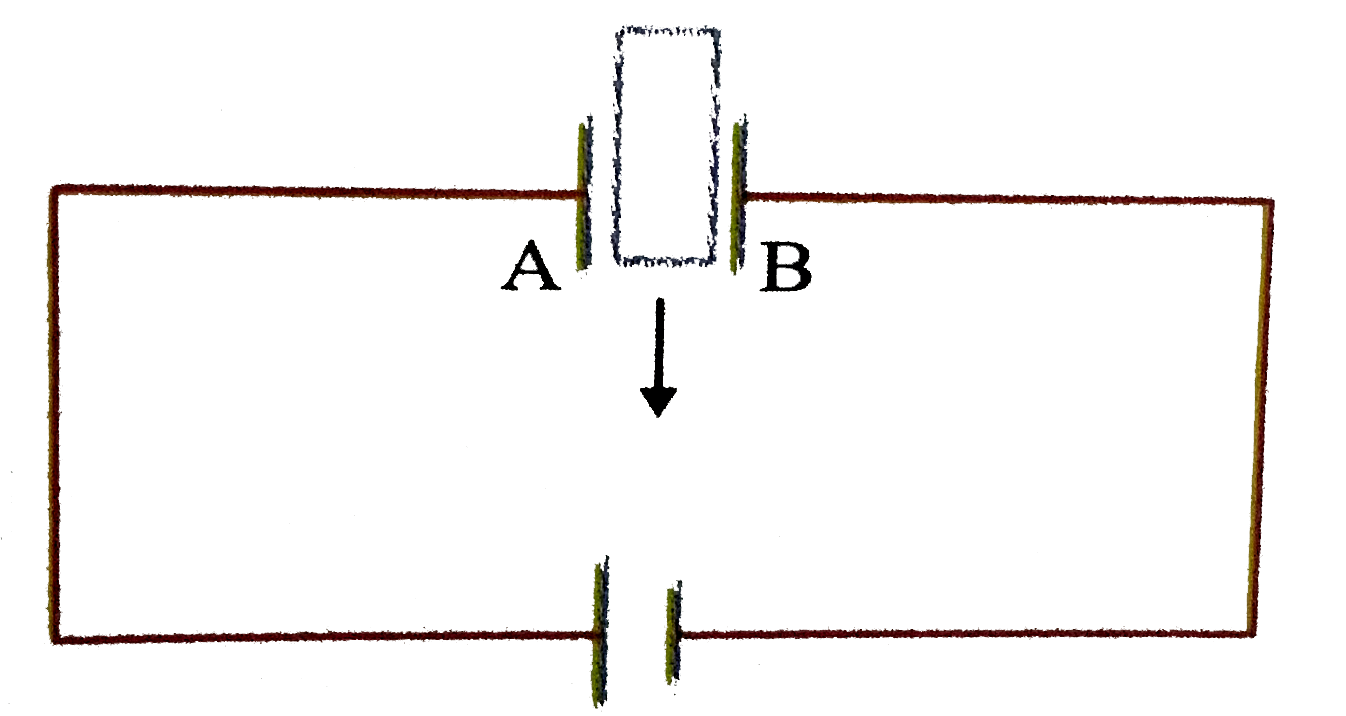Aalways flows from $A$ to $B$
Balways flows from $B$ to $A$
Cfirst flows from $A$ to $B$ and then from $B$ to $A$
Dfirst flows from $B$ and $A$ and then from $A$ to $B$