12 cells of each of emf 1.5 V and internal resistance 0.5Ω are arranged in m rows each containing n cells connected in series. Calculate the values of n and m for which this combination would send maximum current through an external resistance of 1.5Ω . What is the value of the maximum current?
According to the question
mn=12 ……(i)
Now , current along the external resistance R is maximum with .
R=nrm (r is the internal resistance )
⇒ 1.5=n×0.3m⇒n=3m
From Eqs. (i) and (ii) , we have
m = 2 , n = 6
Values of maxium current in the circuit.
I=nEnrm+R=6×1.56×0.52+1.5=6×1.52(1.5)=3A
Similar Questions
12 cells, each of emf 1.5 V and internal resistance of 0.5Ω, are arranged in m rows each containing n cells connected in series, as shown. Calculate the values of n and m for which this combination would send maximum current through an external resistance of 1.5Ω.
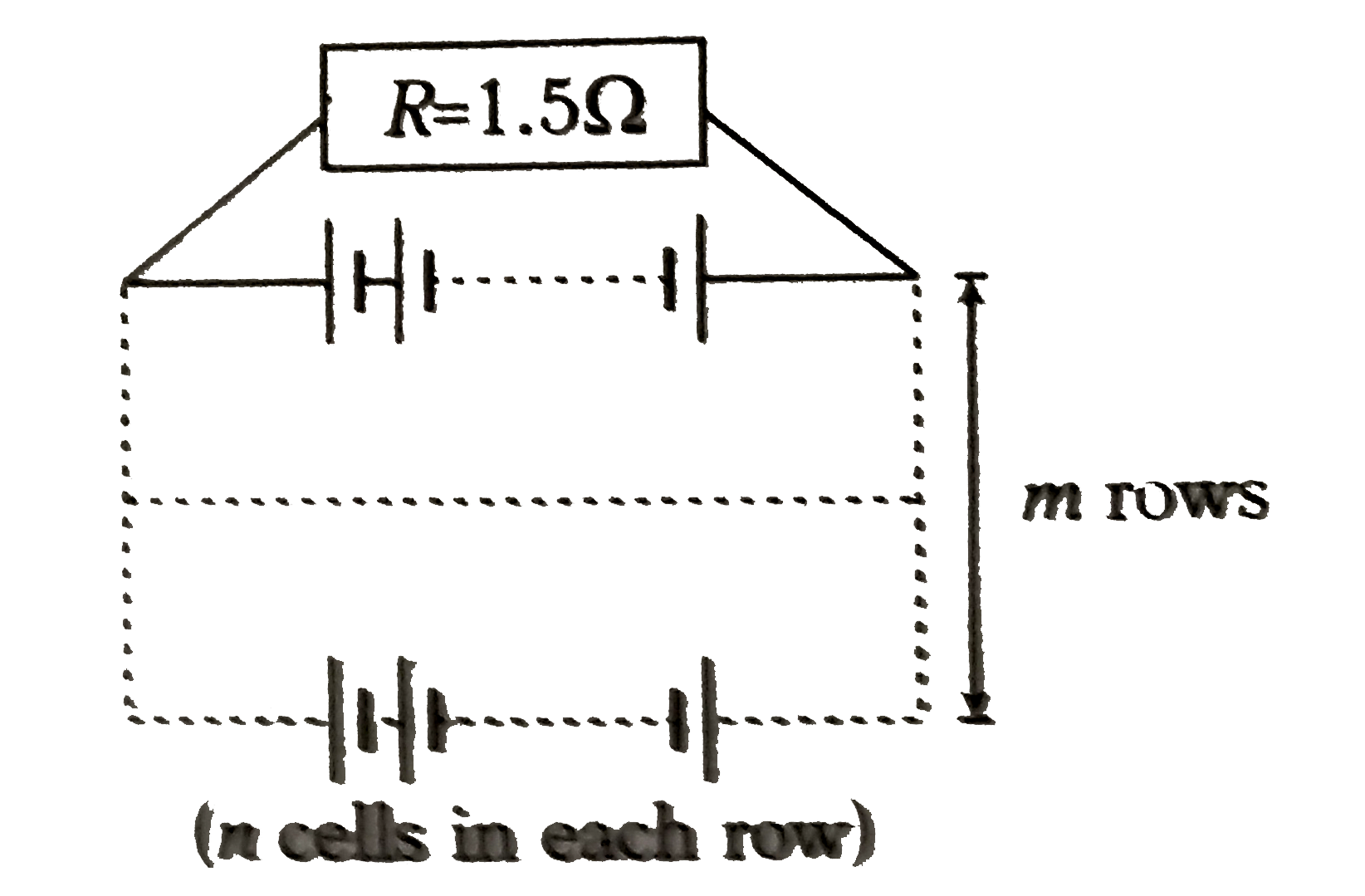
View Solution12 cells, each of emf 1.5 V and internal resistance of 0.5Ω, are arranged in m rows each containing n cells connected in series, as shown. Calculate the values of n and m for which this combination would send maximum current through an external resistance of 1.5Ω.

View Solution
Knowledge Check
24 identical cells, each of internal resistance 0.5Ω, are arranged in a parallel combination of n rows, each row containing m cells in series. The combination is connected across a resistor of 3Ω. In order to send maximum current through the resistor, we should have
Am=12, n=2Bm=8, n=3Cm=2, n=2Dm=3, n=8Two cells of e.m.f. 2.5 V and 2.0 V having internal resistance of 1Ω and 2Ω respectively are connected in parallel with similar poles connected together so as to send the current in the same direction through an external resistance of 2Ω. The current in the external resistance is
A0.87 AB1.29 AC1.00 AD2.29 A24 cells of emf 1.5V each having internal resistance of 1Ω are connected to an external resistance of 1.5Ω. To get maximum current,
Aall cells are connected in series combinationBall cells are connected in parallel combinationC4 cells in each row are connected in series and 6 such rows are connected in parallelD6 cells in each row are connected in series and 4 such rows are connected in parallel
24 cells, each of emf 1.5V and internal resistance is 2Ω connected to 12Ω series external resistance. Then,
View SolutionArrange 32 identical cells of emf 2 V each with internal resistance 0.5Ω such current passing through an external resistance of 1Ω is maximum.
what will be the value of current ?
View SolutionHow would you arrange 64 similar cells each having an emf of 2.0 V and internal resistance 2Ω so as to send maximum current through an external resistance of 8Ω.
View SolutionThirty six cells each of emf 1.5 V and internal resistance 0.5Ω are used to send current through an external resistor of resistance 2Ω. What is the best mode of grouping them and the current through the external resistor
View SolutionTwo cells of 1.5 V and 2 V , having internal resistances of 1Ω and 2Ω respectively, are connected in parallel so as to read the current in the same direction through an external resistance of 5Ω . The current in the external resistance will be
View Solution
