42 cubes each of side 1 cm area glured together to form a solid cuboid . If the perimeter of the base of the cuboid is 18 cm, then it height (in cm) is
3
4
1
2
The correct Answer is:A
To solve the problem, we need to determine the height of a cuboid formed by gluing together 42 cubes, each with a side length of 1 cm. We are also given that the perimeter of the base of the cuboid is 18 cm.
1. Calculate the Volume of the Cuboid:
Each cube has a volume of 1cm3 (since volume = side³). Therefore, the total volume of 42 cubes is:
Total Volume=42×13=42cm3
2. Understand the Perimeter of the Base:
The perimeter P of a rectangle (base of the cuboid) is given by the formula:
P=2×(length+width)
Given that the perimeter is 18 cm, we can set up the equation:
2×(l+w)=18
Simplifying this gives:
l+w=9(Equation 1)
3. Express the Volume of the Cuboid:
The volume V of the cuboid can also be expressed as:
V=l×w×h
We know the volume is 42 cm³, so:
l×w×h=42(Equation 2)
4. Substituting for Height:
From Equation 1, we can express w in terms of l:
w=9−l
Substituting this into Equation 2 gives:
l×(9−l)×h=42
This simplifies to:
(9l−l2)×h=42
5. Finding Possible Dimensions:
To find suitable dimensions, we can try different integer values for l and w such that l+w=9 and both l and w are integers. The pairs are:
- l=1,w=8
- l=2,w=7
- l=3,w=6
- l=4,w=5
6. Calculating Height for Each Pair:
For each pair, we can calculate h:
- For l=1,w=8:
1×8×h=42⟹h=428=5.25(not an integer)
- For l=2,w=7:
2×7×h=42⟹h=4214=3(valid)
- For l=3,w=6:
3×6×h=42⟹h=4218=2.33(not an integer)
- For l=4,w=5:
4×5×h=42⟹h=4220=2.1(not an integer)
7. Conclusion:
The only valid integer height is when l=2 and w=7, giving us:
h=3cm
Final Answer:
The height of the cuboid is 3cm.
Each cube has a volume of 1cm3 (since volume = side³). Therefore, the total volume of 42 cubes is:
Total Volume=42×13=42cm3
The perimeter P of a rectangle (base of the cuboid) is given by the formula:
P=2×(length+width)
Given that the perimeter is 18 cm, we can set up the equation:
2×(l+w)=18
Simplifying this gives:
l+w=9(Equation 1)
The volume V of the cuboid can also be expressed as:
V=l×w×h
We know the volume is 42 cm³, so:
l×w×h=42(Equation 2)
From Equation 1, we can express w in terms of l:
w=9−l
Substituting this into Equation 2 gives:
l×(9−l)×h=42
This simplifies to:
(9l−l2)×h=42
To find suitable dimensions, we can try different integer values for l and w such that l+w=9 and both l and w are integers. The pairs are:
- l=1,w=8
- l=2,w=7
- l=3,w=6
- l=4,w=5
For each pair, we can calculate h:
- For l=1,w=8:
1×8×h=42⟹h=428=5.25(not an integer)
- For l=2,w=7:
2×7×h=42⟹h=4214=3(valid)
- For l=3,w=6:
3×6×h=42⟹h=4218=2.33(not an integer)
- For l=4,w=5:
4×5×h=42⟹h=4220=2.1(not an integer)
The only valid integer height is when l=2 and w=7, giving us:
h=3cm
The height of the cuboid is 3cm.
Topper's Solved these Questions
- BOOK - ARIHANT PUBLICATION PUNJABCHAPTER - MENSURATIONEXERCISE - Chapter Exercise83videos
MENSURATION
- BOOK - ARIHANT PUBLICATION PUNJABCHAPTER - MEASUREMENT SYSTEMEXERCISE - CHAPTER EXERCISE111videos
MEASUREMENT SYSTEM
- BOOK - ARIHANT PUBLICATION PUNJABCHAPTER - NUMBER SYSTEMEXERCISE - Example33videos
NUMBER SYSTEM
Similar Questions
Two cubes each of volume 27cm3 are joined end to end to form a solid. Find the surface area of the resulting cuboid.
View SolutionThree gold cubes each of 3cm length are melted and gold cuboid is formed from it.The area of the base of the cuboid is 27cm2. What is the height of the cuboid?
View Solution
Knowledge Check
- Question 1 - Select One
42 cubes each of side 1 cm area glured together to form a solid cuboid . If the perimeter of the base of the cuboid is 18 cm, then it height (in cm) is
A3B4C1D2 - Question 2 - Select One
Forty - two cubes each of side 1 cm are glued together to form a solid cuboid. If the perimeter of the base of the cuboid is 18 cm then its height, in cm, is
A1B2C3D4 - Question 3 - Select One
The solid cuboid is formed by mutual joining of 24 cubes of 1cm side. If perimeter of the base of this cuboid is 12cm, then height of cuboid will be
A1cmB2cmC3cmD4cm
Two cubes of edge 10 m each joined together to form a single cuboid. The ratio of the total surface area of one of the cubes and that of the single cuboid formed is ______.
View SolutionTwo cubes of edge 10 m each joined together to form a single cuboid. The ratio of the total surface area of one of the cubes and that of the single cuboid formed is ______.
View SolutionIf the volume of a cuboid is 440 cm3, the area of its base is 88 cm2 , then its height is
View SolutionThree cubes each of edge 3 cm long are placed together as shown in the adjoining figure . Find the surface area of the cuboid so formed :
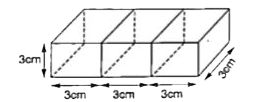 View Solution
View SolutionIf the volume of a cuboid is 440cm3, the area of its base is 88cm2, then its height is
View Solution

