In the given body- centred cubic AB, AC, and AA' are :
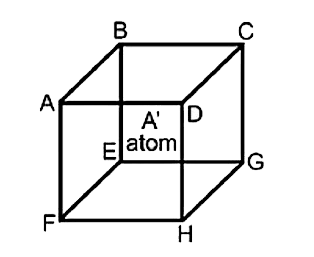
A
a,√2a,√3a2
B
a,√3a2,√2a
C
√3a2,√2a,a
D
a,a√2,√3a2
Text Solution
The correct Answer is:A
Edge length
∴AC=√(AB)2+(BC)2=√2a
Now, AA. + A.G = 2 AA. = √3a
∴ AA. =√3a2
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
In body-centred cubic lattice given below, the three disntances AB,AC, and ∀' are
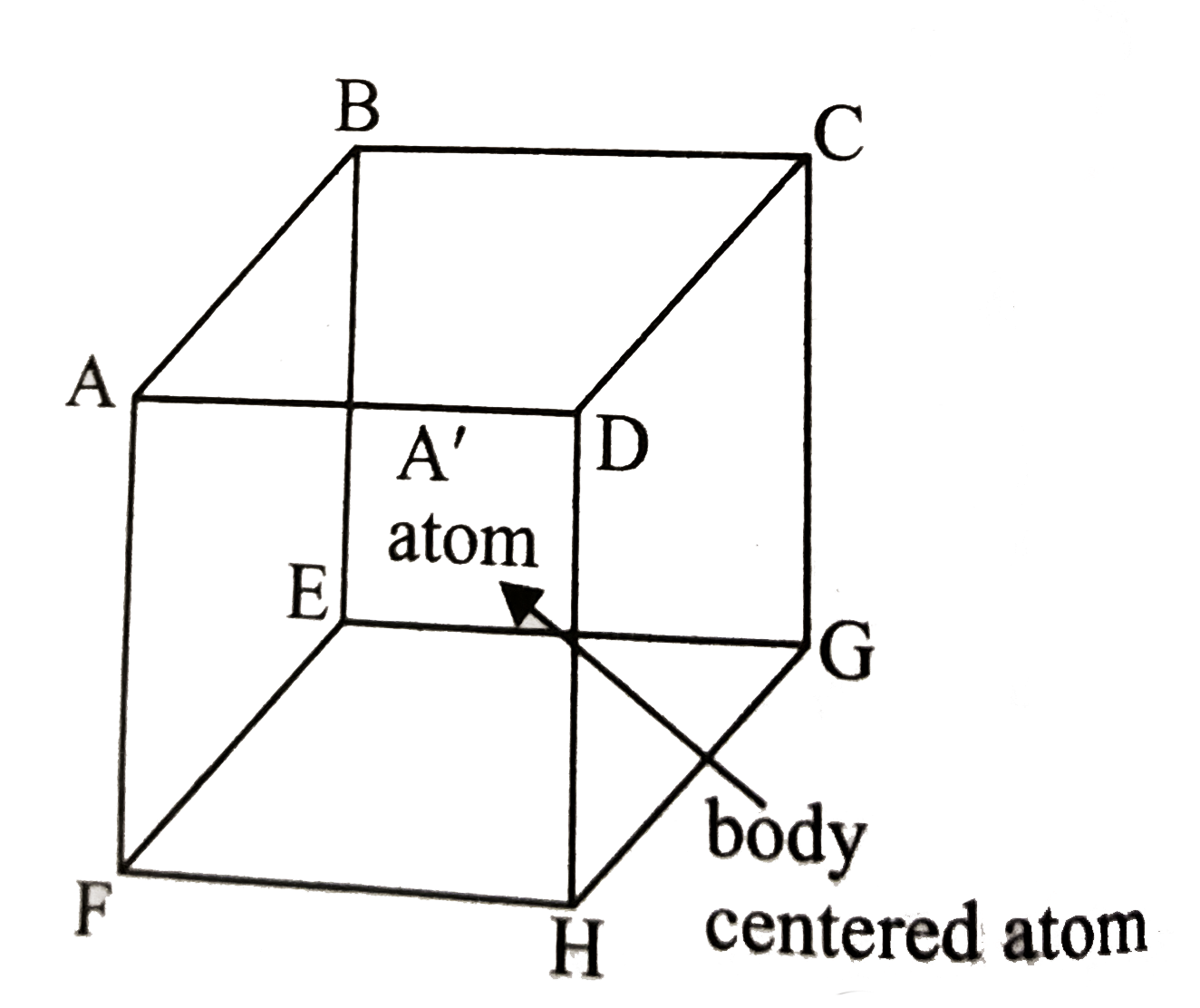 Aa,√2a,√3a2Ba,√3a2,√2aC√3a2,√2a,aDa,a√2a,√3a2
Aa,√2a,√3a2Ba,√3a2,√2aC√3a2,√2a,aDa,a√2a,√3a2In body-centered cubic lattice given below, the three distances AB, AC and AA' are
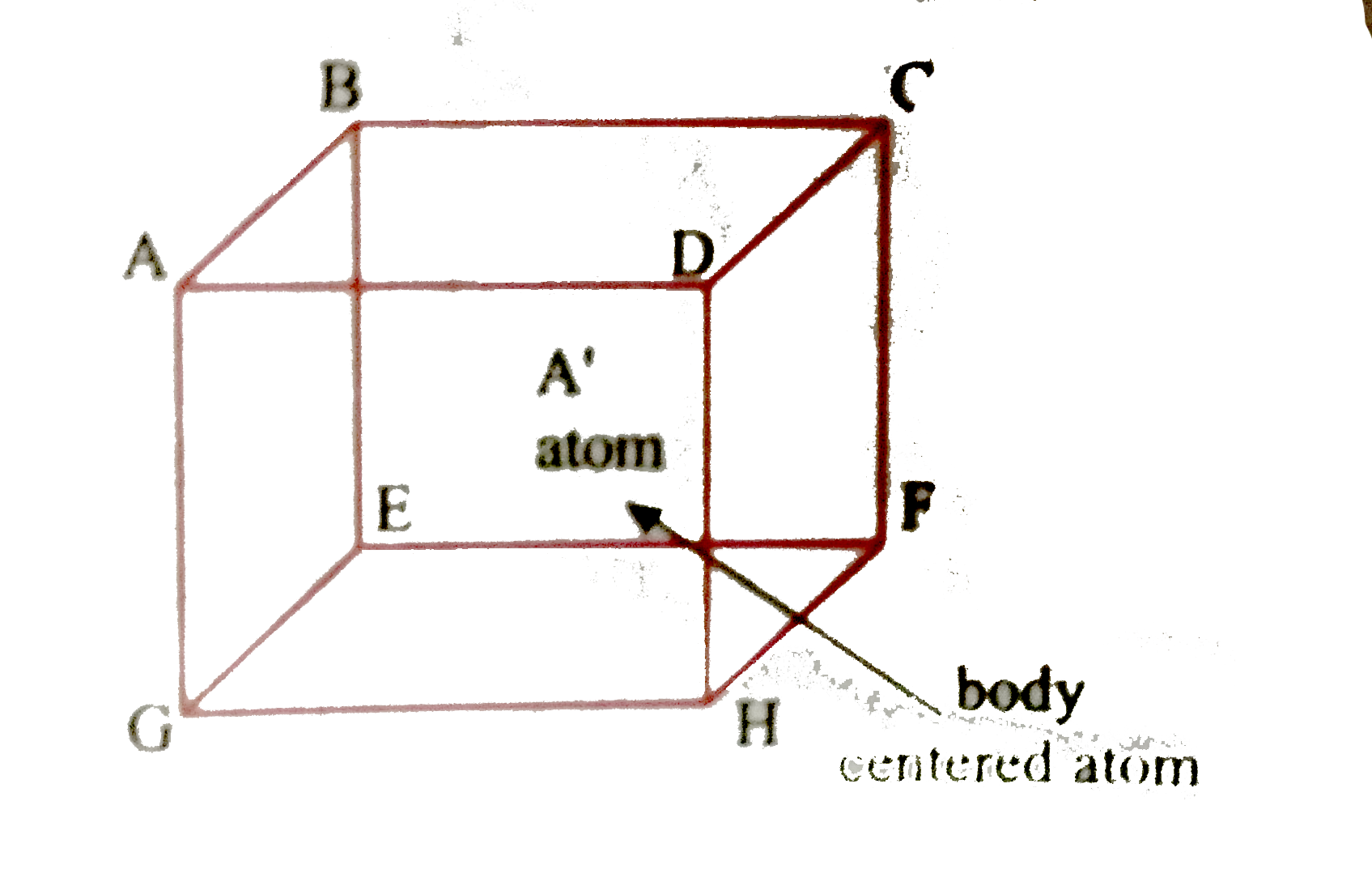 Aa,√2a,√3a2Ba,√3a2,√2aC√3a2,√2a,aDa,a√2,√3a2
Aa,√2a,√3a2Ba,√3a2,√2aC√3a2,√2a,aDa,a√2,√3a2In body-centred cubic lattice given below, the three disntances AB,AC, and A' are
 Aa,√2a,√32aBa,√32a,√2aC√32a,√2a,aDa,a√2,√32a
Aa,√2a,√32aBa,√32a,√2aC√32a,√2a,aDa,a√2,√32a
The coordination number of each atom in body centered cubic unit cell is
View SolutionIn a body centred cubic cell an atom at the body of centre is shared by-
View SolutionIn a simple cubic, body-centred cubic and face-centred cubic structure, the ratio of the number of atoms present is respectively
View SolutionPercentage of free space in cubic in a body- centred cubic unit cell is .
View SolutionIf a stands for the edge length of the cubic system : simple cubic, body − centred cubic anf face − centred cubic, then the ratio of radii of the spheres in these systems will be respectively:
View Solution
