A cuboid of dimensions (6 cm × 4 cm × 1 cm) is painted black on both the surfaces of dimensions (4 cm × 1cm), green on the surfaces of dimensions (6 cm × 4 cm) and red on the surfaces of dimen~1ons (6cm×1cm). Now, the block is divided into various smaller cubes of side 1 cm each. The smaller cubes, so obtained are separated.
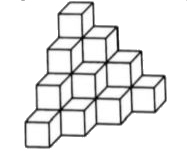
How many cubes are there in the group?
10
15
18
20
The correct Answer is:D
From the top most row of the figure
Cube in 1st row= 1
Cube in 2nd row = 3
Cube in 3rd row = 6
Cube in 4th row = 10
∴Total number of cubes = 1 + 3+ G+ 10 = 20
Topper's Solved these Questions
- CUBE AND DICEBOOK - ARIHANT PUBLICATIONCHAPTER - CUBE AND DICEEXERCISE - LET US PRACTICE (A. BASE LEVEL EXERCISE )
23 Videos
- COUNTING FIGURESBOOK - ARIHANT PUBLICATIONCHAPTER - COUNTING FIGURESEXERCISE - Let us Practice (Expert Level Exercise)
9 Videos
- DATA SUFFICIENCY BOOK - ARIHANT PUBLICATIONCHAPTER - DATA SUFFICIENCY EXERCISE - LET US PRACTICE (B. EXPERT LEVEL EXERCISE)
11 Videos
Similar Questions
Knowledge Check
A cuboid of dimensions (6 cm × 4 cm × 1 cm) is painted black on both the surfaces of dimensions (4 cm × 1cm), green on the surfaces of dimensions (6 cm × 4 cm) and red on the surfaces of dimensions (6cm×1cm). Now, the block is divided into various smaller cubes of side 1 cm each. The smaller cubes, so obtained are separated.
How many cubes are there in this diagram?
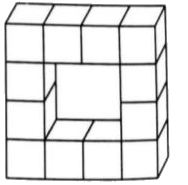 A1. 16B2. 12C3. 10D4. 8
A1. 16B2. 12C3. 10D4. 8A cuboid of dimensions (6 cm × 4 cm × 1 cm) is painted black on both the surfaces of dimensions (4 cm × 1cm), green on the surfaces of dimensions (6 cm × 4 cm) and red on the surfaces of dimen~1ons (6cm×1cm). Now, the block is divided into various smaller cubes of side 1 cm each. The smaller cubes, so obtained are separated.
How many cubes will have all three colours black, green and red each atlest on one side ?A16B12C10D8
Find the volume of cuboid of dimensions 5cm, 4cm and 3cm.
View SolutionRead the given information carefully and answer the questions that follow
All the surfaces of a cube of side 15 cm are painted with red colour and then it is cut into smaller cubes of side 3 cm. The smaller cubes so obtained are separated.
How many smaller cubes are there having two surfaces painted with red colour?
View SolutionRead the given information carefully and answer the questions that follow
All the surfaces of a cube of side 15 cm are painted with red colour and then it is cut into smaller cubes of side 3 cm. The smaller cubes so obtained are separated.
How many smaller cubes are there having only three surfaces painted with red colour?
View SolutionRead the given information carefully and answer the questions that follow
All the surfaces of a cube of side 15 cm are painted with red colour and then it is cut into smaller cubes of side 3 cm. The smaller cubes so obtained are separated.
How many smaller cubes are there having 4 or more faces painted with red colour?
View SolutionOne metal solid of dimensions 16cm×8cm×cm and another solid of the same metal having dimensions 12cm×6cm×5 cm are melted to from a cube . Find the edge of the cube
View SolutionThe dimensions of a cuboid are 15 cm × 12 cm × 10 cm . What is its total surface area ?
View Solution
