Point P is the midpoint of seg CD. If CP = 2.5, find l(CD).
The correct Answer is:5
Similar Questions
Knowledge Check
In the figure below, ABCD is a rectangle, EFGH is a square, and ¯¯¯¯¯¯CD is a diameter of a semicircle. Point K is the midpoint of ¯¯¯¯¯¯CD. Point J is the midpoint of both ¯¯¯¯¯¯AB and ¯¯¯¯¯¯EF. Points E and F lie on ¯¯¯¯¯¯AB. The 3 given lengths are in meters.
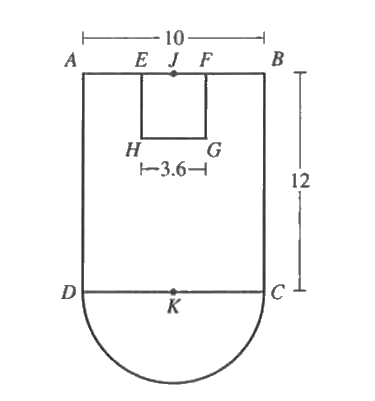
What is the length, in meters, of are ¯¯¯¯¯¯CD?A2.5πB5πC6.25πD10πP(−1,2) is the midpoint of seg AB. If B(4,3), what are the co-ordinates of the point A ?
A(6,1)B(1,6)C(−1,6)D(−6,1)
.Point M is the midpoint of Seg AB.If AB=8 then find the length of AM.
View Solution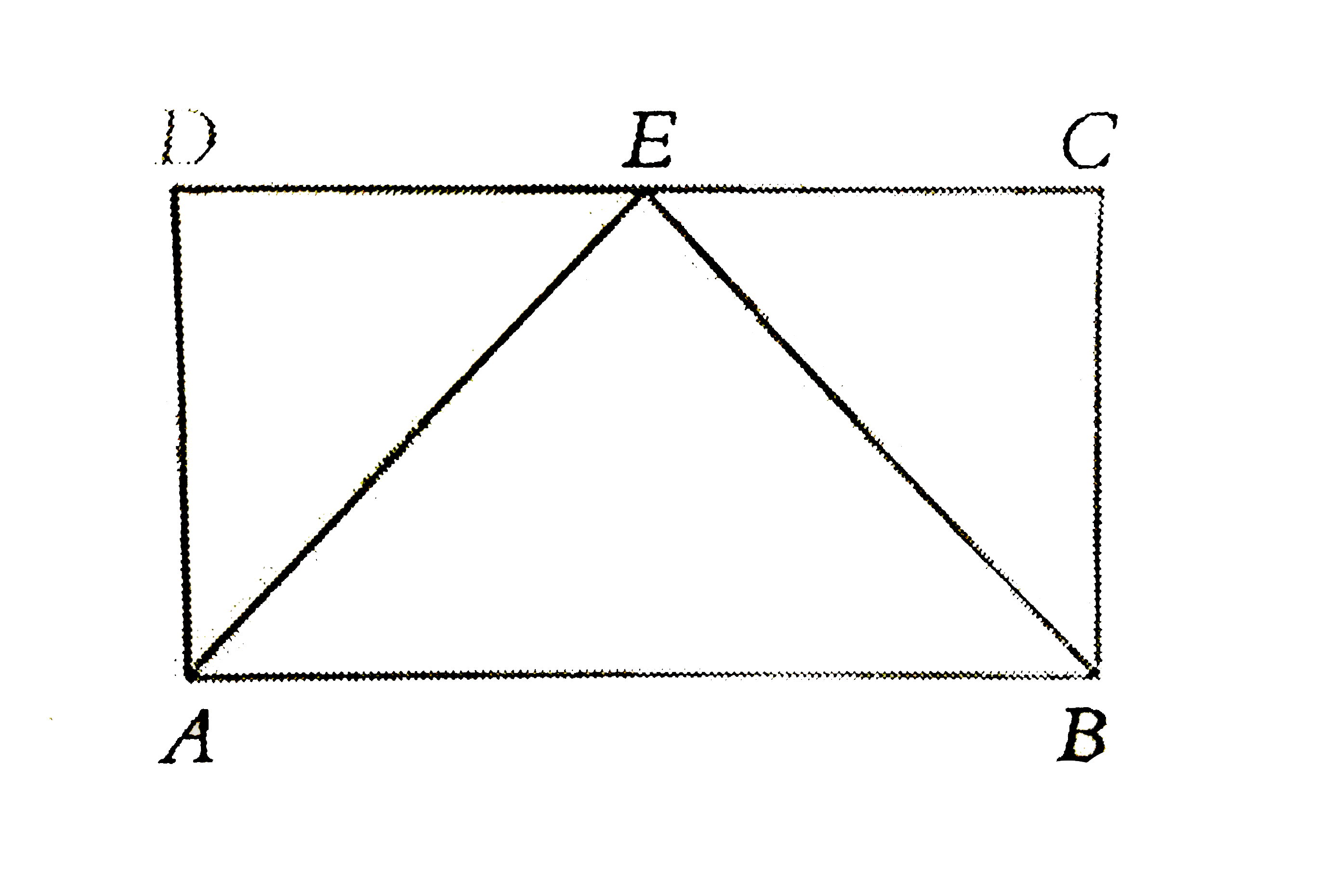
In the figure above ( not to scale ), ABCD is a rectangle, E is the mid - point of CD. If CD=24 cm and AD=5 cm, then find the perimeter of ΔABE.
View SolutionIn ΔLMN, D is the midpoint of seg MN. If L≡(2,4),D≡(2,−2), find the coordinates of the point G which divides the median internally in the ratio 2:1
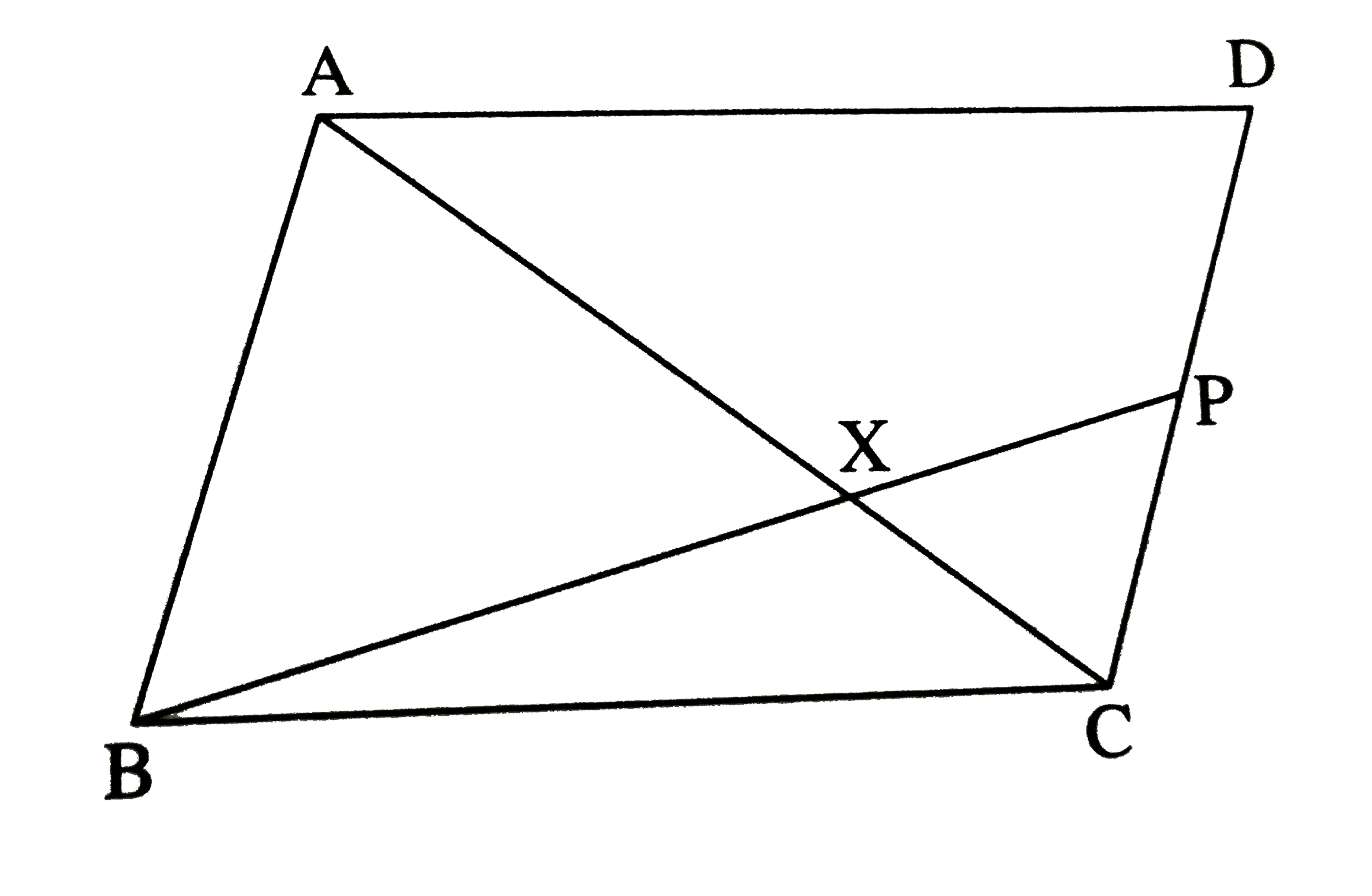
View Solution□ABCD is a parallelogram. P is the midpoint of side CD. Seg BP meets diagonal AC at X. Prove that 3AX=2AC.
View Solution
In the figure above ( not to scale ), ABCD is a rectangle, E is the mid - point of CD. If CD=24 cm and AD=5 cm, then find the perimeter of ΔABE.
View SolutionAB and CD are two chords of a circle intersecting at a point P inside the circle. If
AP=3cm, PB= 2.5cm and CD= 6.5 cm, determine CP.
View Solution
