Sum of the roots of a quadratic equation is double their product.
Find k if equation is x2−4kx+k+3=0.Solution in Marathi
The correct Answer is:3
Step by step video, text & image solution for Sum of the roots of a quadratic equation is double their product. Find k if equation is x^(2)-4kx+k+3=0. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - BAL BHARTICHAPTER - QUADRATIC EQUATIONSEXERCISE - Practice Set 2.68videos
QUADRATIC EQUATIONS
- BOOK - BAL BHARTICHAPTER - QUADRATIC EQUATIONSEXERCISE - Problem Set - 2 (Choose the correct answers)8videos
QUADRATIC EQUATIONS
- BOOK - BAL BHARTICHAPTER - QUADRATIC EQUATIONSEXERCISE - Practice Set 2.410videos
QUADRATIC EQUATIONS
- BOOK - BAL BHARTICHAPTER - PYTHAGORAS THEOREM EXERCISE - PRACTICE SET 227videos
PYTHAGORAS THEOREM
- BOOK - BAL BHARTICHAPTER - SIMILARITY EXERCISE - PROBLEM SET 112videos
SIMILARITY
Similar Questions
Product of Pragati's age 2 years ago and 3 years hence is 84. Find her present age.
03:34View SolutionWhich one is the quadratic equation?
01:33View SolutionCompare the given quadratic equation to the general form and write values of a, b, c: x2−7x+5=0
01:45View SolutionWhich of the following is a Pythagorean triplet?
02:09View SolutionIn ΔABCandΔPQR, in a one to one correspondence.
ABQR=BCPR=CAPQ, then
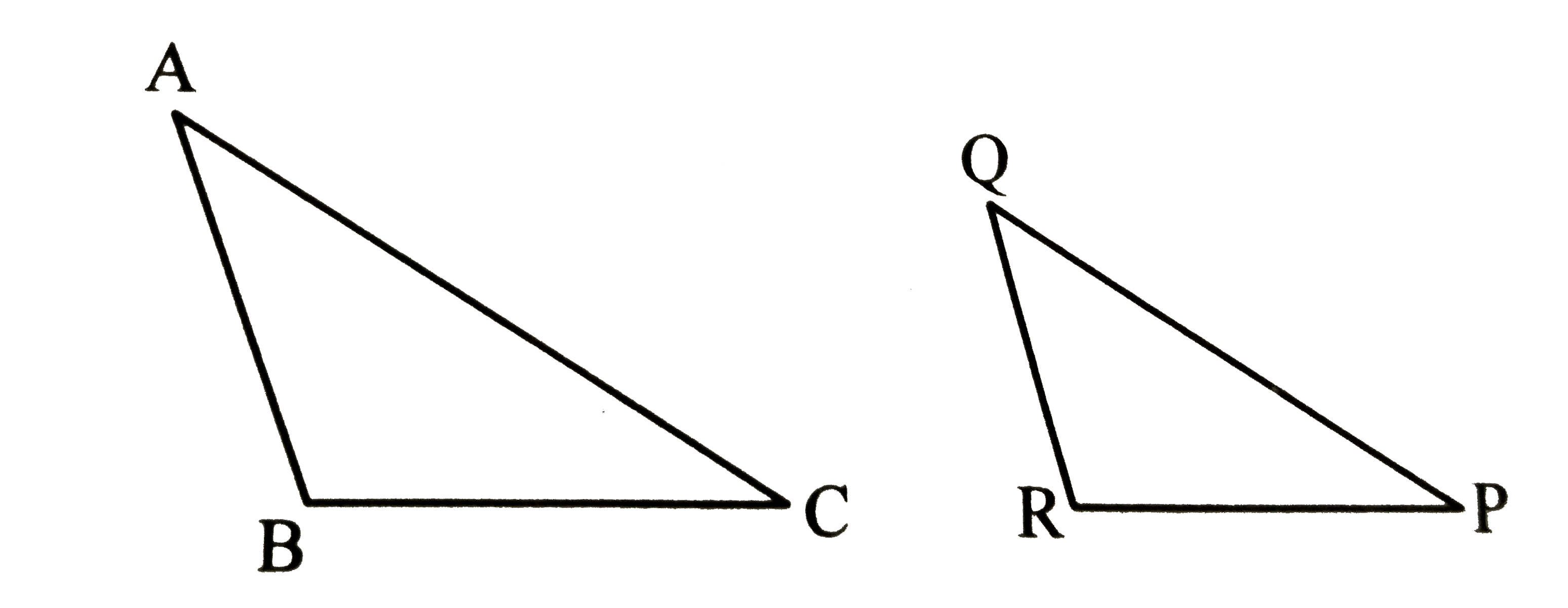02:01View SolutionFind the values of k for which the roots are real and equal in the following equations: kx(x−2)+6=0 (ii) x2−4kx+k=0
02:03View SolutionFind k if the sum of the roots of the quadratic equation 4x^(2)+8kx+k+9=0 is equal to their product.
02:13View SolutionFind the value(s) of k so that,the quadratic equation x^(2)-4kx+k=0 has equal roots.
04:35View Solutionनिम्न प्रत्येक द्विघात समीकरण में k के ऐसे मान ज्ञात कीजिए की उसके मूल वास्तविक तथा बराबर हों :
12x2+4kx+3=002:44View SolutionSum of the roots of a quadratic equation is double their product. Find k if equation is x2−4kx+k+3=0.
03:08View Solutionk का मान ज्ञात कीजिये यदि द्विघात समीकरण x2−4kx+k=0 के समान मूल हैं।
02:15View SolutionIf the sum of the roots and product of the roots of the quadratic equation kx2+2x+3k=0(k≠0) are equal, then find the value of k.
03:22View SolutionIF the product of the roots of the equation x2+4kx+12e3logk−I=0,(K>0) is 323, then the sum of its roots is
05:58View SolutionFind the value of k so that the sum of the roots of the quadratic equation is equal to the product to the roots :
(k+1)x2+2kx+4=004:17View SolutionFind the value of k such that the sum of the squares of the roots of the quadratic equation x2−8x+k=0 is 40:
02:44View SolutionSum of the roots of quadratic equation x2−4kx+k+3=0 is double their product. Find k
03:08View Solution