A factory produced two types of chemicals A and B. The following table gives the units of ingredients P and Q (per kg) of chemicals A and B as well as minimum requirements of P and Q and also cost per kg. chemicals A and B :
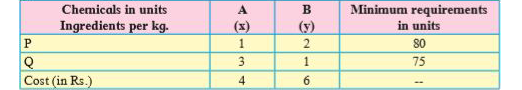
Find the number of units of chemicals A and B should be produced sp as to minimize the cost.
The correct Answer is:x = 14, y = 33
Similar Questions
A farm is engaged in breeding hens. The hens are fed products A and B grown in the farm which contains nutrients P, Q and R. One kilogram of product A contains 36 units, 3 units and 20 units of nutrients P Q and R respectively, whereas one kilogram of product B contains 6 units, 12 units and 10 units of nutrients P, Q and R respectively. The minimum requirement of nutrients P Q and R for a hen is 108, 36 and 100 units respectively. Product A costs 20 per kilogram and product B costs 40 per kilogram. Using linear programming, find the number of kilograms of products A and B to be produced to minimize the total cost. Identify the feasible region from the rough sketch.
View SolutionA company manufactures two types of chemicals A and B. Each chemical requires the types of raw materials P and Q. The table below shows number of units of P and Q required to manufacture one unit of A and one unit of B and the total availability of P and Q.
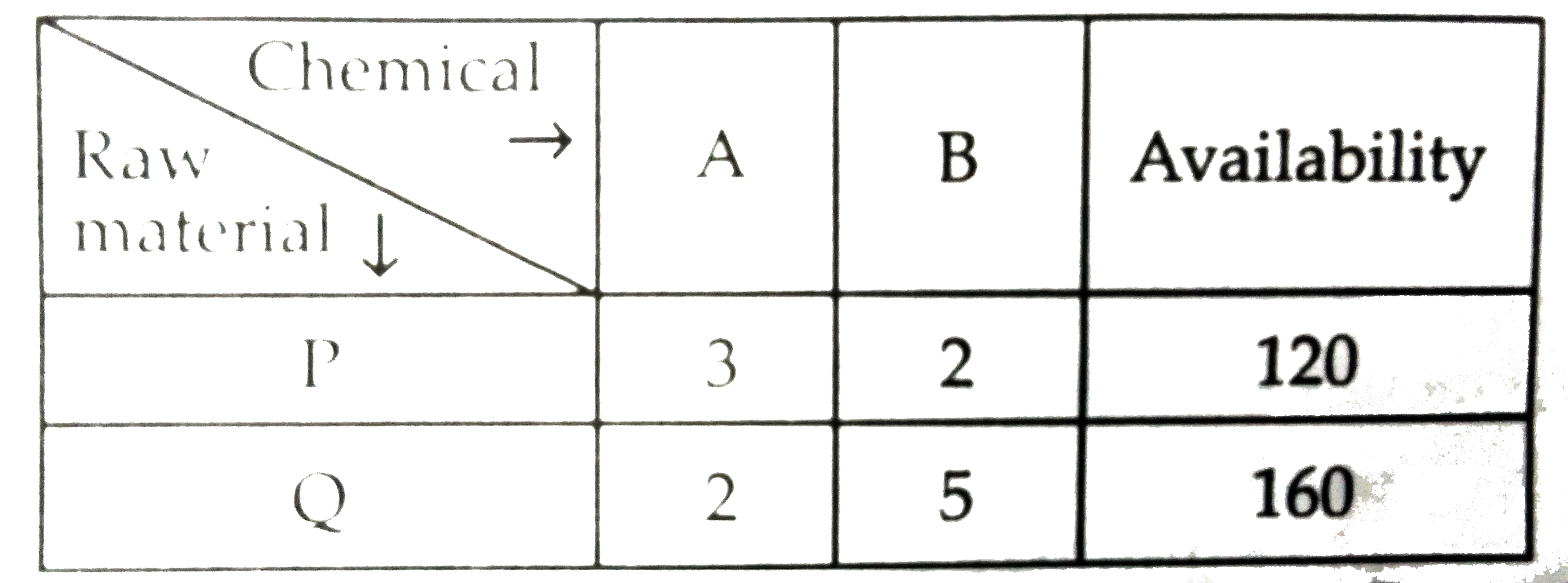
The company gets profit of Rs. 350 and RS.400 by selling one unit of A and one unit of B respectively. If the entire production of A and B is sold, then formulate the problem as LPP.
View Solution
Knowledge Check
Two different kinds of food A and B are being considered to form a weekly diet . The minimum weekly requirements for fats , carbohydrates and protein are 18, 24 and 16 units respectively . One kg of food A has 4 , 16 , and 8 units respectively of these ingredients and one kg of food B has 12 ,4 and 6 units respectively The price of food A is Rs 6 per kg and that of food B is Rs 5 per kg. How many kg of each type of food should he buy per week to minimize the cost and meet his requirements . Formulate this LPP
Az = 15 x+22y, subject to contraints 4x+12y≥18,16x+4y≥24,8x+6y>16,x≥0y≥0Bz = 15x +22y, subject to constraints 4x+12y≥18,16x+4y≥24,8x+6y>16,x≥0y≥0Cz=15x+48y , subject to constraints 4x+12y≥18,17x+4y≥24,8x+6y>160,x≥0y≥0Dz = 15x +22y, subject to constraints 24x+12y≥18,18x+4y≥24,8x+16y>16,x≥0y≥0Food X contains 4 units of vitamin A per gram and 7 units of vitamin B per gram and cost 15 paise per gram . Food Y contains 6 units of vitamin A per gram and 11 units of vitamin B per gram and cost 22 paise per gram . The daily minimum requirement of vitamin A and B are 90 units and 130 units respectively . The formulation of LPP to minimize the cost is
Az = 15x +22y, subject to constraints 4x+6y≥90,7x+11y≥130,x≥0y≥0Bz = 6x +5y, subject to constraints 4x+3y≥90,7x+11y≥130,x≥0y≥0Cz = 15x +62y, subject to constraints 4x+6y≥90,7x+11y≥450,x≥0y≥0Dz = 15x +22y, subject to constraints 4x+6y≥90,7x+45y≥260,x≥0y≥0
A company produces two types of good, A and B, that require gold and silver. Each unit of type A requires 3 gm of silver and 1 gm of gold while that of type B requires 1 gm of silver and 2 gm of gold. The company can produce 9 gm of silver and 8 gm of gold. If each unit of type A brings a profit of Rs. 40 and that of type B Rs. 50, find the number of units of each type that the company should produce to maximize the profit. What is the maximum profit?
View SolutionA farmer mixes two brands P and Q of cattle feed. Brand P, costing Rs 250 per bag, contains 3 units of nutritional element A, 2.5 units of element B and 2 units of element C. Brand Q costing Rs 200 per bag contains 1.5 units of nutritional element A, 11.25 units of element B, and 3 units of element C. The minimum requirements of nutrients A, B and C are 18 units, 45 units and 24 units respectively. Determine the number of bags of each brand which should be mixed in order to produce a mixture having a minimum cost per bag? What is the minimum cost of the mixture per bag?
View SolutionA farmer mixes two brands P and Q of cattle feed. Brand P costing Rs. 250 per bag, contains 3 units of nutritional element A, 2.5 units of element B and 2 units of element C. Brand Q costing Rs. 200 per bag contains 1.5 units of nutritonal element A, 11.25 units of element B and 3 units of element C. The minimum requirements of nutrients A,B and C are 18 units, 45 units and 24 units respectively. Determine the number of bags of each brand which should be mixed in order to produce a mixture having a minimum cost per bag? What is the minimum cost of the mixture per bag?
View SolutionA farmer mixes two brands P and Q of cattle feed. Brand P costing Rs. 250 per bag, contains 3 units of nutritional element A, 2.5 units of element B and 2 units of element C. Brand Q costing Rs. 200 per bag contains 1.5 units of nutritonal element A, 11.25 units of element B and 3 units of element C. The minimum requirements of nutrients A,B and C are 18 units, 45 units and 24 units respectively. Determine the number of bags of each brand which should be mixed in order to produce a mixture having a minimum cost per bag? What is the minimum cost of the mixture per bag?
View SolutionA farm is engaged in breeding pigs. The pigs are fed on various products grown on the farm. To ensure certain nutrient constituents X,Y and Z, It is necessary to mix two types of feeds A and B . One unit of product A contains 36 units of X, 3 units of Y and 20 units of Z. One unit of product B contains 6 units of X, 12 units of Y and 10 units of Z. The minimum requirement of X,Y and Z is 108 units, 36 units and 100 units respectively. Product A costs Rs. 20/unit and product B costs Rs 40/unit. Formulate this problem sa a linear programming problem to minimize the total cost.
View SolutionThe final product of a firm has a requirement that it must weigh at least 150 kg. The two raw materials used in the manufacture of this product are A with a cost of Rs. 2 per unit and B with a cost of Rs. 8 per unit. Each unit of A weighs 5 kg and each unit of B weighs 10 kg. At least 14 units of B and not more than 20 units of A must be used. What quantity of raw material of each type should be used for each unit of final product so as to minimize cost? Pose the problem as an LPP and solve it by graphical method.
View Solution
