Find the derivative of the function y= f(x) using the derivative of the inverse function x=f−1(y) in the following
y=3√x+4
Text Solution
The correct Answer is:x≠−4
|
Updated on:21/07/2023
Similar Questions
Knowledge Check
- Question 1 - Select One
Find the derivatives of each of the following functions :
f(x)=8x4A30x2B32x3C32xDइनमे से कोई नहीं - Question 2 - Select One
The figure shown below is the graph of the derivative of some function y=f'(x)'.
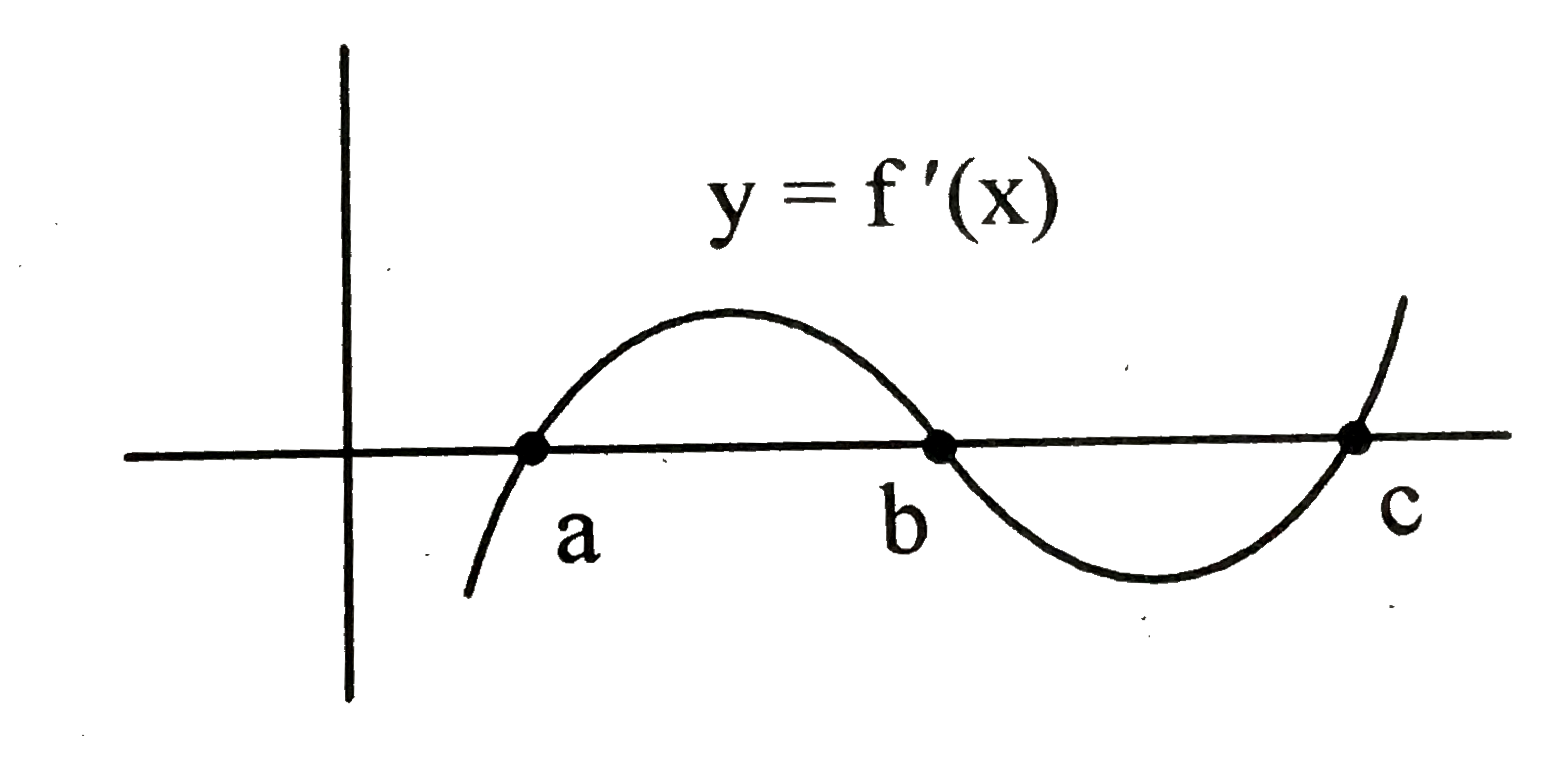
ThenAf has local minima at x=a,b and a local maximum at x=cBf has local minima at x=b,c and a local maximum at x=aCf has local minima at x=c,a and a local maximum at x=bDthe given figure is insufficient to conclude any thing about the local minima and local maxima of f
Find the derivatives of the following (1-3) functions :
y=x2View SolutionFind the derivative of the inverse of functions y=2x3−6x and calculate its value at x=−2
View Solutionfind the derivatives of the following functions : (a) y=x3,(b)y=1/x2
View SolutionIf y = f(x) is a derivable function of x such that the inverse function x=f−1(y) is defined, then show that dxdy=1(dy/dx), where dydx≠0.
View SolutionUsing the first derivative , find the extreme of the following functions :
f(x)=33√x2−x2,View SolutionFind derivative of y=1(f(x))n
View Solution

