Assertion (A): Two parallel metal plates having charge +Q and -Q are facing at a distance between them. The plates are now immersed in kerosene oil and the electric potential between the plates decreases.
Reason (R ): Dielectric constant of kerosene oil is less than 1.
Both A and R are true and R is the correct explanation of A
Both A and R are true but R is NOT the correct explanation of A
A is true but R is false
A is false and R is True
The correct Answer is:C
To analyze the assertion and reason provided in the question, let's break it down step by step.
Step 1: Understand the Assertion
The assertion states that when two parallel metal plates with charges +Q and -Q are immersed in kerosene oil, the electric potential between the plates decreases.
Step 2: Initial Capacitance in Air
Initially, when the plates are in air, the capacitance C0 of the parallel plates can be expressed as:
C0=ε0Ad
where:
- ε0 is the permittivity of free space,
- A is the area of the plates,
- d is the distance between the plates.
Step 3: Final Capacitance in Kerosene Oil
When the plates are immersed in kerosene oil, the capacitance C changes due to the dielectric constant k of the kerosene oil:
C=k⋅C0=kε0Ad
Step 4: Calculate Initial and Final Potential Difference
The potential difference V between the plates is given by:
V=QC
- Initial Potential Difference:
Vinitial=QC0=Qdε0A
- Final Potential Difference:
Vfinal=QC=Qdkε0A
Step 5: Compare Initial and Final Potential Differences
To find the relationship between the initial and final potential differences, we can take the ratio:
VfinalVinitial=Qdkε0AQdε0A=1k
This indicates that:
Vfinal=Vinitialk
Since k>1 (for most dielectrics), it follows that Vfinal<Vinitial. Thus, the electric potential decreases when the plates are immersed in kerosene oil.
Step 6: Evaluate the Reason
The reason states that the dielectric constant of kerosene oil is less than 1. This is incorrect, as the dielectric constant of kerosene oil is actually greater than 1. Therefore, the assertion is true, but the reason is false.
Conclusion
- Assertion (A): True
- Reason (R): False
Final Answer
The assertion is true while the reason is false.
---
The assertion states that when two parallel metal plates with charges +Q and -Q are immersed in kerosene oil, the electric potential between the plates decreases.
Initially, when the plates are in air, the capacitance C0 of the parallel plates can be expressed as:
C0=ε0Ad
where:
- ε0 is the permittivity of free space,
- A is the area of the plates,
- d is the distance between the plates.
When the plates are immersed in kerosene oil, the capacitance C changes due to the dielectric constant k of the kerosene oil:
C=k⋅C0=kε0Ad
The potential difference V between the plates is given by:
V=QC
- Initial Potential Difference:
Vinitial=QC0=Qdε0A
- Final Potential Difference:
Vfinal=QC=Qdkε0A
To find the relationship between the initial and final potential differences, we can take the ratio:
VfinalVinitial=Qdkε0AQdε0A=1k
This indicates that:
Vfinal=Vinitialk
Since k>1 (for most dielectrics), it follows that Vfinal<Vinitial. Thus, the electric potential decreases when the plates are immersed in kerosene oil.
The reason states that the dielectric constant of kerosene oil is less than 1. This is incorrect, as the dielectric constant of kerosene oil is actually greater than 1. Therefore, the assertion is true, but the reason is false.
- Assertion (A): True
- Reason (R): False
The assertion is true while the reason is false.
Similar Questions
Charges 2q and −3q are given to two identical metal plates of area of cross section A. the distance between the plates is d. Find the capacitance and potential difference between the plates.
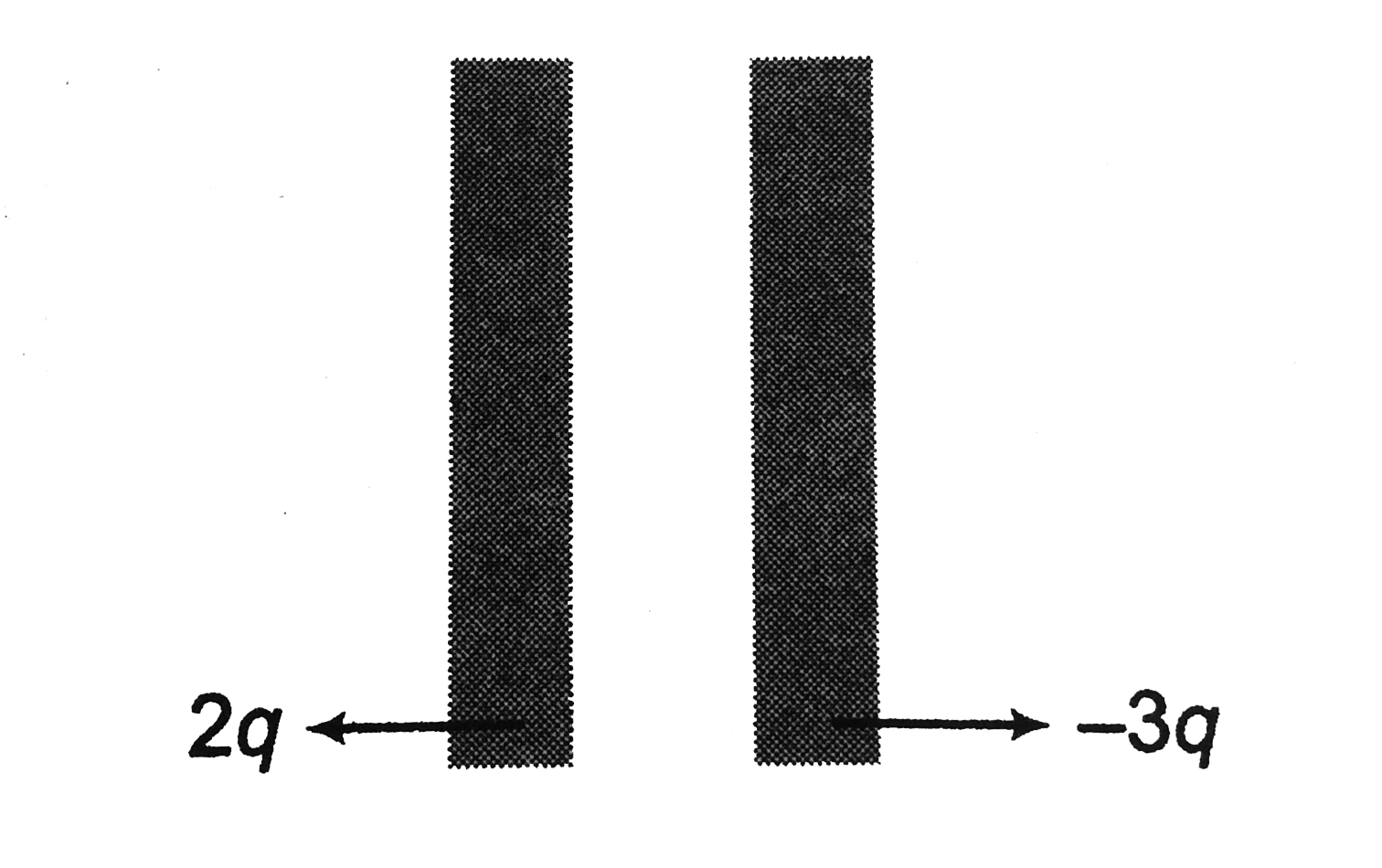
View SolutionA parallel plate air condenser is charged and then disconnected from the charging battery. Now the space between the plates is filled with a dielectric then, it electric field strength between the plates
View Solution
Knowledge Check
Two parallel metal plates having charges +Q and −Q face each other at a certain distance between them.If the plates are now dipped in kerosene oil tank ,the electric field between the plates will
Abecomes zeroBincreaseCdecreaseDremain sameA parallel plate condenser is immersed in an oil of dielectric constant 2. The field between the plates is
Aincreased proportional to 2Bdecreased proportional to 12Cincreased proportional to √2Ddecreased proportional to 1√2An isolated charged capacitor with vacuum between its plates is dipped into an oil tank. If the oil is pumped out, the electric field between the plates of capacitor will
AincreaseBdecreaseCremain unchangeedDbecome zero
An isolated charged capacitor with vacuum between its plates is dipped into an oil tank. If the oil is pumped out, the electric field between the plates of capacitor will
View SolutionBy inserting a plate of dielectric material between the plates of a parallel plate capacitor at constant potential, the energy is increased five times. The dielectric constant of the material is
View SolutionCapacitor of capacitance C with oil between the plates of dielectric constant 2. If oil is removed, the capacitance is .
View SolutionA parallel plate capacitor with air between the plates has a capacitance C. If the distance between the plates is doubled and the space between the plates is filled with a dielectric of dielectric constant 6, then the capacitance will become.
View SolutionA parallel-plate capacitor is filled with a dielectric upto one-half of the distance between the plates. The manner in which, the potential between the plates varies is illustrated in the figure
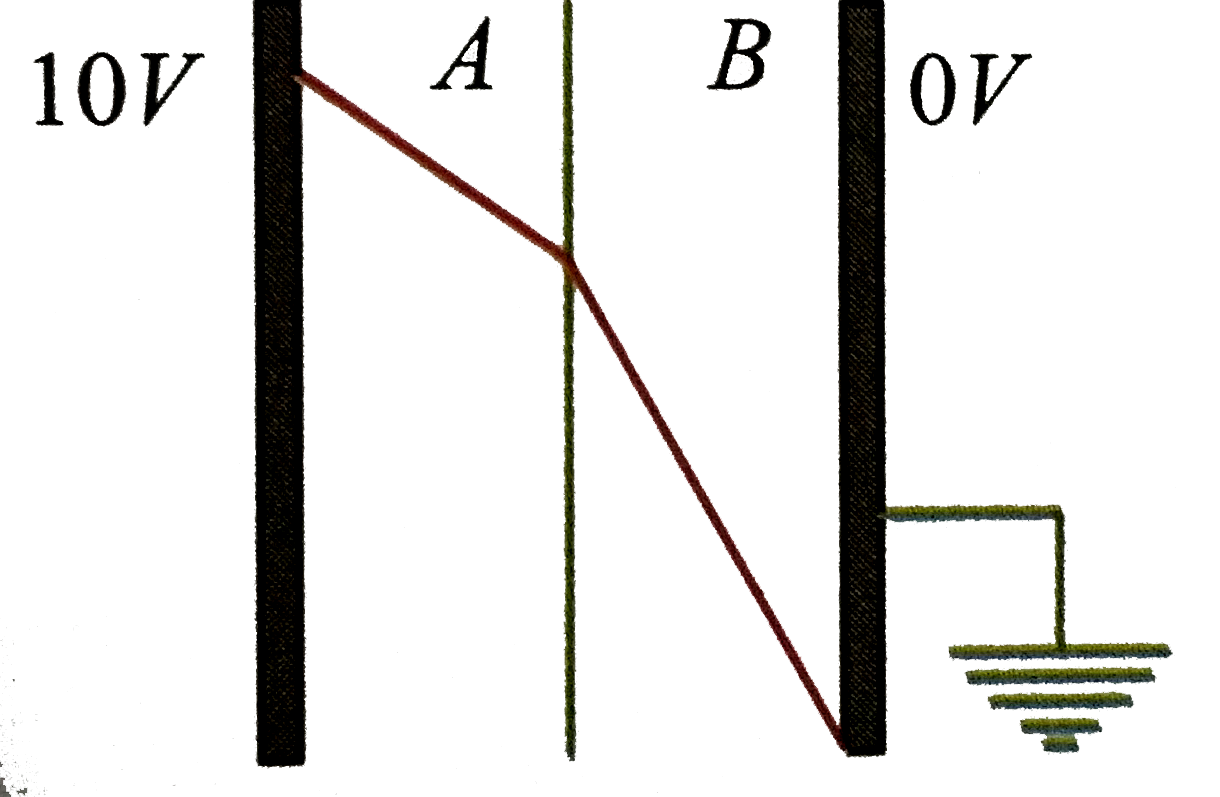 .
.
View Solution
