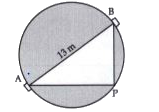
In the Dwarika residency colony, there is a circular park of diameter 13 metres. A pole has to erected at the point P on the boundary of circular park in such a way that the difference of its distances from two diametrically opposite fixed gates A and B on the boundary is 7 metres.
For equation, is called the .....
Discriminant
Distinct roots
Quadratic equation
None of these
Step by step video & image solution for In the Dwarika residency colony, there is a circular park of diameter 13 metres. A pole has to erected at the point P on the boundary of circular park in such a way that the difference of its distances from two diametrically opposite fixed gates A and B on the boundary is 7 metres. For equation, ax^(2) + bx + c=0, b^(2)-4ac is called the ..... by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - OSWAL PUBLICATIONCHAPTER - QUADRATIC EQUATIONSEXERCISE - SELF ASSESSMENT -2 (Multiple Choice Questions:)3videos
QUADRATIC EQUATIONS
- BOOK - OSWAL PUBLICATIONCHAPTER - QUADRATIC EQUATIONSEXERCISE - SELF ASSESSMENT -2 (Fill in the Blanks:)3videos
QUADRATIC EQUATIONS
- BOOK - OSWAL PUBLICATIONCHAPTER - QUADRATIC EQUATIONSEXERCISE - SELF ASSESSMENT -1 (Long Answer Type Questions-III)2videos
QUADRATIC EQUATIONS
- BOOK - OSWAL PUBLICATIONCHAPTER - PROBABILITYEXERCISE - Self Assessment66videos
PROBABILITY
- BOOK - OSWAL PUBLICATIONCHAPTER - REAL NUMBERSEXERCISE - Self-Assessment11videos
REAL NUMBERS
Similar Questions
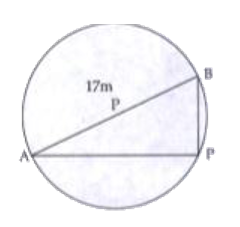
Aniket is studying in X standard. He created a pole at on the boundary of a circular park of diameter 17 m in such a way that the difference of its distances from two diametrically opposite fixed gets A and B on the boundary is 7m.
Find the area of triangle ABP.View Solution
Aniket is studying in X standard. He is created a pole at on the boundary of a circular park of diameter 17 m in such a way that the difference of its distances from two diametrically opposite fixed gets A and B on the boundary is 7m.
What is the length of (AP+BP) ?View SolutionA pole has to be erected at a point on the boundary of a circular park of diameter 13 metres in such a way that the difference of its distances from two diametrically opposite fixed gates A and B on the boundary is 7 metres. Is it the possible to do so? If yes, at what distances from the two gates should the pole be erected?
View SolutionA pole has to be erected at a point on the boundary of a circular park of diameter 13 metres in such a way that the differences of its distances from two diametrically opposite fixed gates A and B on the boundary is 7 metres. Is it possible to do so? If yes, at what distances from the two gates should the pole be erected?
View Solution
Aniket is studying in X standard. He is created a pole at on the boundary of a circular park of diameter 17 m in such a way that the difference of its distances from two diametrically opposite fixed gets A and B on the boundary is 7m.
Find the distance between pole and gate B.View Solution
Aniket is studying in X standard. He is created a pole at on the boundary of a circular park of diameter 17 m in such a way that the difference of its distances from two diametrically opposite fixed gets A and B on the boundary is 7m.
Find the distance between pole and gate A.View Solution
Aniket is studying in X standard. He is created a pole at on the boundary of a circular park of diameter 17 m in such a way that the difference of its distances from two diametrically opposite fixed gets A and B on the boundary is 7m.
Find a quadratic equations in variable x for above situation.View Solution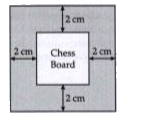
Sohan and Mohan are playing a chess on Sunday. The chess board contains equal squares and the are of each equal square is 6.25m2.
A border round the board is 2 cm wide.
For equation ax2+bx+c=0,x=−b±√b2−4ac2a is called the.......View SolutionIf the roots of the equation ax2+bx+c=0 differ by K then b2−4ac=
View SolutionFind the locus of appoint which moves such that the sum of the squares of its distance from the points A(1,2,3),B(2,−3,5)andC(0,7,4)is120.
View Solution

