What is the angle between the two tangents drawn from an external point to a circle and the angle subtended by the line-segment joining the points of the contact at the centre.
To solve the problem of finding the angle between the two tangents drawn from an external point to a circle and the angle subtended by the line segment joining the points of contact at the center, we can follow these steps:
1. Identify the Elements:
- Let the circle be centered at point O.
- Let P be the external point from which the tangents PA and PB are drawn to the circle, touching the circle at points A and B respectively.
2. Understand the Tangent Properties:
- The radius of the circle (OA and OB) is perpendicular to the tangent at the point of contact. Therefore, we have:
- ∠OAP = 90° (angle between radius OA and tangent PA)
- ∠OBP = 90° (angle between radius OB and tangent PB)
3. Consider the Quadrilateral:
- The points A, O, B, and P form a quadrilateral AOBP. The sum of the angles in any quadrilateral is 360°.
- Therefore, we can write:
∠A+∠O+∠B+∠P=360°
4. Substituting Known Angles:
- We know that:
- ∠A = 90°
- ∠B = 90°
- Substitute these values into the equation:
90°+∠O+90°+∠P=360°
5. Simplifying the Equation:
- Combine the known angles:
180°+∠O+∠P=360°
- Rearranging gives:
∠O+∠P=360°−180°=180°
6. Conclusion:
- The angle subtended at the center (∠O) and the angle at the external point (∠P) are supplementary, meaning:
∠O+∠P=180°
- Therefore, the angle between the two tangents (∠P) is equal to the angle subtended by the line segment joining the points of contact (∠O) at the center.
Final Answer:
The angle between the two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line segment joining the points of contact at the center, summing up to 180°.
- Let the circle be centered at point O.
- Let P be the external point from which the tangents PA and PB are drawn to the circle, touching the circle at points A and B respectively.
- The radius of the circle (OA and OB) is perpendicular to the tangent at the point of contact. Therefore, we have:
- ∠OAP = 90° (angle between radius OA and tangent PA)
- ∠OBP = 90° (angle between radius OB and tangent PB)
- The points A, O, B, and P form a quadrilateral AOBP. The sum of the angles in any quadrilateral is 360°.
- Therefore, we can write:
∠A+∠O+∠B+∠P=360°
- We know that:
- ∠A = 90°
- ∠B = 90°
- Substitute these values into the equation:
90°+∠O+90°+∠P=360°
- Combine the known angles:
180°+∠O+∠P=360°
- Rearranging gives:
∠O+∠P=360°−180°=180°
- The angle subtended at the center (∠O) and the angle at the external point (∠P) are supplementary, meaning:
∠O+∠P=180°
- Therefore, the angle between the two tangents (∠P) is equal to the angle subtended by the line segment joining the points of contact (∠O) at the center.
The angle between the two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line segment joining the points of contact at the center, summing up to 180°.
Topper's Solved these Questions
- CONSTRUCTIONSBOOK - OSWAL PUBLICATIONCHAPTER - CONSTRUCTIONSEXERCISE - self assesment -2 (II. Short Answer Type Questions)
3 Videos
- CONSTRUCTIONSBOOK - OSWAL PUBLICATIONCHAPTER - CONSTRUCTIONSEXERCISE - self assesment -2 (III. Short Answer Type Questions)
1 Videos
- CONSTRUCTIONSBOOK - OSWAL PUBLICATIONCHAPTER - CONSTRUCTIONSEXERCISE - self assesment -2 (Fill in the blanks)
3 Videos
- CIRCLES BOOK - OSWAL PUBLICATIONCHAPTER - CIRCLES EXERCISE - SELF ASSESSMENT
5 Videos
- COORDINATE GEOMETRYBOOK - OSWAL PUBLICATIONCHAPTER - COORDINATE GEOMETRYEXERCISE - SELF ASSESSMENT
20 Videos
Similar Questions
Prove that the angle between the two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line-segment joining the points of contact at the centre.
View SolutionProve that the angle between two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line segments joining the points of contact at the centre.
View Solution
Knowledge Check
If the angle between two tangents drawn from an external point P to a circle of radius a and center O , is 60∘, then find the length of OP.
A2aB4aC6aD8a
Prove that the angle between the two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line segments joining the points of contact at the centre.
View SolutionProve that the angle between the two tangents drawn from an external point are supplementary to the angle subtended by the line segment joining the centre.
View SolutionThe lengths of tangents drawn from an external point to a circle are ________.
View SolutionTheorem: The length of two tangents drawn from an external point to a circle are equal.
View SolutionThe number of tangents drawn from an external point to a circle is _____________.
View Solution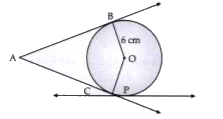
The length of tangents drawn from the external point to a circle are ........... .
View SolutionIf the angle between two tangents drawn from an external point ‘P’ to a circle of radius ‘r’ and centre O is 60∘, then find the length of OP.
View Solution
