The thickness of a cylinder is 1 foot, the inner radius of the cylinder is 3 feet and height is 7 feet. To paint the inner suface it requires one litre of a particular colour . How much quantity of the same colour is required to paint all the surfaces of the cylinder?
73litre
32 litre
83 litre
103 litre
To solve the problem of how much paint is required to cover all the surfaces of the cylinder, we will follow these steps:
Step 1: Identify the given data
- Inner radius (r) = 3 feet
- Thickness of the cylinder = 1 foot
- Height (h) = 7 feet
- Paint required for the inner surface area = 1 liter
Step 2: Calculate the inner surface area of the cylinder
The formula for the curved surface area of a cylinder is given by:
Curved Surface Area=2πrh
Using the inner radius:
Inner Surface Area=2π(3)(7)=42π square feet
Step 3: Calculate the outer radius of the cylinder
The outer radius (R) can be calculated by adding the thickness to the inner radius:
R=r+thickness=3+1=4 feet
Step 4: Calculate the outer surface area of the cylinder
Using the outer radius:
Outer Surface Area=2πRh=2π(4)(7)=56π square feet
Step 5: Calculate the area of the top and bottom rims
The area of the top and bottom rims can be calculated using the formula for the area of a ring:
Area of rims=2×π(R2−r2)
Calculating the area:
R2=42=16
r2=32=9
R2−r2=16−9=7
Thus,
Area of rims=2×π(7)=14π square feet
Step 6: Calculate the total surface area to be painted
The total surface area to be painted is the sum of the inner surface area, outer surface area, and the area of the rims:
Total Area=Inner Surface Area+Outer Surface Area+Area of rims
Total Area=42π+56π+14π=112π square feet
Step 7: Calculate the quantity of paint required
We know that 1 liter of paint is required for the inner surface area of 42π square feet. To find out how much paint is needed for 112π square feet, we set up the proportion:
Paint required=1 liter42π×112π
Cancelling π:
Paint required=11242=83 liters
Final Answer
The quantity of paint required to paint all the surfaces of the cylinder is 83 liters.
---
- Inner radius (r) = 3 feet
- Thickness of the cylinder = 1 foot
- Height (h) = 7 feet
- Paint required for the inner surface area = 1 liter
The formula for the curved surface area of a cylinder is given by:
Curved Surface Area=2πrh
Inner Surface Area=2π(3)(7)=42π square feet
The outer radius (R) can be calculated by adding the thickness to the inner radius:
R=r+thickness=3+1=4 feet
Using the outer radius:
Outer Surface Area=2πRh=2π(4)(7)=56π square feet
The area of the top and bottom rims can be calculated using the formula for the area of a ring:
Area of rims=2×π(R2−r2)
Calculating the area:
R2=42=16
r2=32=9
R2−r2=16−9=7
Thus,
Area of rims=2×π(7)=14π square feet
The total surface area to be painted is the sum of the inner surface area, outer surface area, and the area of the rims:
Total Area=Inner Surface Area+Outer Surface Area+Area of rims
Total Area=42π+56π+14π=112π square feet
We know that 1 liter of paint is required for the inner surface area of 42π square feet. To find out how much paint is needed for 112π square feet, we set up the proportion:
Paint required=1 liter42π×112π
Cancelling π:
Paint required=11242=83 liters
The quantity of paint required to paint all the surfaces of the cylinder is 83 liters.
Similar Questions
A closed cylindrical container, the radius of which is 7 cm and height 10 cm is to be made out of a metal sheet. Find
(a) the area of metal sheet required .
(b) the volume of the cylinder made.
(c ) the cost of painting the lateral surface of the cylinder at the rate of Rs. 4 per cm2.
View SolutionRahul an engieering student, prepared a model shaped like a cylinder with two cones attached at its ens. The diameter of the model is 3 cm and its length is 12 cm .If each conical part has a model (assume the oute and inner dimensions of the model to be nearly the same ),(ii) cost of painting the outer surface of the model at ₹ 12. 50 per cm2
View Solution
Knowledge Check
The base radius of the cylinder is 1 2/3 times its height. The cost of painting its C.S.A. at 2 paise/cm2 is Rs 92.40. The volume of the cylinder is
A80850 cm3B88850 cm3C80508 cm3DNone of theseThe cost of painting the total outside surface of a closed cylinder at Rs 3 per cm2 is Rs 2772. If the height of the cylinder is 2 times the radius , then find its volume .
A34,312cm3B3342cm3C2154cm3D2156cm3
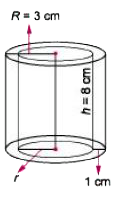
A hollow cylinder made of wood has thickness 1 cm while its external radius is 3 cm. If the height of the cylinder is 8 cm, then find the volume, curved surface area and total surface area of the cylinder.
View SolutionThe thickness of a hollow wooden cylinder is 2cm. It is 35cm long and its inner radius is 12cm.
Find the volume of the wood required to make the cylinder, assuming it is open at either end.
View SolutionThe thickness of a hollow wooden cylinder is 2cm. It is 35cm long and its inner radius is 12cm. Find the volume of the wood required to make the cylinder, assuming it is open at either end.
View SolutionA cylinder of mass M and radius R moves with constant speed v through a region of space that contains dust particles of mass m which is at rest. There are n number of particles per unit volume. The cylinder moves in a direction perpendicular to its axis. Assume m<<M, and assumes the particles do not interact wilth each other. All the collision takes place is perfectly elastic and the surface of the cylinder is smooth. The drag force per unit length of the cyliner require to maintain the speed v contant for the cylinder is K3nmRv2. Find the value of K?

View SolutionThe volume of a conical frustum and that of a cylinder are same. The height of the cylinder is 1/3 m and radius is 62 m. The height of the frustum is 31 m and the radius of one end is 10 m. Find the radius of the other end.
View SolutionA glass is in the shape of a cylinder of radius 7 cm and height 10 cm. Find the volume of juice in litre required to fill 6 such glasses. [Use π=227]
View Solution
