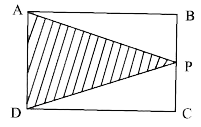
A line through the vertex A of a parallelogram ABCD meets DC in P and BC produced in Q. If P is the mid-point of DC, then which of the following is $/$ arre correct?
1. Area of $Δ P D A$ is equal to that of $Δ P C Q$
2. Area of $Δ Q A B$ is equal to twice that of $Δ P C Q$
Select the correct answer using the code given below $:$

1 only

2 only

Both 1 and 2

Neither 1 nor 2

Video Solution

Text Solution

Generated By DoubtnutGPT

To solve the problem, we need to analyze the given parallelogram ABCD and the triangles formed by the line through vertex A.
1. Draw the Parallelogram:
- Draw parallelogram ABCD with vertices A, B, C, and D.
- Label the sides such that AB is parallel to DC and AD is parallel to BC.
2. Identify Points P and Q:
- Draw a line from vertex A that intersects side DC at point P and extends to meet the line BC produced at point Q.
- According to the problem, point P is the midpoint of side DC.
3. Establish Lengths:
- Since P is the midpoint of DC, we can denote the lengths as follows:
- Let DP = PC = x (where x is half the length of DC).
4. Analyze Triangles PDA and PCQ:
- We need to compare triangles PDA and PCQ.
- From the properties of the triangles, we can observe:
- Angle APD = Angle CPQ (alternate interior angles).
- Angle ADP = Angle PCQ (corresponding angles).
- Angle DAP = Angle CQP (remaining angles).
- Since two angles are equal and one side (PD = PC) is equal, by the Angle-Angle-Side (AAS) congruency criterion, triangles PDA and PCQ are congruent.
5. Conclusion for Statement 1:
- Since the triangles PDA and PCQ are congruent, their areas are equal.
- Thus, Area of triangle PDA = Area of triangle PCQ (Statement 1 is true).
6. Analyze Triangles QAB and PCQ:
- Now, we need to compare triangles QAB and PCQ.
- We know that AB = 2x (since opposite sides of a parallelogram are equal).
- The triangles QAB and PCQ share angle QAB and have angle QBA equal to angle QCP (by the properties of parallel lines).
- Therefore, triangles QAB and PCQ are similar (AA criterion).
7. Area Ratio of Similar Triangles:
- The ratio of the areas of similar triangles is equal to the square of the ratio of their corresponding sides.
- Thus, we can write:
$\frac{Area of P C Q}{Area of Q A B} = {(\frac{P C}{A B})}^{2} = {(\frac{x}{2 x})}^{2} = {(\frac{1}{2})}^{2} = \frac{1}{4}$
- This implies that:
$Area of Q A B = 4 \times Area of P C Q$
- Therefore, Area of triangle QAB is equal to four times that of triangle PCQ, which contradicts the statement that it is twice (Statement 2 is false).
Final Answer:
- The correct answer is that only Statement 1 is true.

Updated on:8/8/2024

ABC is a triangle right- angled at B and D is a point on BC produced $(B D > B C)$ , such that $B D = 2 D C .$ Which one of the following is correct?
A $A C^{2} = A D^{2} - 3 C D^{2}$
B $A C^{2} = A D^{2} - 2 C D^{2}$
C $A C^{2} = A D^{2} - 4 C D^{2}$
D $A C^{2} = A D^{2} - 5 C D^{2}$
In a triangle ABC, it is known that AB=AC. Suppose D is the mid-point of AC and BD=BC=2. Then the area of the triangle ABC is-
A2
B $2 \sqrt{2}$
C $\sqrt{7}$
D $2 \sqrt{7}$