Equal loads are suspended using identical wires of steel and copper. Which wire is elongated more ?
To determine which wire, steel or copper, will elongate more when equal loads are suspended from them, we can use the concept of Young's modulus of elasticity. Here’s a step-by-step solution:
Step 1: Understand Young's Modulus
Young's modulus (E) is defined as the ratio of stress (force per unit area) to strain (relative change in length). Mathematically, it is given by:
E=F/AΔL/L
Where:
- E = Young's modulus
- F = Force applied (equal load in this case)
- A = Cross-sectional area of the wire
- ΔL = Change in length (elongation)
- L = Original length of the wire
Step 2: Compare Young's Modulus of Steel and Copper
The Young's modulus for steel is significantly higher than that for copper. This means that steel is stiffer than copper. For typical values:
- Young's modulus of steel Esteel≈200GPa
- Young's modulus of copper Ecopper≈110GPa
Step 3: Relate Elongation to Young's Modulus
From the formula for Young's modulus, we can rearrange it to find the elongation:
ΔL=FLAE
This shows that elongation (ΔL) is inversely proportional to Young's modulus (E).
Step 4: Analyze the Elongation for Both Wires
Since both wires are subjected to the same force (equal loads) and have the same dimensions (identical wires), we can compare their elongations:
- For steel:
ΔLsteel=FLAEsteel
- For copper:
ΔLcopper=FLAEcopper
Step 5: Conclusion
Since Esteel>Ecopper, it follows that:
ΔLsteel<ΔLcopper
Thus, the copper wire will elongate more than the steel wire when equal loads are suspended from them.
Final Answer
The copper wire is elongated more than the steel wire.
---
Young's modulus (E) is defined as the ratio of stress (force per unit area) to strain (relative change in length). Mathematically, it is given by:
E=F/AΔL/L
Where:
- E = Young's modulus
- F = Force applied (equal load in this case)
- A = Cross-sectional area of the wire
- ΔL = Change in length (elongation)
- L = Original length of the wire
The Young's modulus for steel is significantly higher than that for copper. This means that steel is stiffer than copper. For typical values:
- Young's modulus of steel Esteel≈200GPa
- Young's modulus of copper Ecopper≈110GPa
From the formula for Young's modulus, we can rearrange it to find the elongation:
ΔL=FLAE
This shows that elongation (ΔL) is inversely proportional to Young's modulus (E).
Since both wires are subjected to the same force (equal loads) and have the same dimensions (identical wires), we can compare their elongations:
- For steel:
ΔLsteel=FLAEsteel
- For copper:
ΔLcopper=FLAEcopper
Since Esteel>Ecopper, it follows that:
ΔLsteel<ΔLcopper
Thus, the copper wire will elongate more than the steel wire when equal loads are suspended from them.
The copper wire is elongated more than the steel wire.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - ELASTICITYEXERCISE - MANDATORY EXERCISE (Exercise Set II) Multiple - Choice Questions with One correct Answer21videos
ELASTICITY
- BOOK - CENGAGE PHYSICSCHAPTER - ELASTICITYEXERCISE - CONSOLIDATED EXERCISE5videos
ELASTICITY
- BOOK - CENGAGE PHYSICSCHAPTER - ELASTICITYEXERCISE - MANDATORY EXERCISE (Exercise Set I)6videos
ELASTICITY
- BOOK - CENGAGE PHYSICSCHAPTER - DESCRIPTION OF MOTIONEXERCISE - OLYMPIAD AND NTSE LEVEL EXERCISE10videos
DESCRIPTION OF MOTION
- BOOK - CENGAGE PHYSICSCHAPTER - FRICTIONEXERCISE - OLYMPIAD AND NTSE LEVEL EXERCISES (Read the given statements and select the correct option.)1videos
FRICTION
Similar Questions
Why are copper wires used as connected wires ?
View SolutionA weight of 100N is suspended by two wires made by steel and copper as shown in figure length of steel wire is 1m and copper wire is √3m. Find ratio of change in length of copper wire (Δlc) to change in length of steel wire (Δls). given Young's modulus YSteel=2×1011N/m2,YCopper=1×1011N/m2
_PHY_E01_017_Q01.png) View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Assertion : identical springs of steel and copper are equally stretched. More work will be done on the steel spring.
Reason : Steel is more elastic than copper.A(a) If both Assertion and Reason are true and the Reason is correct explanation of the Assertion.B(b) If both Assertion and Reason are true but Reason is not the correct explanation of Assertion.CIf Assertion is true, but the Reason is false.DIf Assertion is false but the Reason is true. - Question 2 - Select One
A copper wire and a steel wire of the same length and same cross-section are joined end to end to form a composite wire. The composite wire is hung form a rigid support and a load is suspended form the other end. If the increses in length of the composite wire is 2.4mm. then the increases in lenghts of steel and copper wires are
A0.1mm,2.0mmB1.2mm,1.2mmC0.6mm,1.8mmD0.8mm,1.6mm - Question 3 - Select One
Two wire of same radius and lengh the are subjected to the same load. One wire is of steel and the other is of copper. If the Young's modulus of steel is twice that of copper, the ratio the energy stored per unit volume in steel to that of copper wire is
A1:2B2:1C1:4D4:1
(i) Two identical rods, one of steel, the other of copper, are stretched by an identical amount. On which operation more work is expended?
(ii) Two identical rods, one of steel, the other of copper, are stretched with equal force. On which operation is more work needed?View SolutionCan we use copper wire as potentiometer wire ? Explain.
View SolutionA homogeneous block with a mass m hangs on three vertical wires of equal length arranged symmetrically . Find the tensions of the wires if the middle wire is of steel and the other two are of copper . All the wires have the same cross - section . Consider the modulus of elasticity of steel to be double than that of copper .
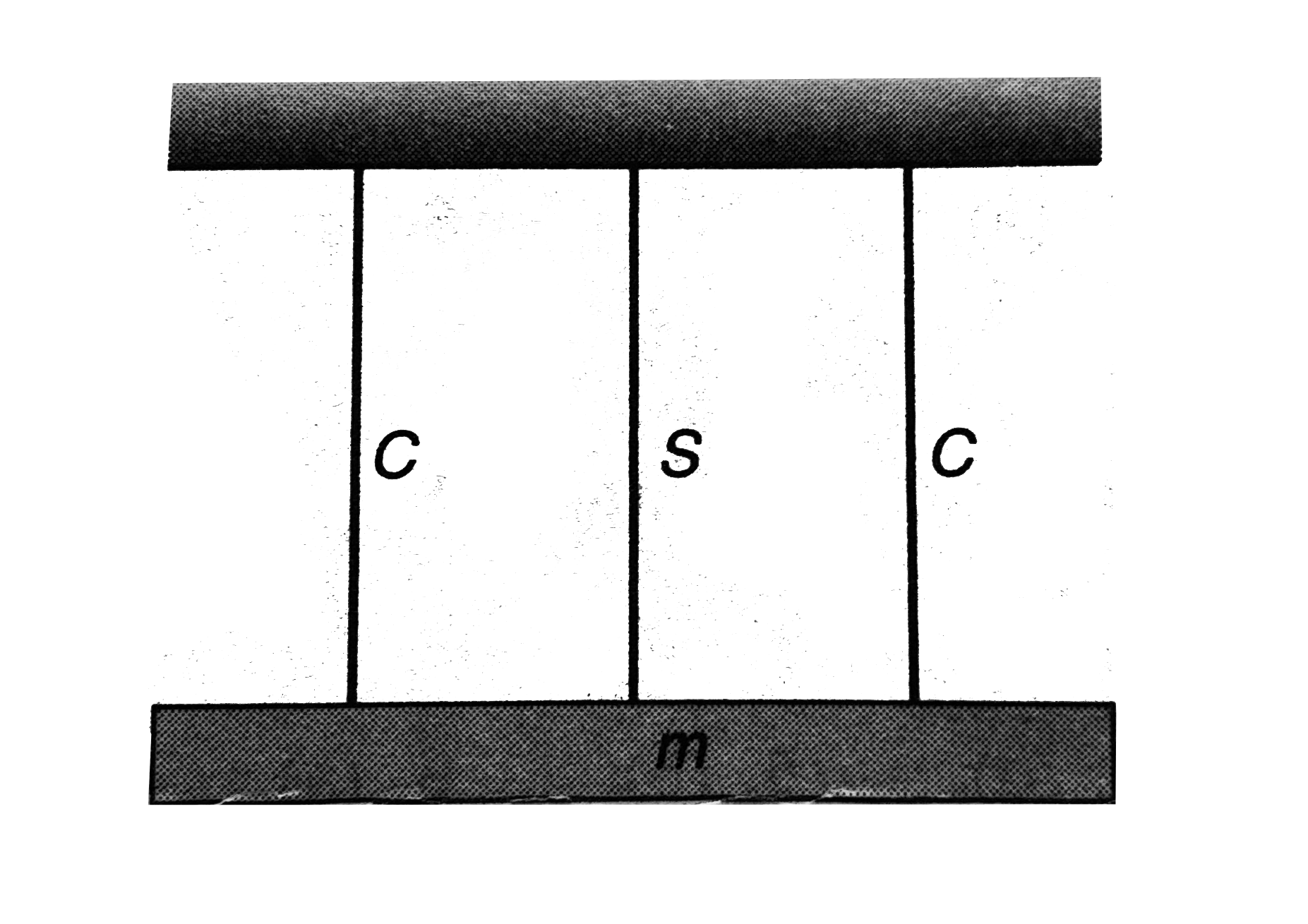 View Solution
View SolutionAssertion : Identical springs of steel and copper are equally stretched. More work will be done on the steel spring.
Reason : Steel is more elastic than copperView SolutionTwo wires of equal cross-section but one made of steel and the other of copper are joined end to end. When the cobination is kept under tension, the elongations in the two wires are found to be equal elongations in the two wire are found to be equal. What is the ratio of the lengths of the two wires?
(Given, Young's modulus of steel =2×1011Nm−2 and young's modulus of copper =1.1×1011Nm−2)View Solution

