BDS812YMGJET?13UDYKJI9?FST
12,10
10,12
9,14
None of there
The correct Answer is:B
The given sequence of number follows the pattern:
BDS⇒[3+(B+D+S)5]=[3+(2+4+19)5]=[3+255]=8
YMF⇒[3+(Y+M+G)5]=[3+(25+13+7)5]=12
JET⇒[3+(J+E+T)5]=[3+(10+5+20)5]=10
UDY⇒[3+(U+D+Y)5]=[3+(21+4+25)5]=13
KJI⇒[3+(K+J+I)5]=[3+(11+10+9)5]=9
FST⇒[3+(F+S+T)5]=[3+(6+19+20)5]=12
Therefore, missing letter number = 10 and 12.
Topper's Solved these Questions
- BOOK - LUCENT PUBLICATIONCHAPTER - SERIES COMPLETION : MIXED SERIESEXERCISE - Exercise (Type-I: Missing Letter-Number Group)5videos
SERIES COMPLETION : MIXED SERIES
- BOOK - LUCENT PUBLICATIONCHAPTER - SERIES COMPLETION : MIXED SERIESEXERCISE - Exercise (Type-II: Wrong Letter Number Group)2videos
SERIES COMPLETION : MIXED SERIES
- BOOK - LUCENT PUBLICATIONCHAPTER - SERIES COMPLETION : ALPHABETEXERCISE - EXERCISE 38videos
SERIES COMPLETION : ALPHABET
- BOOK - LUCENT PUBLICATIONCHAPTER - SERIES COMPLETION : NUMBER EXERCISE - EXERCISE-317videos
SERIES COMPLETION : NUMBER
Similar Questions
R is a relation from {11, 12, 13} to {8, 10, 12} defined by y=x−3 . Then, R−1 is (a) {(8, 11), (10, 13)} (b) {(11, 8), (13, 10)} (c) {(10, 13), (8, 11), (8, 10)} (d) none of these
View SolutionIf R is a relation from set A={11,12,13} to set B={8,10,12} defined by y=x−3, then write R−1.
A.{(8,11),(10,13)}
B. {(8,11),(10,12)}
C. {(10,13),(10,11)}
D. none of these
ANSWER: AView Solution
Knowledge Check
- Question 1 - Select One
87101213121517109?14
A12B10C21D25 - Question 2 - Select One
8131071291015?
A5B19C12D8 - Question 3 - Select One
7866591213?504520486
A7B12C8D9
Find the sum of deviations of the following data measured from their actual mean.
10.3, 12.5, 13.7, 9.4, 8.6, 11.2, 12.1, 10.9, 13.2, 15.4, 14.7, 9.1, 14.2, 15.8, 7.8.View SolutionLet (nk) denote nCk and
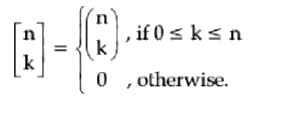
If Ak=9∑i=0(9i)[ 1212−k+i]+8∑i=0(8i)[ 1313−k+i]and A4−A3=190p ,then p is equal toView Solution911131315171012141416181113?
View Solution9,12,11,14,13…15
View SolutionSTATEMENT-1 The points A(2, 9, 12), B(1, 8, 8), C(-2, 11, 8) and D(-1, 12, 12) are the vertices of rhombus.
and
STATEMENT-2 : AB=BC=CD=DAandAC≠BD then the quadrilateral ABCD is called a rhombus.View Solution

