The mode of a distribution is 55 and the modal class is 45-60 and the frequency preceding the modal class is 5 and the frequnecy after the modal class is 10 . Find the frquency of the modal class .
To find the frequency of the modal class, we can use the formula for mode in a grouped frequency distribution. The formula is:
Mode=L+F1−F02F1−F0−F2×H
Where:
- L = lower limit of the modal class
- F1 = frequency of the modal class (what we need to find)
- F0 = frequency of the class preceding the modal class
- F2 = frequency of the class succeeding the modal class
- H = class width (size)
Step 1: Identify the values
From the question:
- The mode is given as 55.
- The modal class is 45-60, so the lower limit L=45.
- The frequency preceding the modal class F0=5.
- The frequency succeeding the modal class F2=10.
- The class width H=60−45=15.
Step 2: Substitute the values into the mode formula
Substituting the known values into the formula:
55=45+F1−52F1−5−10×15
Step 3: Simplify the equation
First, simplify the equation:
55−45=F1−52F1−15×15
This simplifies to:
10=F1−52F1−15×15
Step 4: Cross-multiply to eliminate the fraction
Cross-multiplying gives:
10(2F1−15)=15(F1−5)
Expanding both sides:
20F1−150=15F1−75
Step 5: Rearranging the equation
Now, rearranging the equation to isolate F1:
20F1−15F1=150−75
This simplifies to:
5F1=75
Step 6: Solve for F1
Dividing both sides by 5 gives:
F1=15
Conclusion
The frequency of the modal class is F1=15.
---
- L = lower limit of the modal class
- F1 = frequency of the modal class (what we need to find)
- F0 = frequency of the class preceding the modal class
- F2 = frequency of the class succeeding the modal class
- H = class width (size)
From the question:
- The mode is given as 55.
- The modal class is 45-60, so the lower limit L=45.
- The frequency preceding the modal class F0=5.
- The frequency succeeding the modal class F2=10.
- The class width H=60−45=15.
Substituting the known values into the formula:
First, simplify the equation:
Cross-multiplying gives:
Now, rearranging the equation to isolate F1:
Dividing both sides by 5 gives:
The frequency of the modal class is F1=15.
Topper's Solved these Questions
- DIKSHA QUESTIONS BOOK - OSWAL PUBLICATIONCHAPTER - DIKSHA QUESTIONS EXERCISE - UNIT -VII: Statistics and Probability (Statistics) (Long Answer Type Questions)
4 Videos
- DIKSHA QUESTIONS BOOK - OSWAL PUBLICATIONCHAPTER - DIKSHA QUESTIONS EXERCISE - UNIT -VII: Statistics and Probability (Probability) (MULTIPLE CHOICE QUESTIONS)
6 Videos
- DIKSHA QUESTIONS BOOK - OSWAL PUBLICATIONCHAPTER - DIKSHA QUESTIONS EXERCISE - UNIT -VII: Statistics and Probability (Statistics) (VERY SHORT ANSWER TYPE QUESTIONS)
6 Videos
- COORDINATE GEOMETRYBOOK - OSWAL PUBLICATIONCHAPTER - COORDINATE GEOMETRYEXERCISE - SELF ASSESSMENT
20 Videos
- INTRODUCTION TO TRIGONOMETRY BOOK - OSWAL PUBLICATIONCHAPTER - INTRODUCTION TO TRIGONOMETRY EXERCISE - Self - Assessment
15 Videos
Similar Questions
Knowledge Check
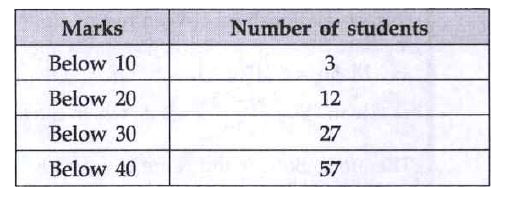
For the following distribution
the modal class isA40-50B20-30C30-40D50-60If the greater than cumulative frequency of a class is 60 and that of the next class is 40 , then find the frequency of that class .
A10B20C50D30In a frequency distribution, the mid values of a class is 10 and width of the class is 6. The lower limit of the class is
A6.5B7C8.5D12
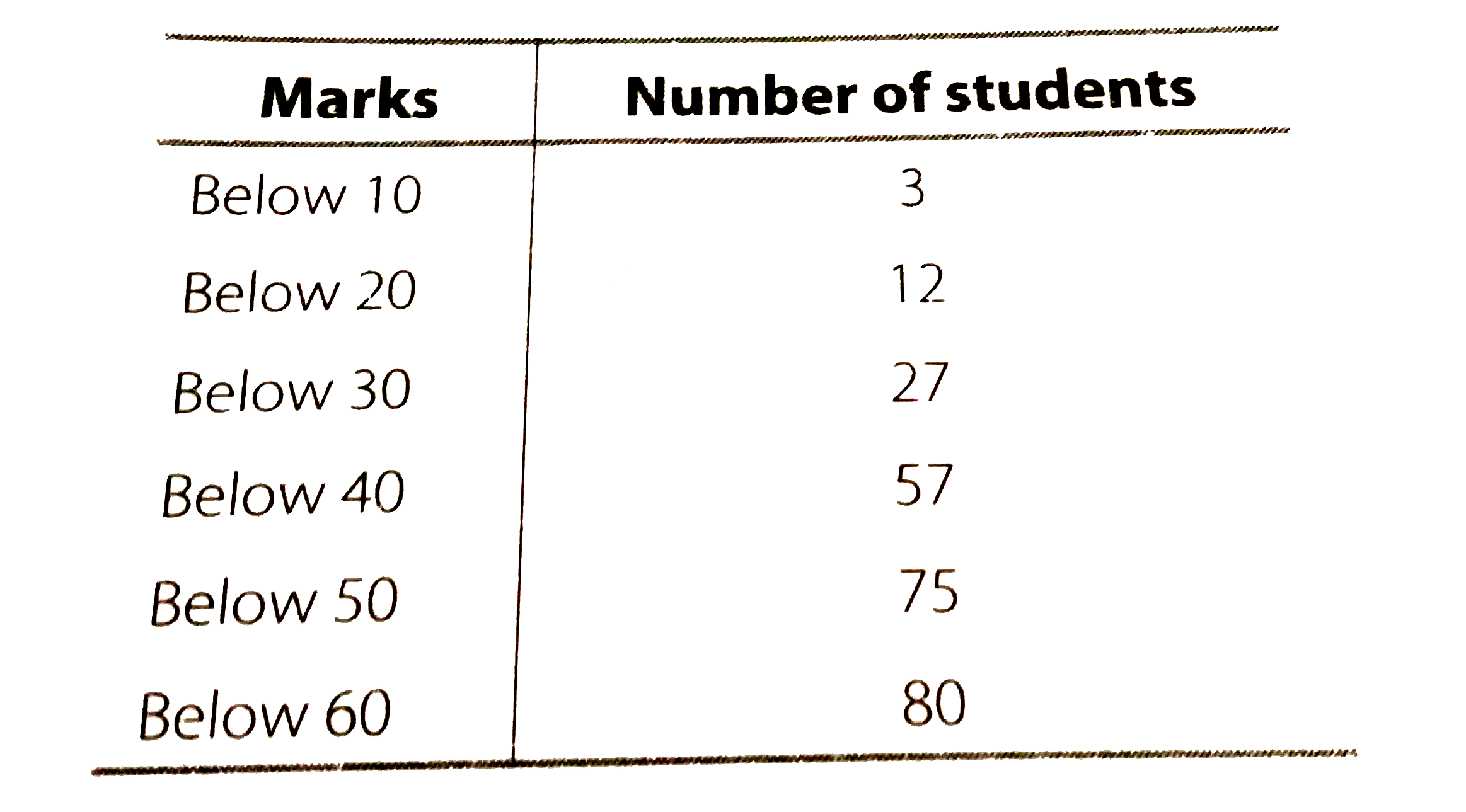
Find the sum of the lower limit of the median class and the upper limit of the modal class.
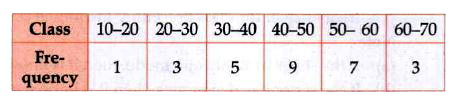
View SolutionWrite the modal class for the following frequency distribution.
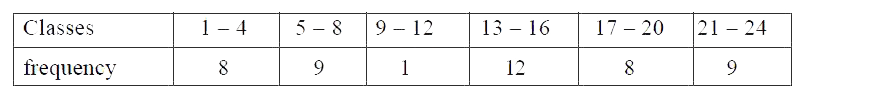
View SolutionConsider the data
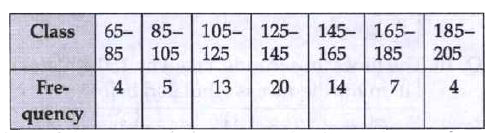
The difference of the upper limit of the median class and the lower limit of the modal class is
View SolutionIn a frequency distribution, the mid value of a class is 10 and the width of the class is 6. The lower limit of the class is
View SolutionIn a frequency distribution, the mid-value of a class is 10 and width of each class is 6. The upper limit of the class is
View Solution