A rubber ball is throuwn, after touching the ground it jumps again and again and forms a series : 100,90,80… then which is the term in the series when the ball will be at rest.
To solve the problem, we need to analyze the series given: 100, 90, 80, ..., and determine when the ball will be at rest.
1. Identify the Series:
The series starts at 100 and decreases by 10 each time. The terms are:
- First term (T1) = 100
- Second term (T2) = 90
- Third term (T3) = 80
- And so on...
2. Determine the Pattern:
The pattern shows that each term is obtained by subtracting 10 from the previous term. This can be expressed as:
- Tn = 100 - 10(n - 1), where n is the term number.
3. Find the Last Term Before the Ball Stops:
The ball will be at rest when it can no longer bounce, which is represented by the term reaching 0. We need to find n such that:
- Tn = 0.
4. Set Up the Equation:
Using the formula for Tn:
100−10(n−1)=0
5. Solve for n:
- Rearranging the equation:
100−10n+10=0
110−10n=0
10n=110
n=11010=11
6. Conclusion:
The term in the series when the ball will be at rest is the 11th term.
Final Answer:
The ball will be at rest at the 11th term of the series.
---
The series starts at 100 and decreases by 10 each time. The terms are:
- First term (T1) = 100
- Second term (T2) = 90
- Third term (T3) = 80
- And so on...
The pattern shows that each term is obtained by subtracting 10 from the previous term. This can be expressed as:
- Tn = 100 - 10(n - 1), where n is the term number.
The ball will be at rest when it can no longer bounce, which is represented by the term reaching 0. We need to find n such that:
- Tn = 0.
Using the formula for Tn:
100−10(n−1)=0
- Rearranging the equation:
100−10n+10=0
110−10n=0
10n=110
n=11010=11
The term in the series when the ball will be at rest is the 11th term.
The ball will be at rest at the 11th term of the series.
Topper's Solved these Questions
- NEW TYPOLOGIES INTRODUCED BY CBSE FOR BOARD 2021-22 EXAM BOOK - OSWAL PUBLICATIONCHAPTER - NEW TYPOLOGIES INTRODUCED BY CBSE FOR BOARD 2021-22 EXAM EXERCISE - UNIT-III COORDINATE GEOMETRY (CHAPTER 6 -LINES (IN TWO DIMENSIONS)
2 Videos
- NEW TYPOLOGIES INTRODUCED BY CBSE FOR BOARD 2021-22 EXAM BOOK - OSWAL PUBLICATIONCHAPTER - NEW TYPOLOGIES INTRODUCED BY CBSE FOR BOARD 2021-22 EXAM EXERCISE - UNIT-III COORDINATE GEOMETRY (CHAPTER 6 -LINES (IN TWO DIMENSIONS) (SELF ASSESSMENT)
4 Videos
- NEW TYPOLOGIES INTRODUCED BY CBSE FOR BOARD 2021-22 EXAM BOOK - OSWAL PUBLICATIONCHAPTER - NEW TYPOLOGIES INTRODUCED BY CBSE FOR BOARD 2021-22 EXAM EXERCISE - UNIT-II ALGEBRA (CHAPTER 5 -ARTHMETIC PROGRESSION)
1 Videos
- MAT STAGE I 2019-2020BOOK - OSWAL PUBLICATIONCHAPTER - MAT STAGE I 2019-2020EXERCISE - QUESTION PART
100 Videos
- NTSE 2019 -20BOOK - OSWAL PUBLICATIONCHAPTER - NTSE 2019 -20EXERCISE - Probability Stage -2
1 Videos
Similar Questions
A ball is thrown horizontally with speed 20m/s from a tower of height 80m.
(a) After how much time and at what horizontal distance from the foot of tower it strikes the ground.
(b) Find the magnitude and direction of the velocity with which it strikes the ground.
( c) Find the velocity vector of ball after 1s.
(d) Trajectory followed by the ball.
View Solution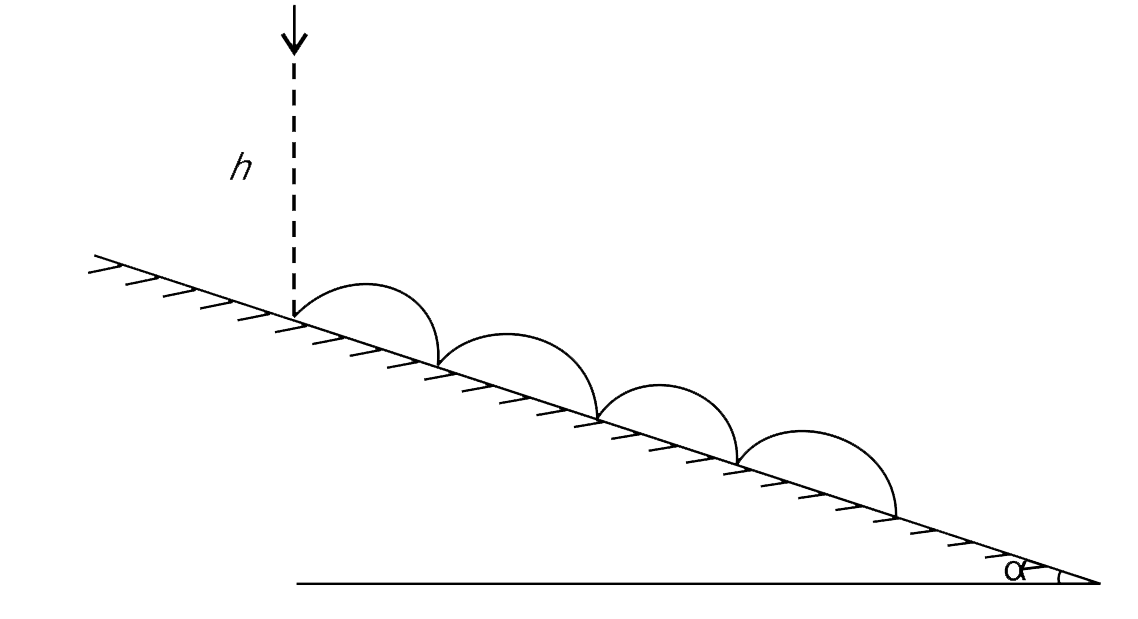
A ball is released in air above an incline plane inclined at an angle α to the horizontal. After falling vertically through a distance h it hits the incline and rebounds. The ball flies in air and then again makes an impact with the incline. This way the ball rebounds multiple times. Assume that collisions are elastic, i.e., the ball rebound without any loss in speed and in accordance to the law of reflection.
(a) Distance between the points on the incline where the ball makes first and second impact is l1 and distance between points where the ball makes second and third impact is l2. Which is large l1 or l2?
(b) Calculate the distance between the points on the incline where the ball makes second and fifth impact.
View Solution
Knowledge Check
A ball is thrown from ground vertically upward, reaches the roof of a house 100 meters high. At the moment this ball was thrown vertically upward, another ball is dropped from rest vertically downward from the roof of the house. At which height from the ground do the balls pass each other and after what time?
At=100√1960 sec , h = 25 mBt=100√1690 sec, h = 25 mCt=200√1690 , h = 75 mD100√1960 sec, h = 75 mA ball is dropped from a height 100 m on the ground. If the coefficient of restitution is 0.2, the height to which the ball will go up after it rebounds for the IInd time.
A16 mB1.6 cmC1.6 mD16 cmA ball starts falling freely from a height h from a point on the inclined plane forming an angle α with the horizontal as shown. After collision with the incline it rebounds elastically off the plane. Then it again strikes the incline at
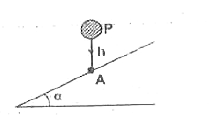 At=√8hg after it strikes the inclined at ABt=√2hgafter it strikes the inclined at A.Ca distance 4h sin α from A along the inclineDa distance 8h sin α from A along the incline
At=√8hg after it strikes the inclined at ABt=√2hgafter it strikes the inclined at A.Ca distance 4h sin α from A along the inclineDa distance 8h sin α from A along the incline
A small ball is released from rest and it accelerates due to gravity, after 1 sec suddenly gravity disappears and again appears after 5 sec Ball takes total 8 sec to reach the ground. The height above ground from where ball was released is?
View SolutionWhen a ball is dropped from a certain height h , it robounds from the ground , strikes again and rebounds again , a number of times before finally coming to rest . If the coefficient of restitution is e , find the height to which it robunds after pth collision ..
View SolutionA ball is thrown upwards with a speed of 20m/s from the ground. Another ball is dropped from rest from a building 80m high at the same time
(i) Find the difference in their height after they have fallen for 2s?
(ii) If the balls are along the same line of motion, when will they colide?
View SolutionA shot putter with a mass of 80kg pushes the iron ball of mass of 6kg from a standing position accelerating it uniformly form rest at an angle of 45∘ with the horizontal during a time interval of 0.1 seconds. The ball leaves his hand when it 2m high above the level ground and hits the ground 2 seconds later (g=10m/s2).
The horizontal disatnce between the point of release and the point where the ball hits the ground .
View SolutionA ball is projected vertically upwards with a velocity of 24.5 m/sec from the bottom of a tower. A boy who is standing at the top of the tower is unable to catch the ball when it is passing by him in the upwards direction. But the ball again reaches him after 3 seconds when it is falling and then he catches it. The velocity of the ball when the boy caught it and the height of the tower are
View Solution
