Fisher’s Price Number is given by
A
√Σp1q0Σp0q0+Σp1q1Σp0q1×100
B
√Σp0q0Σp1q0+Σp0q1Σp1q1×100
C
√Σp0q1Σp0q0+Σp1q1Σp1q0×100
D
√Σp1q0Σp1q1+Σp0q0Σp0q1×100
Text Solution
The correct Answer is:A
|
Similar Questions
Knowledge Check
Fisher’s Price Index Number is
A√P01(L)×P01(P)BP01(L)×P01(P)C√P01(L)×P01(P)×100D√P01(L)×P01(P)Walsh's Price Index Number is given by
A∑(q0√p0p1)∑(q1√p0p1)×100B∑(p0√q0q1)∑(p1√q0q1)×100C∑(q1√p0p1)∑(q0√p0p1)×100D∑(p1√q0q1)∑(p0√q0q1)×100Fisher's index number is considered ideal because:
Ait is based on variable weightsBit satisfies Time Reversal TestCit satisfies Factor Reversal TestDall of these
If Laspeyre’s and Paasche’s Price Index Numbers are 50 & 72 respectively, find Dorbish-Bowley’s and Fisher’s Price Index Numbers
View SolutionThe average of Laspeyre’s and Paasche’s Price Index Numbers is called……………..Price Index Number.
View SolutionFind the missing price if Laspeyre’s and Paasche’s Price Index Numbers are equal for following data.
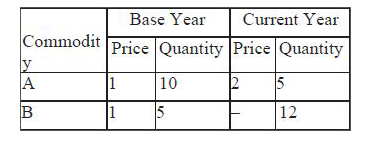
View SolutionConstruct price index number of the following data by using:
(i) Laspeyre's Method, (ii) Paasche's Method, and (iii) Fisher's Method.
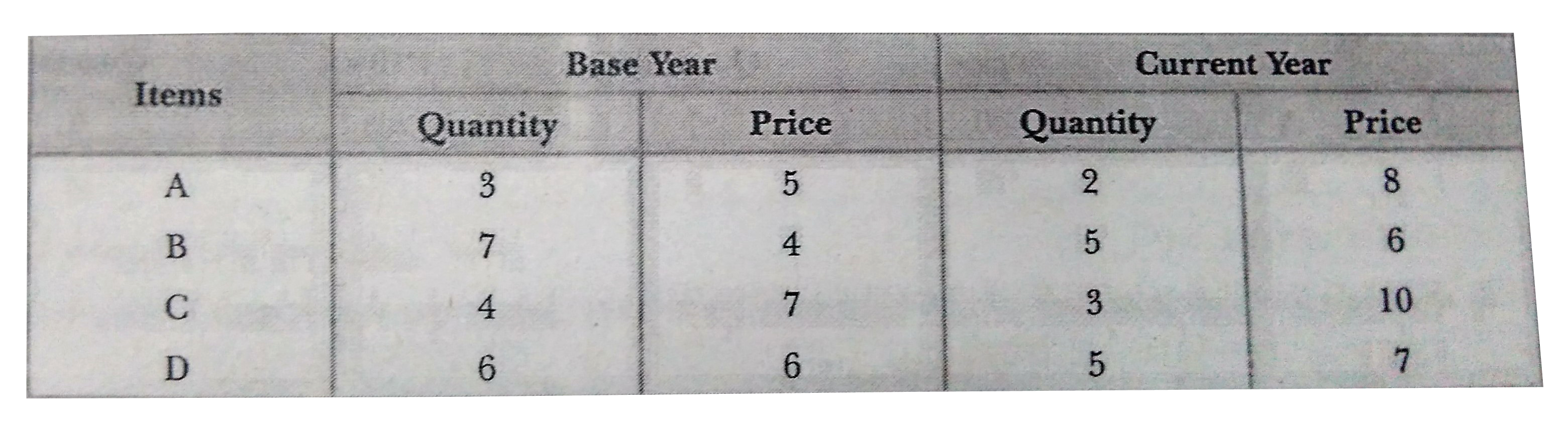
Note: Answers by all the methods are more or less equal. Nevertheless, Fisher's Index number is regarded as an ideal method.
View SolutionDorbish Bowley’s Price Index Number is
View Solution
