Question

What is the maximum number of parallel tangents a circle can have on a diameter?

Answer

2

Explanation

To determine the maximum number of parallel tangents a circle can have on a diameter, we can follow these steps:

1. Understanding Tangents: A tangent to a circle is a straight line that touches the circle at exactly one point. It does not cross the circle.

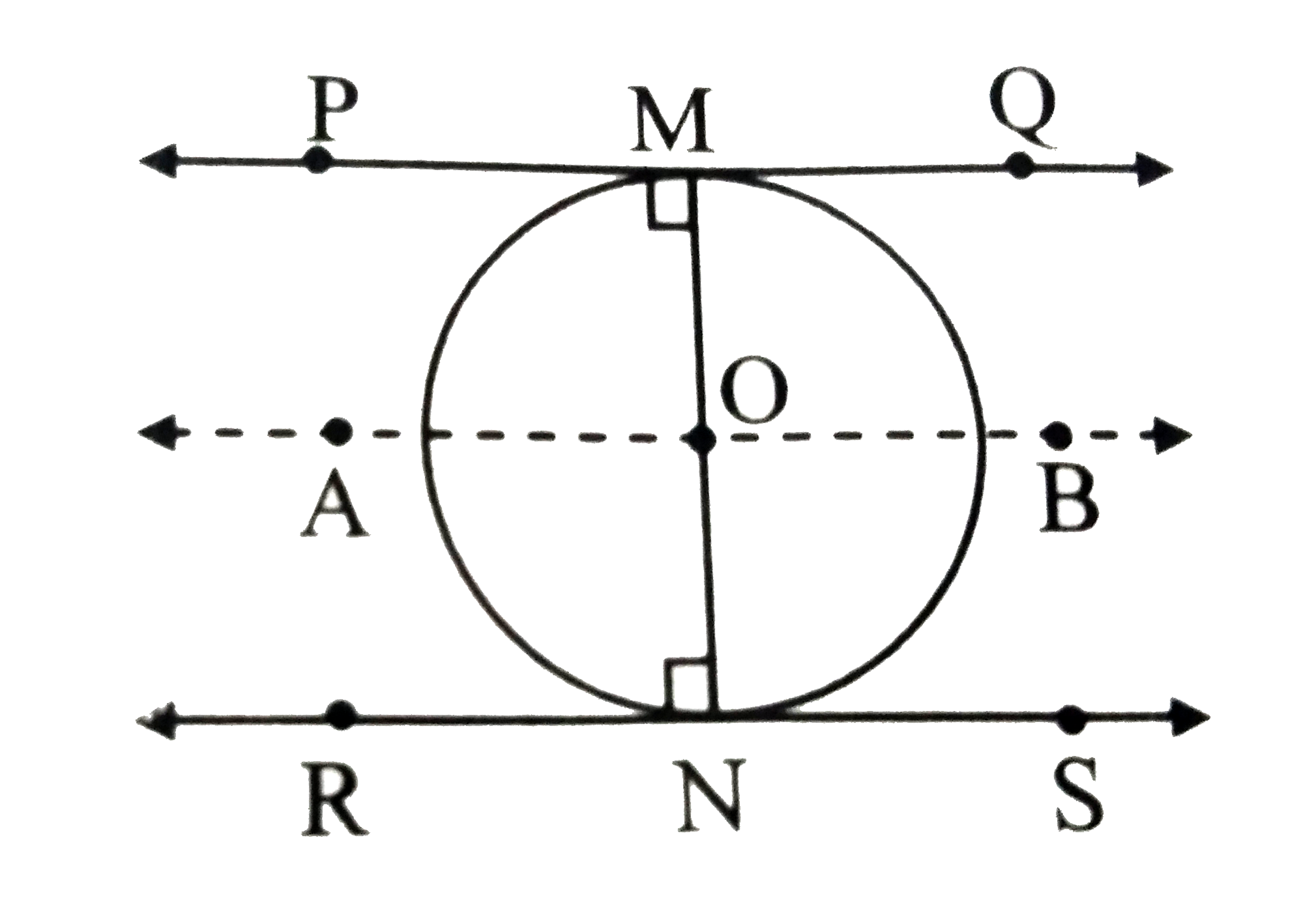
2. Identifying the Diameter: Consider a circle with a diameter. Let's denote the endpoints of the diameter as points A and B.

3. Drawing the Diameter: Draw a horizontal line segment AB, which represents the diameter of the circle.

4. Positioning the Circle: Place the circle such that its center is at the midpoint of the diameter AB. The circle will extend above and below this diameter.

5. Drawing Parallel Tangents: For any given point on the diameter, there can be a tangent line that touches the circle at that point.

6. Maximum Parallel Tangents: Since the diameter is a straight line, we can draw two parallel tangents to the circle at the endpoints of the diameter (points A and B). These tangents will be horizontal lines that run parallel to the diameter.

7. Conclusion: Therefore, the maximum number of parallel tangents that can be drawn on the diameter of a circle is 2.

Final Answer:
The maximum number of parallel tangents a circle can have on a diameter is 2.

---