An ideal gas 'X' has same density at 1 bar pressure as that of dinitrogen at 2 bar at 298 K. What is the molar mass of the gas 'X'?
The correct Answer is:56
d=PMRT
dN2=2×28R×298
Let the molar mass of gas be x
dgas=1×xR×298
dgas=dN2
1×xR×298=2×28R×298
∴x=56 g mol−1
Topper's Solved these Questions
Similar Questions
At 300 K, the density of a certain gaseous molecule at 2 bar is double to that of dinitrogen (N2) at 4 bar. The molar mass of gaseous molecule is:
View SolutionAt 300 K, the density of a certain gaseous molecule at 2 bar is double to that of dinitrogen (N2) at 4 bar. The molar mass of gaseous molecule is:
View Solution
Knowledge Check
At 0∘C , the density of a gaseous oxide at 2 bar is the same as that of dinitrogen at 5 bar. The molar mass of the oxide is
A60gmol−1B50gmol−1C70gmol−1D30gmol−1At 300 K, the density of a certain gaseous molecule at 2 bar is double to that of dinitrogen (N2) at 4 bar. The molar mass of gaseous molecule is:
A28gmol−1B56gml1−1C112gmol−1D224gmol−1At 300 K, the density of a certain gaseous molecule at 2 bar is double to that of dinitrogen (N2) at 4 bar. The molar mass of gaseous molecule is:
A56gmol−1B112gmol−1C224gmol−1D28gmol−1
The density of a gas at 27∘C and 1 bar pressure is 2.56 g L−1. Calculate the molar mass.
View SolutionTwo separate bulbs contain ideal gas A and B. The density of a gas A is twice that of a gas B. The molecular mass of A is half that of gas B. The two gases are at the same temperature. The ratio of the pressure of A to that gas B is
View SolutionThe density of a gas is found to be 1.56gdm−3 at 0.98 bar pressure and 65∘C. Calculate the molar mass of the gas.
View SolutionThe density of a gas is found to be 1.56 g dm−3at 0.98 bar pressure and 65∘C. Calculate the molar mass of the gas.
View Solution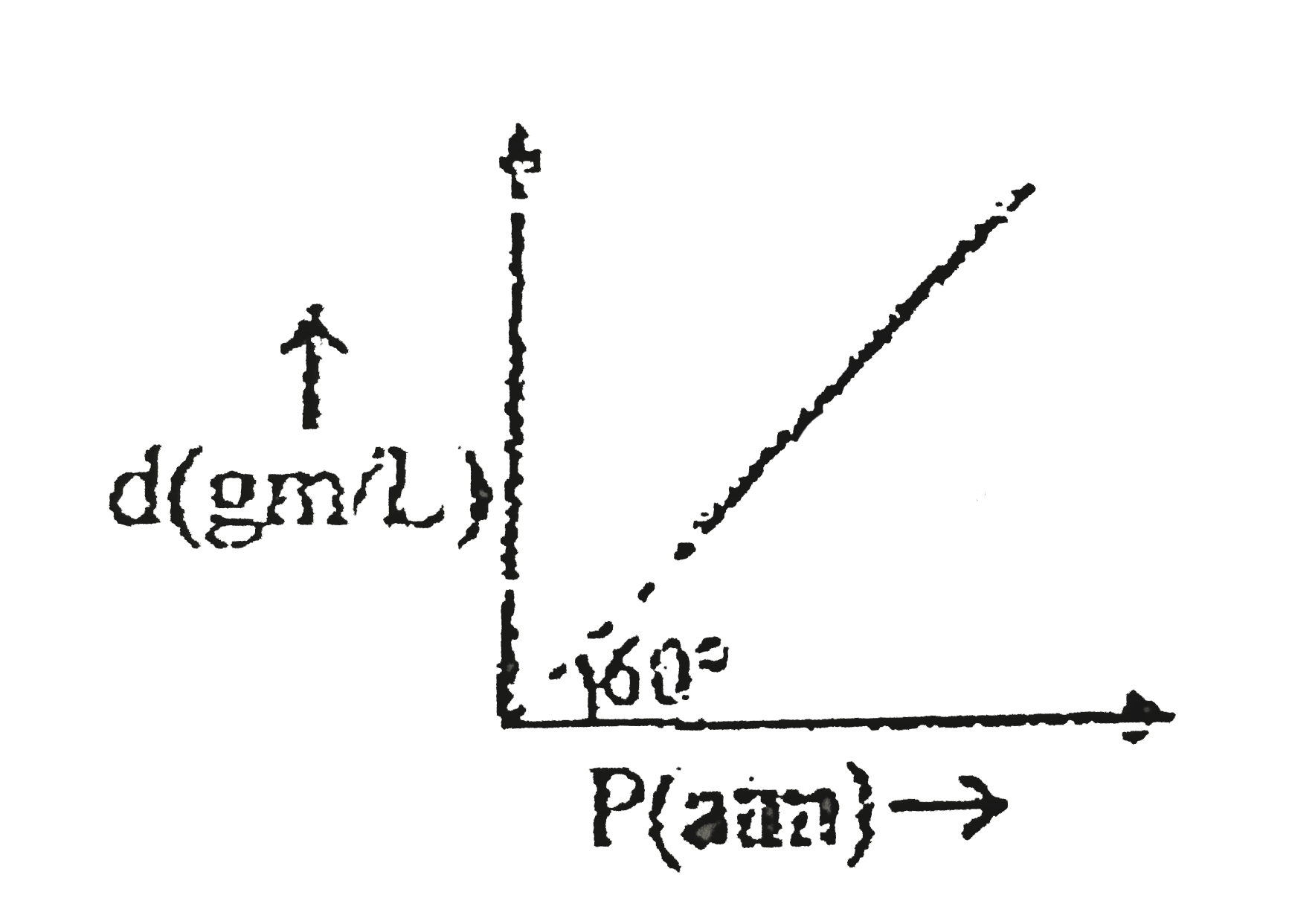
if density of an ideal gas when plotted against pressure exerted, shows the above variation at 273K. Find the molar mass (in gm/mol) the gas?
View Solution
