A small firm manufactures gold rings and chains. The total number of rings and chains manufactured per day is almost 24. it takes 1 hour to make a ring and 30 minutes to make a chain. The maximum number of hours available per day is 16. If the profit on a ring is ₹ 300 and that on a chain is ₹ 190, find the number of rings and chains that should be manufactured per day, so as to earn the maximum profit. Makes it is an L.P.P and solve it graphically.Solution in Punjabi
Step by step video & image solution for A small firm manufactures gold rings and chains. The total number of rings and chains manufactured per day is almost 24. it takes 1 hour to make a ring and 30 minutes to make a chain. The maximum number of hours available per day is 16. If the profit on a ring is ₹ 300 and that on a chain is ₹ 190, find the number of rings and chains that should be manufactured per day, so as to earn the maximum profit. Makes it is an L.P.P and solve it graphically. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- LINEAR PROGRAMMINGBOOK - MODERN PUBLICATIONCHAPTER - LINEAR PROGRAMMINGEXERCISE - EXERCISE
156 Videos
- INVERSE-TRIGONOMETRIC FUNCTIONSBOOK - MODERN PUBLICATIONCHAPTER - INVERSE-TRIGONOMETRIC FUNCTIONSEXERCISE - EXERCISE
111 Videos
- MATRICESBOOK - MODERN PUBLICATIONCHAPTER - MATRICESEXERCISE - EXERCISE
232 Videos
Similar Questions
Draw the graph of the following LPP: 3x+y≤17,x≥0,y≥0.
View SolutionFind the value of cos−1(cos(3π2))
View SolutionIf a matrix has 8 elements, what are the possibles orders it can have?
View SolutionA small firm manufactures gold rings and chains. The total number of rings and chains manufactured per day is atmost 24. It takes 1 hour to make a ring and 30 minutes to make a chain. The maximum number of hours available per day is 16. If the profit on a ring is Rs. 300 and that on a chain is Rs 190, find the number of rings and chains that should be manufactured per day, so as to earn the maximum profit. Make it as an L.P.P. and solve it graphically.
View SolutionRING-CHAIN ISOMERISM
View SolutionA block of mass m is attached to a chain as shown in the diagram. Find the tension in each of the three chains. The ring and chains are light.
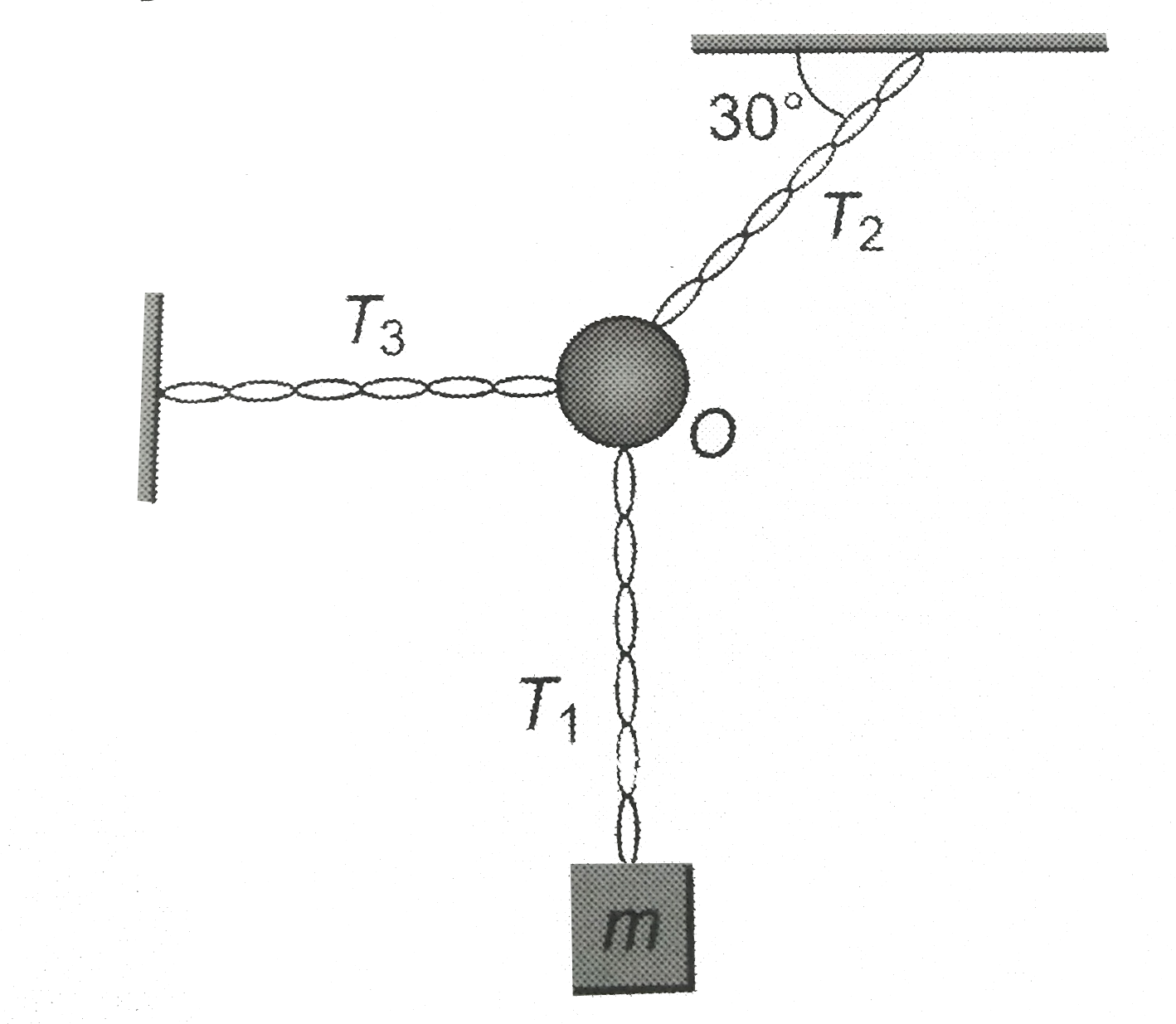
View SolutionIdentify the parent chain in the following compounds as ring or side chain
View SolutionA small firm manufactures gold rings and chains. The total number of rings and chains manufactured per day is atmost 24. It takes 1 hour to make a ring and 30 minutes to make a chain. The maximum number of hours available per day is 16. If the profit on a ring is Rs. 300 and that on a chain is Rs. 190, find the number of rings and chains that should be manufactured per day, so as to earn the maximum profit. Make it as an L.P.P. and solve it graphically.
View SolutionWrite the ring structure and open chain structure of fructose .
View Solutionएक छोटी फर्म, सोने की अंगूठियाँ व चेने बनाती है । एक दिन में अधिकतम 24 अंगूठियाँ व चेने बनायी जा सकती है । एक अँगूठी बनाने में 1 घंटा व 1 चेने बनाने में 30 मिनट लगते है । प्रतिदिन काम करने के लिए 16 घंटे ही उपलब्ध है । यदि एक अँगूठी पर रु 130 का तथा एक चेन पर रु 190 का लाभ होता है तो ज्ञात कीजिए कि अधिकतम लाभ कमाने के लिए इस फर्म को प्रतिदिन कितनी अँगूठियाँ व चेने बनानी चाहिए ?
View SolutionWhich property of carbon is responsible for forming straight chains, branched chains or rings?
View SolutionA shopkeeper manufactures gold rings and chains.The combined number of rings and chains manufactured per day is at most:(i) 16 (ii) 24 (iii)24
It takes (i) one hour (ii) half an hour (iii) one hour yo make a ring and (i) half an hour (ii) one hour (iii) half an hour for a chain.The maximum number of hours available is (i) 12(ii)16 (iii)16.
If the profit on a ring is :
(i)₹ 300 (ii) ₹ 100 (iii) ₹ 300
and on a chain is :
(i)₹ 200 (ii)₹ 300 (iii) ₹ 190.
How many of each should be manufactured daily, so as to maximize profti?Form an LPP and solve it graphically.
View SolutionWhat is ring - chain tautomerism ?
View SolutionA small firm manufactures gold rings and chains. The total number of rings and chains manufactured per day is almost 24. it takes 1 hour to make a ring and 30 minutes to make a chain. The maximum number of hours available per day is 16. If the profit on a ring is ₹ 300 and that on a chain is ₹ 190, find the number of rings and chains that should be manufactured per day, so as to earn the maximum profit. Makes it is an L.P.P and solve it graphically.
View SolutionA small firm manufacturers Golden chains and rings. The total number of chains and rings that it can manufacture in a day is at the most 24. The ring takes 30 minutes. The maximum time available per day is 16 hours. If the profit on the ring be Rs. 300 and one chain be Rs. 190. How many of rings and chains be produced to maximize the profit ?
View SolutionChain Position and Functional Group Isomerism || Ring chain Isomerism || Metamerism Isomerism
View Solution
