Find the perimeter of a quadrant of a circle of radius 'r'.
The correct Answer is:=12(π+4)
To find the perimeter of a quadrant of a circle with radius 'r', we can follow these steps:
Step 1: Understand the Quadrant
A quadrant of a circle is one-fourth of the circle. It consists of two straight sides (the radii) and one curved side (the arc).
Step 2: Identify the Components of the Perimeter
The perimeter (P) of the quadrant can be expressed as the sum of:
- The length of the two straight sides (the radii)
- The length of the arc
Step 3: Calculate the Length of the Straight Sides
Since both sides OA and OB are the radii of the circle:
- OA = r
- OB = r
Thus, the total length of the straight sides is:
OA+OB=r+r=2r
Step 4: Calculate the Length of the Arc
The circumference (C) of a full circle is given by the formula:
C=2πr
Since we only need one-fourth of the circumference for the quadrant, we calculate:
Arc length (AB)=14×C=14×2πr=πr2
Step 5: Combine the Lengths to Find the Perimeter
Now, we can combine the lengths of the straight sides and the arc to find the total perimeter of the quadrant:
P=OA+OB+Arc length
P=2r+πr2
Step 6: Factor Out 'r'
To simplify, we can factor 'r' out of the expression:
P=r(2+π2)
Final Expression for the Perimeter
Thus, the perimeter of the quadrant of a circle with radius 'r' is:
P=r(2+π2)
A quadrant of a circle is one-fourth of the circle. It consists of two straight sides (the radii) and one curved side (the arc).
The perimeter (P) of the quadrant can be expressed as the sum of:
- The length of the two straight sides (the radii)
- The length of the arc
Since both sides OA and OB are the radii of the circle:
- OA = r
- OB = r
OA+OB=r+r=2r
The circumference (C) of a full circle is given by the formula:
C=2πr
Since we only need one-fourth of the circumference for the quadrant, we calculate:
Arc length (AB)=14×C=14×2πr=πr2
Now, we can combine the lengths of the straight sides and the arc to find the total perimeter of the quadrant:
P=OA+OB+Arc length
P=2r+πr2
To simplify, we can factor 'r' out of the expression:
P=r(2+π2)
Thus, the perimeter of the quadrant of a circle with radius 'r' is:
P=r(2+π2)
Similar Questions
What is the perimeter of a quadrant of a circle of radius 'r' ?
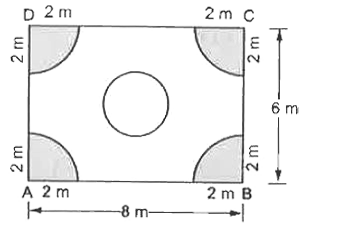
View SolutionIn each of the corners, there is a flower bed in the form of a quadrant of a circle of radius 2 m. Also, there is a flower bed in the form of a circle of radius 2 m in the middle of the plot. Find the area of the remaining plot.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
The perimeter of a quadrant of a circle of radius 'r' is :
Aπr2B2πrCr2[π+4]D2πr+r2 - Question 2 - Select One
The perimeter of a quadrant of a circle of radius 'r' is :
A≠r2B2πrCr2[π+4]D2πr+r2 - Question 3 - Select One
Find the perimeter of the quadrant of a circle of radius 14 cm.
A20 cmB500 cmC50 cmD5 cm
The perimeter of a certain sector of a circle of radius 6.5 cm is 31cm. Find the area of the sector.
View SolutionThe perimeter of a certain sector of a circle of radius 5.7 m is 27.2 m. find the area of the sector
View SolutionThe perimeter of a certain sector of a circle of radius 5.6 m is 27.2 m. Find the area of the sector
View SolutionFor r>0,f(r) is the ratio of perimeter to area of a circle of radius r. Then f(1) + f(2) is equal to
View SolutionFor r > 0, f(r) is the ratio of perimeter to area of a circle of radius r. Then f(1)+f(2) is equal to
View Solution

