Two dielectric blocks in series make a parallel plate capacitor. Blocks are of thickness d1 and d2 respectively.
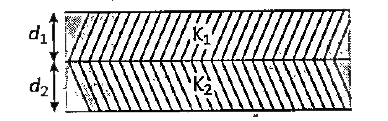
Effective dielectric constant is:
K1d1+K2d2K1+K2
K1d1+K2d2d1+d2
2K1K2K1+K2
K1K2(d1+d2)(K1d2+K2d1)
The correct Answer is:D
Capacitance of Block 1.
C1=K1ε0Ad1
Capacitance of Block 2
C2=K2ε0Ad2
Since the two capacitors are in series
1C=1C1+1C2
C=C1C2C1+C2
=K1K2ε0AK1d2+K2d1
Equivalent capacitance is given by
C=Kε0Ad1+d2
K=K1K2(d1+d2)K1d2+K2d1
Topper's Solved these Questions
- SAMPLE PAPER 04 (SOLVED)BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 04 (SOLVED)EXERCISE - SECTION-C
6 Videos
- SAMPLE PAPER 04 (SOLVED)BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 04 (SOLVED)EXERCISE - SECTION-C
6 Videos
- SAMPLE PAPER - 8BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER - 8EXERCISE - Section C
6 Videos
- SAMPLE PAPER 2BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 2EXERCISE - SECTION-C
6 Videos
Similar Questions
A parallel plate capacitor is made of two dielectric blocks in series. One of the blocks has thickness d1 and dielectric constant k1 and the other has thickness d2 and dielectric constant k2 as shown in Figure. This arrangement can be thought as a dielectric slab of thickness d(=d1+d2) and effective dielectric constant k. The k is :

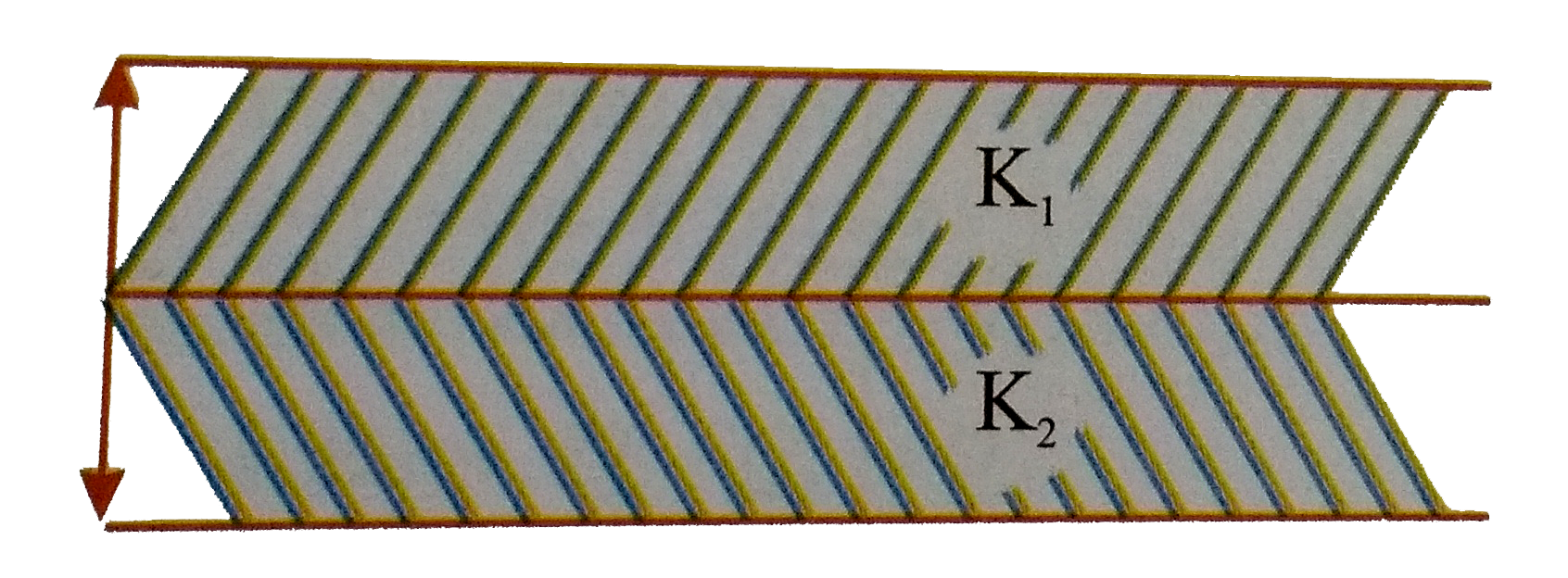
View SolutionA parallel plate capacitor is made of two dielectric blocks in series. One of the blocks has thickness d1 and dielectric constant K1 and the other has thickness d2 and dielectric constant K2 as shown in figure. This arrangement can be through as a dielectric slab of thickness d(=d1+d2) and effective dielectric constant K. The K is.
 .
.
View Solution
Knowledge Check
A parallel plate capacitor is made of two dielectric blocks in series. One of the blocks has thickness d1 and dielectric constant K1 and the other has thickness d2 and dielectric constant K2 as shown in figure. This arrangement can be through as a dielectric slab of thickness d(=d1+d2) and effective dielectric constant K. The K is.
 .AK1d1+K2d2d1+d2BK1d1+K2d2K1+K2CK1K2(d1+d2)K2d1+K1d2D2K1K2K1+K2
.AK1d1+K2d2d1+d2BK1d1+K2d2K1+K2CK1K2(d1+d2)K2d1+K1d2D2K1K2K1+K2A battery charges a parallel plate capacitor of thickness (d) so that an energy [U0] is stored in the system. A slab of dielectric constant (K) and thickness (d) is then introduced between the plates of the capacitor. The new energy of the system is given by
AKU0BK2U0CU0KDU0/K2The separation between the plates of a parallel plate capacitor is d and the area of each plate is A. iff a dielectric slab of thickness x and dielectric constant K is introduced between the plates, then the capacitance will be
Aε0Ad−x(1−1K)Bε0Ad+x(1−1K)Cε0Ad+x(1+1K)Dε0Ad−x(1+2K)
A parallel plate capacitor is made of two dielectric blocks in series. One of the blocks has thickness d1 and dielectric constant K1 and the other has thickness d2 and dielectric constant K2 as shown in figure. This arrangement can be through as a dielectric slab of thickness d(=d1+d2) and effective dielectric constant K. The K is.
 .
.
View SolutionA parallel plate capacitor is made of two dielectric blocks in series. One of the blocks has thickness d1 and dielectric constant K1 and the other has thickness d2 and dielectric constant K2 as shown in figure. This arrangement can be through as a dielectric slab of thickness d(=d1+d2) and effective dielectric constant K. The K is.
 .
.
View SolutionA parallel plate cepacitor is made of two dielectric blocks in series. One of the blocks has thickness d1 and dielectric constant k, and the ather has thickness d2, and dielectric constant k2 as showni in the figure, This arrangement can be thought as a dielestric slab of thickness d=(d1+d2) and effective dielectic eonstant k. Then k is (Given: {k}1=2,k2=3,d1=d2+4cm})
'(##CEN_KSR_PHY_JEE_CO19_E01_023_Q13##)'
View SolutionA battery charges a parallel plate capacitor of thickness (d) so that an energy [U0] is stored in the system. A slab of dielectric constant (K) and thickness (d) is then introduced between the plates of the capacitor. The new energy of the system is given by
View SolutionA slab of material of dielectric constant K has the same area as the plates of a parallel plate capacitor, but has a thickness 3d/4. Find the ratio of the capacitance with dielectric inside it to its capacitance without the dielectric.
View Solution
