In an examination, six subjects - S1, S2, S3, S4, S5, and S6 have equal maximum marks. The number
of marks scored by one particular candidate in subject S1 is 20% less than his marks in subject S6. The
ratio of marks scored by the same candidate in subject S2 to that in S3 is 4: 5 and that in S4 to S5 is 3: 4. The
number of marks scored by this candidate in subject S5 is 25% more than that of S6. He scored 65 marks in the
subject S3. He scored 436/9 % in the examination and the average of marks scored by him in all the subjects is
218/3
The number of marks obtained by the candidate in the subject S2 was how much less than in the
subject S4?
28
26
27
31
To solve the problem step by step, we will denote the marks scored by the candidate in each subject as follows:
- Let the marks in S6 be x.
- Then, the marks in S1 will be 0.8x (20% less than S6).
- The marks in S3 are given as 65.
- The ratio of marks in S2 to S3 is 4:5. Therefore, marks in S2 will be 45×65=52.
- The ratio of marks in S4 to S5 is 3:4. Let the marks in S5 be y. Then, the marks in S4 will be 34y.
- It is also given that marks in S5 are 25% more than in S6, which means y=1.25x.
Now, we can summarize the marks in each subject:
- S1 = 0.8x
- S2 = 52
- S3 = 65
- S4 = 34y=34(1.25x)=1516x
- S5 = 1.25x
- S6 = x
Next, we calculate the total marks scored by the candidate in all subjects:
Total Marks=S1+S2+S3+S4+S5+S6
Substituting the values we have:
Total Marks=0.8x+52+65+1516x+1.25x+x
Combining the terms:
Total Marks=(0.8x+1516x+1.25x+x)+117
To combine the x terms, we convert 0.8 and 1.25 to fractions:
0.8=45=6480,1.25=54=10080
Thus, we can write:
0.8x=6480x,1.25x=10080x,x=8080x
Now we can combine:
Total Marks=(6480+1516+10080+8080)x+117
Finding a common denominator (80):
1516=7580
So we have:
Total Marks=(64+75+100+8080)x+117=(31980)x+117
We know that the average marks scored by the candidate is 2183, and since there are 6 subjects:
Total Marks=6×2183=436
Setting the two expressions for total marks equal:
(31980)x+117=436
Subtracting 117 from both sides:
(31980)x=319
Multiplying both sides by 80319:
x=80
Now, substituting x back to find the marks in each subject:
- S1 = 0.8×80=64
- S2 = 52
- S3 = 65
- S4 = 1516×80=75
- S5 = 1.25×80=100
- S6 = 80
Now we need to find how much less the marks in S2 are than in S4:
Marks in S4−Marks in S2=75−52=23
Thus, the number of marks obtained by the candidate in subject S2 was 23 marks less than in subject S4.
- Then, the marks in S1 will be 0.8x (20% less than S6).
- The marks in S3 are given as 65.
- The ratio of marks in S2 to S3 is 4:5. Therefore, marks in S2 will be 45×65=52.
- The ratio of marks in S4 to S5 is 3:4. Let the marks in S5 be y. Then, the marks in S4 will be 34y.
- It is also given that marks in S5 are 25% more than in S6, which means y=1.25x.
- S1 = 0.8x
- S2 = 52
- S3 = 65
- S4 = 34y=34(1.25x)=1516x
- S5 = 1.25x
- S6 = x
Total Marks=S1+S2+S3+S4+S5+S6
Substituting the values we have:
Total Marks=0.8x+52+65+1516x+1.25x+x
Combining the terms:
Total Marks=(0.8x+1516x+1.25x+x)+117
To combine the x terms, we convert 0.8 and 1.25 to fractions:
0.8=45=6480,1.25=54=10080
Thus, we can write:
0.8x=6480x,1.25x=10080x,x=8080x
Now we can combine:
Total Marks=(6480+1516+10080+8080)x+117
Finding a common denominator (80):
1516=7580
So we have:
Total Marks=(64+75+100+8080)x+117=(31980)x+117
Total Marks=6×2183=436
Setting the two expressions for total marks equal:
(31980)x+117=436
Subtracting 117 from both sides:
(31980)x=319
Multiplying both sides by 80319:
x=80
- S1 = 0.8×80=64
- S2 = 52
- S3 = 65
- S4 = 1516×80=75
- S5 = 1.25×80=100
- S6 = 80
Marks in S4−Marks in S2=75−52=23
Similar Questions
In an examination, six subjects - S1, S2, S3, S4, S5, and S6 have equal maximum marks. The number of marks scored by one particular candidate in subject S1 is 20% less than his marks in subject S6. The ratio of marks scored by the same candidate in subject S2 to that in S3 is 4: 5 and that in S4 to S5 is 3: 4. The number of marks scored by this candidate in subject S5 is 25% more than that of S6. He scored 65 marks in the subject S3. He scored 436/9 % in the examination and the average of marks scored by him in all the subjects is 218/3
The number of marks obtained by the candidate in the subject S3 was how much percentage less than that of subject S5?View SolutionIn an examination, six subjects - S1, S2, S3, S4, S5, and S6 have equal maximum marks. The number of marks scored by one particular candidate in subject S1 is 20% less than his marks in subject S6. The ratio of marks scored by the same candidate in subject S2 to that in S3 is 4: 5 and that in S4 to S5 is 3: 4. The number of marks scored by this candidate in subject S5 is 25% more than that of S6. He scored 65 marks in the subject S3. He scored 436/9 % in the examination and the average of marks scored by him in all the subjects is 218/3
What was the average of marks obtained by the candidate in the subject S5 and S6 together?View Solution
Knowledge Check
- Question 1 - Select One
Highest marks are scored in the subject
AEnglishBHindiCMathsDNone of these - Question 2 - Select One
The marks obtained in 4 subjects in an examination are 200. If the average of marks obtained in 3 subjects is 48, then how many marks are obtained in fourth subject?
A50B144C152D56 - Question 3 - Select One
The Bar graph given below presents the marks (out of 100) obtained by 10 students in a subject.
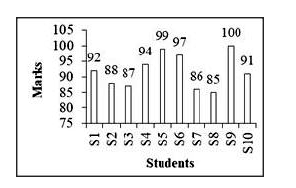
The average of marks obtained by S3 and 55 is what percent less than the marks obtained by S9?A8%B10%C7%D9%
In an examination, six subjects - S1, S2, S3, S4, S5, and S6 have equal maximum marks. The number of marks scored by one particular candidate in subject S1 is 20% less than his marks in subject S6. The ratio of marks scored by the same candidate in subject S2 to that in S3 is 4: 5 and that in S4 to S5 is 3: 4. The number of marks scored by this candidate in subject S5 is 25% more than that of S6. He scored 65 marks in the subject S3. He scored 436/9 % in the examination and the average of marks scored by him in all the subjects is 218/3
What percentage of marks the candidate had scored in the subject S5 over the maximum marks of that subject?View SolutionIn an examination, six subjects - S1, S2, S3, S4, S5, and S6 have equal maximum marks. The number of marks scored by one particular candidate in subject S1 is 20% less than his marks in subject S6. The ratio of marks scored by the same candidate in subject S2 to that in S3 is 4: 5 and that in S4 to S5 is 3: 4. The number of marks scored by this candidate in subject S5 is 25% more than that of S6. He scored 65 marks in the subject S3. He scored 436/9 % in the examination and the average of marks scored by him in all the subjects is 218/3
Total marks scored by S2 and S3 is multiple of which of the following pairs.View SolutionThe pie chart given shows the number of marks scored by a student in the given 5 subjects. The total marks scored by the student is 480.
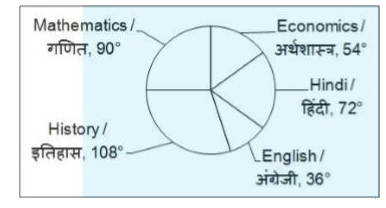
What is the average marks scored by the student in Hindi and Mathematics?View SolutionThe pie chart given shows the number of marks scored by a student in the given 5 subjects. The total marks scored by the student is 480.
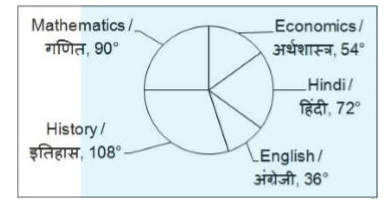
The marks scored by the student in Hindi is how much percent more than the marks scored in Economics?View SolutionThe pie chart given shows the number of marks scored by a student in the given 5 subjects. The total marks scored by the student is 480.
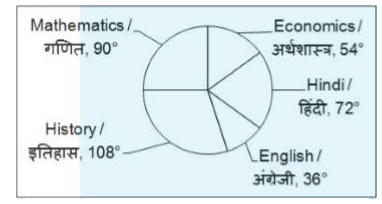
In which of the following 2 subjects the sum of marks scored by the student is equal to the marks scored in Mathematics?View Solution

