each of the following number series, the wrong number is given, find out that number. 15, 17, 19, 25, 32, 43
17
19
32
43
To find the wrong number in the series 15, 17, 19, 25, 32, 43, we can analyze the differences between consecutive terms and look for a pattern.
1. Identify the Series: The series given is:
15,17,19,25,32,43
2. Calculate the Differences: We will calculate the differences between consecutive terms:
- 17−15=2
- 19−17=2
- 25−19=6
- 32−25=7
- 43−32=11
So, the differences are:
2,2,6,7,11
3. Analyze the Differences: Let's look at the differences we calculated:
- The first two differences are 2 and 2.
- The next difference is 6, which seems larger than the previous differences.
- The subsequent differences are 7 and 11.
4. Look for a Pattern: Notice that the differences do not seem to follow a consistent pattern. If we assume that the differences should be increasing in a certain way, we can check if replacing 19 with 20 gives a better pattern:
- If we replace 19 with 20, the new series becomes:
15,17,20,25,32,43
5. Recalculate the Differences with the New Series:
- 17−15=2
- 20−17=3
- 25−20=5
- 32−25=7
- 43−32=11
The new differences are:
2,3,5,7,11
6. Identify the Correct Pattern: The new differences 2,3,5,7,11 are all prime numbers. This indicates a consistent pattern in the series.
7. Conclusion: Since replacing 19 with 20 maintains a consistent pattern of prime number differences, we conclude that the wrong number in the original series is:
Wrong Number: 19
15,17,19,25,32,43
- 17−15=2
- 19−17=2
- 25−19=6
- 32−25=7
- 43−32=11
2,2,6,7,11
- The first two differences are 2 and 2.
- The next difference is 6, which seems larger than the previous differences.
- The subsequent differences are 7 and 11.
- If we replace 19 with 20, the new series becomes:
15,17,20,25,32,43
- 17−15=2
- 20−17=3
- 25−20=5
- 32−25=7
- 43−32=11
2,3,5,7,11
Wrong Number: 19
Similar Questions
Knowledge Check
In each of the following number series, the wrong number is given, find out that number. 5541, 5506, 5425, 5304, 5135, 4910, 4621
A5541B5506C5425D4621In each of the following number series, a wrong number is given, find out the number.
2160, 360, 69.18.6, 3–A69B18C360D6In each of the following number series, a wrong number is given, find out the number.
7,8,18.57. 228. 1165,6996–A57B1165C228DNone of these
In each of the following number series, the wrong number is given, find out that number. 5, 23, 43, 66, 94, 131
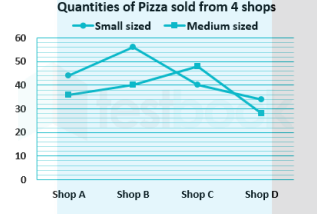
View SolutionDirection: Study the data carefully and answer the following questions. Following is the data given of selling 2 types of pizza from 4 shops A, B, C and D.
In each of the following number series, the wrong number is given, find out that number. 38, 39, 43, 52, 70, 93, 129
View SolutionIn each of the following number series, the wrong number is given, find out that number.
2,3,6,15,45,156.5,630
View SolutionIn each of the following number series, the wrong number is given, find out that number. 36, 20, 12, 8, 6, 5.5, 4.5
View SolutionIn the following number series a wrong number is given. Find out the wrong number
25,23,27,19,34,3,67
View Solution
