Two waves of same frequency and same amplitude from two monochromatic sources are allowed to superpose at a certain point. If in one case, the phase difference is 0∘ and in other case it is π2, then the ratio of the intensities in the two cases will be
1:1
2:1
4:1
None of these
The correct Answer is:B
Similar Questions
If two waves of same frequency and same amplitude superimpose and produce third wave of same amplitude, then waves differ in phase by –
View SolutionTwo waves of same frequency but of amplitude a and 2a respectively superimpose over each other. The intensity at a point where the phase difference is 3π2, will be proportional to :
View Solution
Knowledge Check
If two waves of same frequency and same amplitude superimpose and produce third wave of same amplitude, then waves differ in phase by –
Aπ/3B2π/3Cπ/2DπIf the two waves of the same frequency and same amplitude, on superposition produce a resultant disturbance of the same amplitude, then the phase difference between the two arriving wave will be
Aπ/2B2π/3CπD2πTwo waves of same frequency but of amplitude a and 2a respectively superimpose over each other. The intensity at a point where the phase difference is 3π2, will be proportional to :
A9a2B3a2Ca2D5a2
Two waves of the same frequency have amplitudes 1.60 and 2.20. They interfere at a point where their phase difference is 60.0∘. What is the resultant amplitude?
View SolutionTwo waves of the same frequency and same amplitude 'a' are reaching a point simultaneously. What should be the phase difference between the waves so that the amplitude of the resultant wave be :
(i) 2a (ii) √2a (iii) a (iv) zero.
View SolutionWhen two waves of the same frequency and same amplitude ‘a’ are superposed in phase, the resulting intensity at the point of superposition is proportional to
View SolutionIf maximum velocities of both the SHMs is same then find the ratio of amplitudes of the two cases
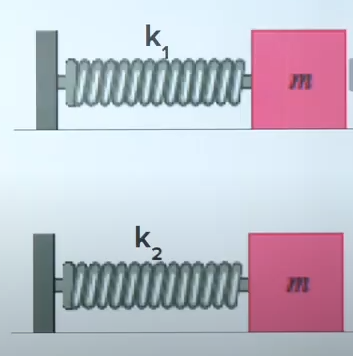
View SolutionTwo waves of same frequency have amplitudes 5 cm and 3 cm, These waves are made to superpose in the same direction. The ratio of maximum intensity to minimum intensity at various places will be
View Solution
