If [x] denotes the greatest integer less than or equal to x for any re

Let [x] denote the greatest integer less than or equal to x for any real number x. then $lim x \to \infty \frac{[n \sqrt{2}]}{n}$ is equal to
A0
B2
C $\sqrt{2}$
D1
Let [x] be the greatest integer less than or equal to x, for a real number x. Then the following sum

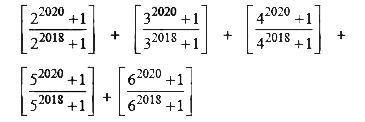
is-
A80
B85
C90
D95
Let [x] be the greatest integer less than or equal to x, for a real number x. Then the equation $[x^{2}] = x + 1$ has
Atwo solutions
Bone solution
Cno solution
Dmore than two solutions

If [x] denotes the greatest integer less than or equal to x for any real number x. Then, $lim x \to \infty \frac{[n \sqrt{2}]}{n}$ is